(a) Define electric flux. Write its SI units.
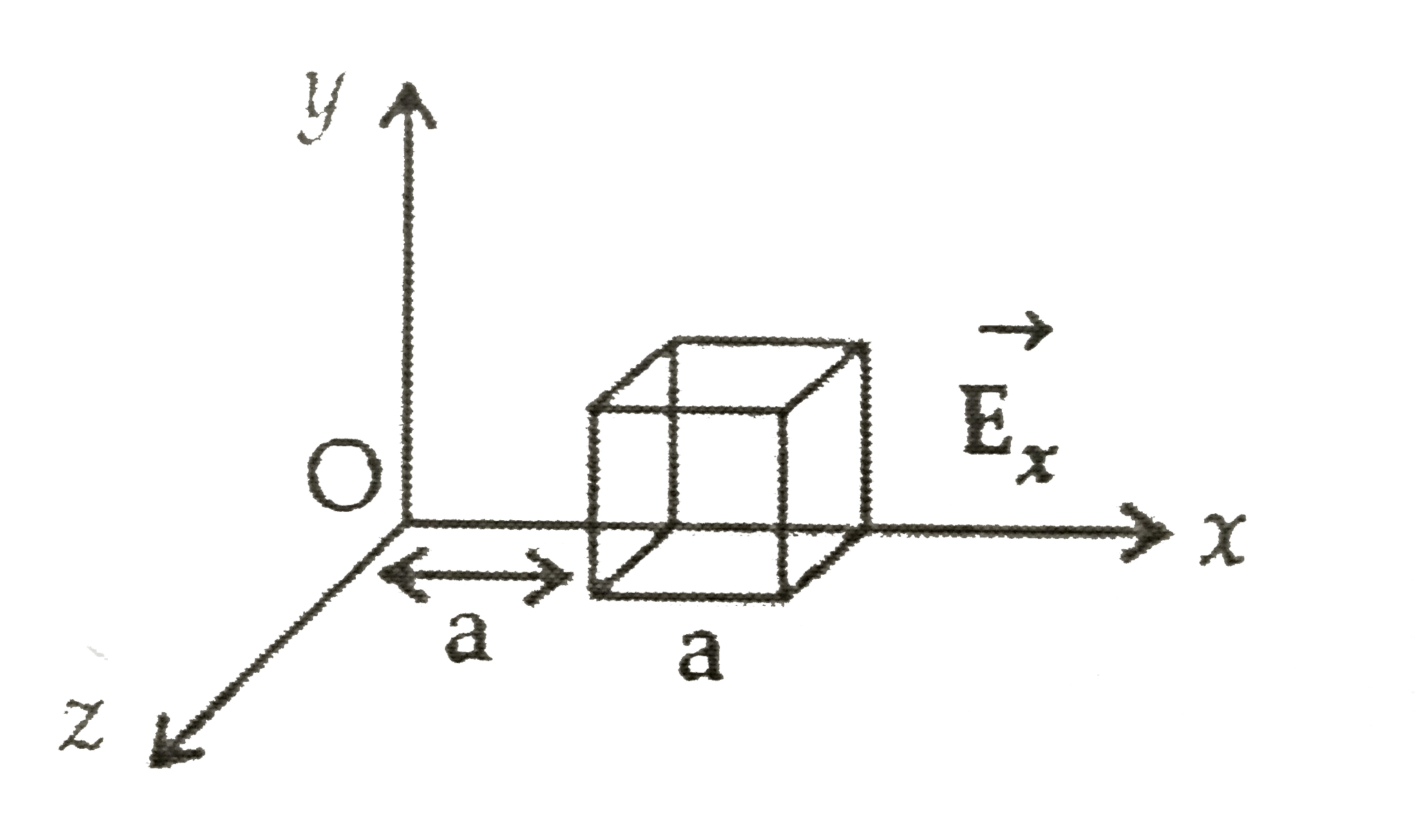
(b) The electric field components due to a charge inside the cube of side 0.1 m are as shown :
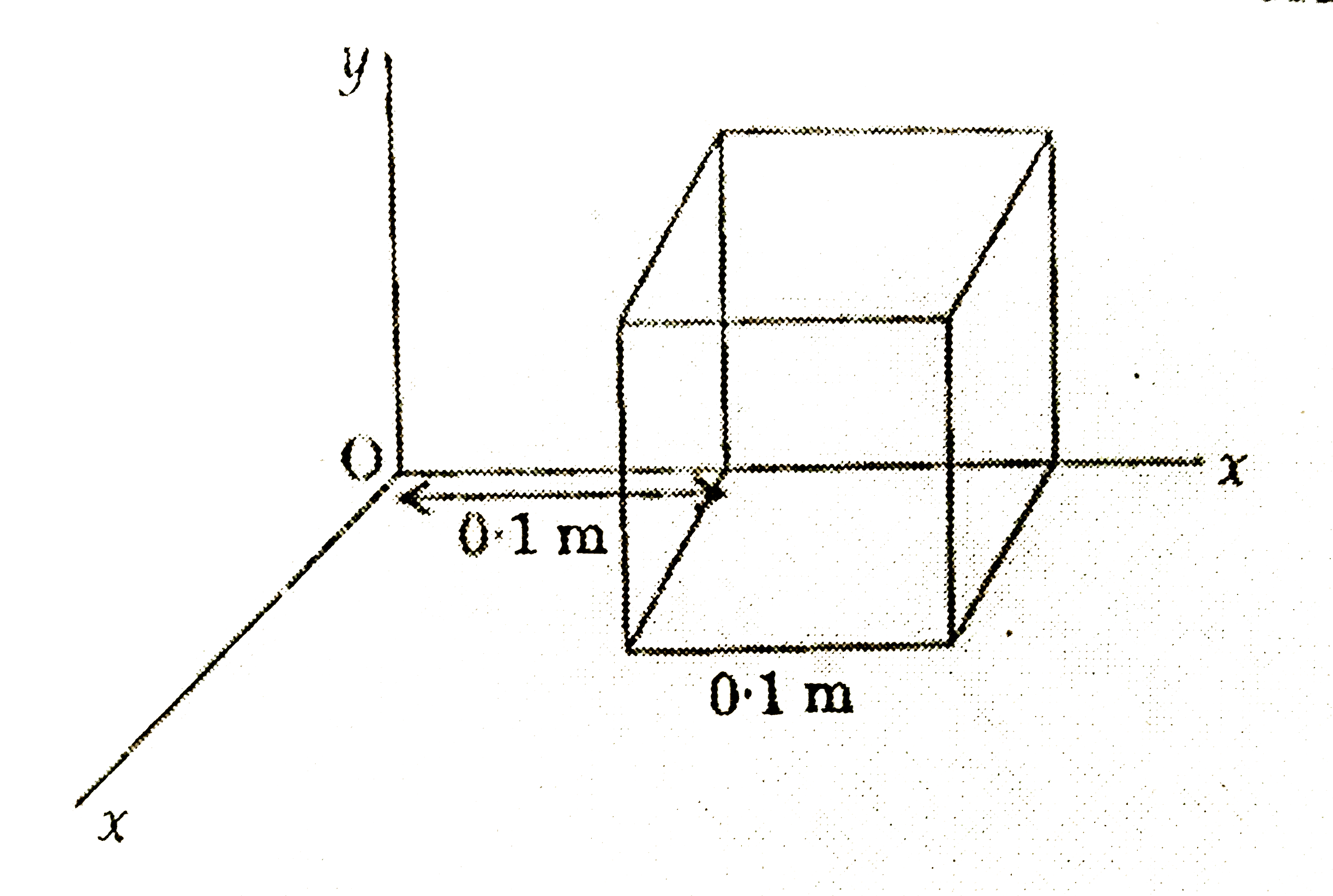
Ex=αx, where α=500N/C−m
Ey=0,Ez=0.
Calculate (i) the flux through the cube, and (ii) the charge inside the cube.
(a) Electric flux : Electric flux over an area in an electric field represents the total number of electric field lines crossing this area. It is denoted by ϕE.
Electric flux is a scalar quantity, its SI unit is Nm2c−1.
(b) Here, Ex=αx,Ey=0,Ez=0.
α=500N/C−m, side of cube a=0.1m
Since the electric field has only x component,
∴ϕE=→E.−−→ΔS=0 for each of four faces of cube ⊥ to y-axis and z-axis.
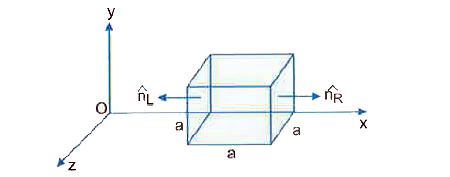
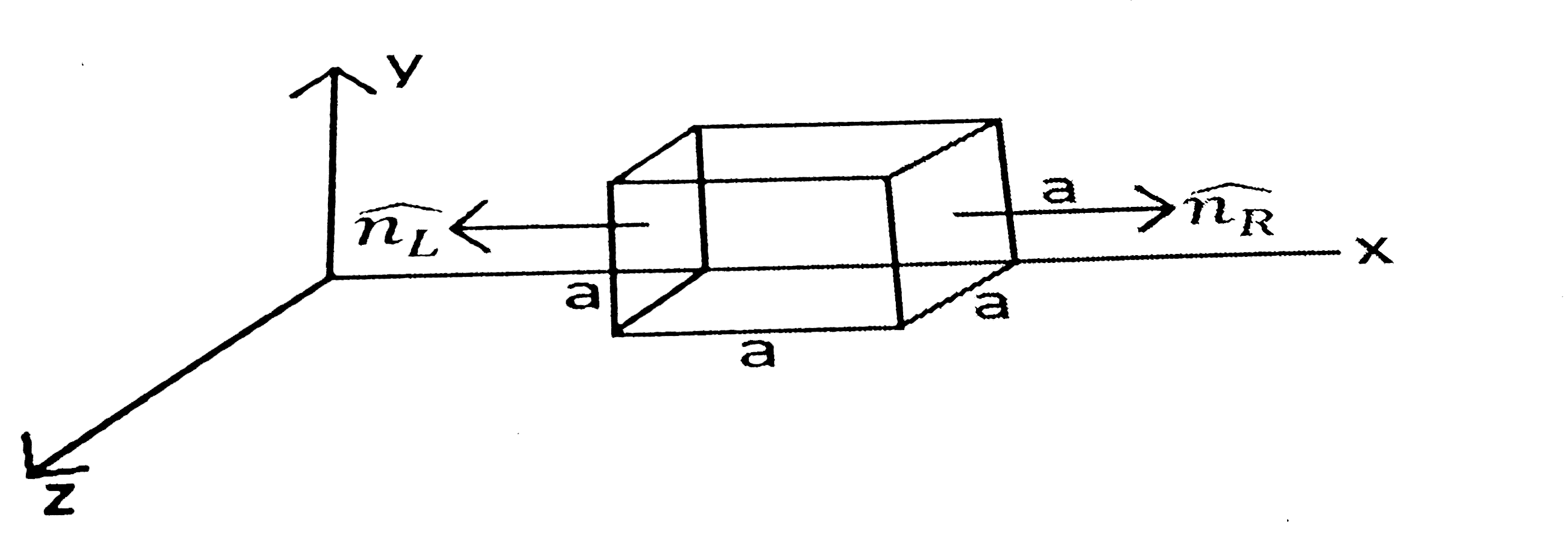
∴ Electric field at the left face, x=a is
∴EL=αa
ϕL=−→EL.−−→ΔS=αaa2cos180∘=−αa3 and electric field at the right face, [∵E=αx]
x=a+a=2a is
∴ER=α(2a)
ϕR=−→ER.−→Δs=α(2a)a2cos0∘=2αa3
∴ Net flux through the cube =ϕL+ϕL
=−αa3+2αa3=αa3=500×(0.1)3
=0.5Nm2C−1
(ii) By Gauss's law
q=∈0ϕ
=8.85×10−12×0.5=4.425×10−12C.
Topper's Solved these Questions
Similar Questions
Define electric flux. Write its SI units.
(b) The electric field components due to a charge inside the cube of side 0.1m area as shown in Ex=αx, where α=500N/Cm,EY=0andEZ=0.
Calculate (i) the flux through the cube , and
(ii) the charges inside the cube.
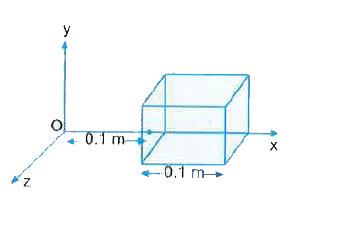
View SolutionThe electric field componets due to a charge inside the cube of side 0.1m are Ex=αx, where α=500(N/C)m−1,
Ey=0,Ez=0. Calculate the flux through the cube and the charge inside the cube.
View Solution
Knowledge Check
Due to a charge inside the cube, the electric field is: Ex = 600x, Ey = 0, Ez = 0. The charge inside the cube is nearly -
 A600μCB60μCC53μCD6μC
A600μCB60μCC53μCD6μCThe electric components in the figure are Ex=αx1/2,Ey=0,Ez=0 where α=800N/m2 if a=0.1m is the side of cube then the charge with in the cube is
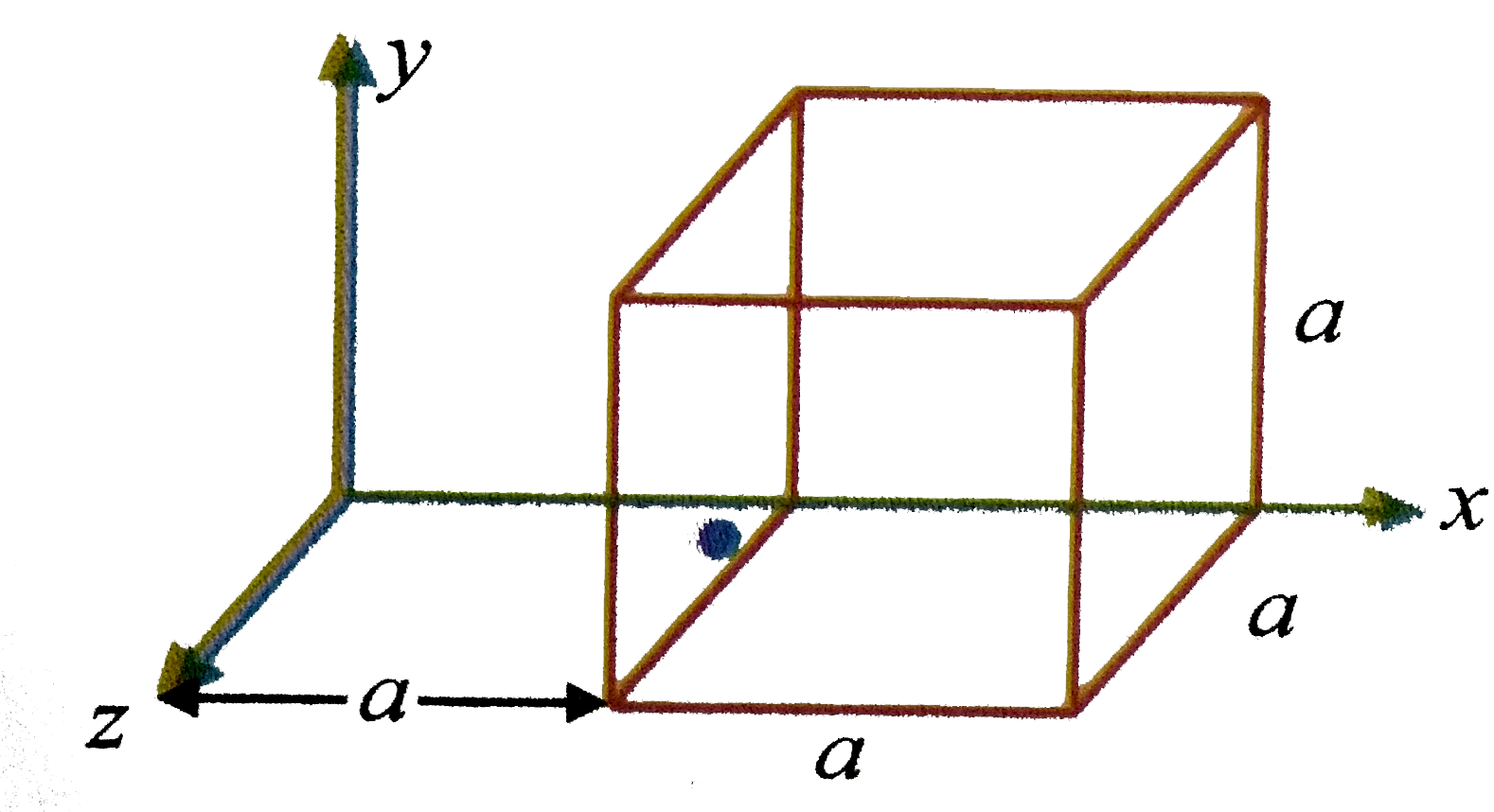 A9.27×10−12CB6×10−12CC2.5×10−12CDZero
A9.27×10−12CB6×10−12CC2.5×10−12CDZero
Define electric flux and write its SI unit . The electric field components in the figure shown are : Ex=αx,Ey=0,Ez=0 where α=100NCm.
Calculate the charge within the cube , assuming a = 0.1 m .
View Solution(a) Using Gauss's law, derive expression for intensity of electric field at any point near the infinitely long straight uniformly charged wire.
(b) The electric field components in the following figure are Ex=αx,Ey=0,Ez=0, in which α=400N/Cm. Calculate (i) the electric flux through the cube, and (ii) the charge within the cube assume that a=0.1m.
View SolutionUsing Gauss’s law, derive expression for intensity of electric field at any point near the infinitely long straight uniformly charged wire.
The electric field components in the following figure are Ex=αx,Ey=0,Ez=0, in which α=400N/C m. Calculate (i) the electric flux through the cube, and (ii) the charge within the cube assume that a = 0.1m.
View SolutionGiven the components of an electric field as Ex=αx,Ey=0 and Ez=0, where α is a dimensional constant. Calculate the flux through each face of the cube of side a, as shown in the figure.

View SolutionA non-uniform electric field E=(5xˆi+3ˆj)NC goes through a cube of side length 2.0m oriented as shown. Then,
View Solutioncalculate the electric field at point (5 m, 0) due to a point charge of 1 x 10(-6) C placed at (0,5m).
View Solution