Question

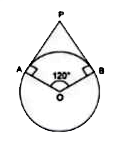
Two tangents drawn at the points A and B of a circle centred at O meet at P. If $\angle AOB={120}^{\circ }$ then $\angle APB:\angle APO$ is

Options

A. `2 : 5`
B. `3 : 2`
C. `4 : 1`
D. `2 : 1`

Answer

D

Explanation

To solve the problem, we need to find the ratio of angles $$\angle APB$$ to $$\angle APO$$ given that $$\angle AOB={120}^{\circ }$$.

1. Draw the Circle and Tangents:
- Draw a circle with center \( O \).
- Mark points \( A \) and \( B \) on the circumference of the circle.
- Draw tangents at points \( A \) and \( B \) that meet at point \( P \).

2. Identify the Angles:
- We know that \( \angle AOB = 120^\circ \).
- Since \( OA \) and \( OB \) are radii of the circle, the angles \( \angle OAP \) and \( \angle OBP \) are both \( 90^\circ \) because the radius is perpendicular to the tangent at the point of tangency.

3. Analyze Quadrilateral \( AOBP \):
- The sum of the angles in quadrilateral \( AOBP \) is \( 360^\circ \).
- Thus, we can write:
\( \angle AOB + \angle OAP + \angle OBP + \angle APB = 360^\circ \)
- Substituting the known values:
\( 120^\circ + 90^\circ + 90^\circ + \angle APB = 360^\circ \)
- Simplifying this gives:
\( 120^\circ + 180^\circ + \angle APB = 360^\circ \)
\( \angle APB = 360^\circ - 300^\circ = 60^\circ \)

4. Determine \( \angle APO \):
- Triangles \( AOP \) and \( BOP \) are congruent because:
- \( OA = OB \) (radii of the circle),
- \( AP = BP \) (tangents from a point outside the circle),
- \( \angle OAP = \angle OBP = 90^\circ \).
- Therefore, the angles \( \angle AOP \) and \( \angle BOP \) are equal.
- Let \( \angle AOP = \angle BOP = \theta \).
- The sum of angles in triangle \( AOB \) gives:
\( \theta + \theta + 120^\circ = 180^\circ \)
- Thus:
\( 2\theta = 60^\circ \implies \theta = 30^\circ \)
- Therefore, \( \angle APO = 30^\circ \).

5. Calculate the Ratio:
- Now we have:
\( \angle APB = 60^\circ \quad \text{and} \quad \angle APO = 30^\circ \)
- The ratio \( \angle APB : \angle APO \) is:
\( \frac{\angle APB}{\angle APO} = \frac{60^\circ}{30^\circ} = 2 \)
- Thus, the ratio \( \angle APB : \angle APO = 2 : 1 \).

Final Answer:
The ratio \( \angle APB : \angle APO \) is \( 2 : 1 \).