One end of a thin rod is attached to a pivot, about which it can rotate without friction. Air resistance is absent. The rod has a length of 0.80 m and is uniform. It is hanging vertically straight downward. The end of the rod nearest the floor is given a linear speed , so that the rod begins to rotate upward about the pivot. What must be the value of , such that the rod comes to a momentary halt in a straight-up orientation, exactly opposite to its initial orientation?
6.9 m/s
5.6 m/s
4.7 m/s
8.1 m/s
The correct Answer is:A
To solve the problem, we will use the principle of conservation of energy. The initial kinetic energy of the rod when it is given a linear speed v0 will be converted into gravitational potential energy when the rod reaches its highest point (momentarily halting in a vertical position).
1. Identify the Length of the Rod:
The length of the rod L is given as 0.80 m.
2. Determine the Change in Height:
When the rod is hanging vertically downward, its center of mass is at a height of L2 (0.40 m) from the pivot. When the rod is vertical upwards, the center of mass will be at a height of L (0.80 m) from the pivot. Thus, the change in height h is:
h=L−L2=L2=0.40m
3. Calculate the Potential Energy Gain:
The gravitational potential energy gained when the rod moves from the downward position to the upward position is given by:
ΔPE=mgh=mg⋅0.40
where g is the acceleration due to gravity (approximately 9.81m/s2).
4. Calculate the Initial Kinetic Energy:
The initial kinetic energy of the rod when it is given a linear speed v0 is:
KE=12Iω2
For a thin rod rotating about one end, the moment of inertia I is:
I=13mL2
The angular velocity ω can be related to the linear speed v0 by:
ω=v0L2=2v0L
Therefore, the kinetic energy can be rewritten as:
KE=12(13mL2)(2v0L)2=12⋅13mL2⋅4v20L2=23mv20
5. Set Up the Conservation of Energy Equation:
According to the conservation of energy:
Initial Kinetic Energy=Potential Energy Gain
23mv20=mg⋅0.40
6. Cancel Mass m from Both Sides:
23v20=g⋅0.40
7. Solve for v20:
v20=3⋅g⋅0.402
8. Substitute g=9.81m/s2:
v20=3⋅9.81⋅0.402=11.7722=5.886
9. Calculate v0:
v0=√5.886≈2.43m/s
Final Answer:
The required linear speed v0 is approximately 2.43m/s.
The length of the rod L is given as 0.80 m.
When the rod is hanging vertically downward, its center of mass is at a height of L2 (0.40 m) from the pivot. When the rod is vertical upwards, the center of mass will be at a height of L (0.80 m) from the pivot. Thus, the change in height h is:
h=L−L2=L2=0.40m
The gravitational potential energy gained when the rod moves from the downward position to the upward position is given by:
ΔPE=mgh=mg⋅0.40
where g is the acceleration due to gravity (approximately 9.81m/s2).
The initial kinetic energy of the rod when it is given a linear speed v0 is:
KE=12Iω2
For a thin rod rotating about one end, the moment of inertia I is:
I=13mL2
The angular velocity ω can be related to the linear speed v0 by:
ω=v0L2=2v0L
Therefore, the kinetic energy can be rewritten as:
KE=12(13mL2)(2v0L)2=12⋅13mL2⋅4v20L2=23mv20
According to the conservation of energy:
Initial Kinetic Energy=Potential Energy Gain
23mv20=mg⋅0.40
23v20=g⋅0.40
v20=3⋅g⋅0.402
v20=3⋅9.81⋅0.402=11.7722=5.886
v0=√5.886≈2.43m/s
The required linear speed v0 is approximately 2.43m/s.
Topper's Solved these Questions
- RIGID BODY DYNAMICS - IBook:RESNICK AND HALLIDAYChapter:RIGID BODY DYNAMICS - IExercise:PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
Explore 7 Videos
- RIGID BODY DYNAMICS - IBook:RESNICK AND HALLIDAYChapter:RIGID BODY DYNAMICS - IExercise:PRACTICE QUESTIONS (LINKED COMPREHENSION)
Explore 7 Videos
- RIGID BODY DYNAMICS - IBook:RESNICK AND HALLIDAYChapter:RIGID BODY DYNAMICS - IExercise:PROBLEMS
Explore 100 Videos
- RELATIVITYBook:RESNICK AND HALLIDAYChapter:RELATIVITYExercise:PRACTICE QUESTIONS (Integer Type)
Explore 5 Videos
- RIGID BODY DYNAMICS-IIBook:RESNICK AND HALLIDAYChapter:RIGID BODY DYNAMICS-IIExercise:PRACTICE QUESTIONS (Integer Type)
Explore 2 Videos
Similar Questions
A rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle θ from the vertical.

View SolutionA uniform. rod of length l can rotate without friction about an axis passing through its upper end (Fig). The rod is deflected by an angle a0 and let go. Find the speed of the lower end of the rod as a function of the angle α
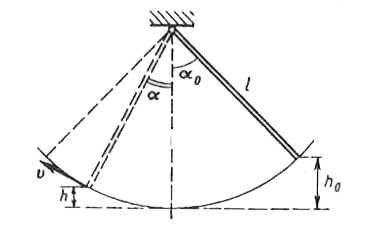
View Solution
Knowledge Check
A uniform rod AB of mass m and length 2a is falling freely without rotationb under gravity with AB horizontal. Suddenly the end A is fixed when the speed of the rod is v. The angular speed which the rod begains to rotate is
Av2aB4v3aCv3aD3v4aA uniform rod smoothly pivoted at one of its ends is released from rest. If it swings in vertical plane, the maximum speed of the end P of the rod is.
 .A2√3glB√3glC2√2glD√gl
.A2√3glB√3glC2√2glD√glA uniform rod of length l is from rest such that it rotates about a smooth pivot. The angular speed of the rod when it becomes vertical is.
 .A2√6g7lB√6g7lC2√3g7lD√3g7l
.A2√6g7lB√6g7lC2√3g7lD√3g7l
A rod AB of mass m and length L is rotating in horizontal plane about one of its ends which is pivoted. The constant angular speed with which the rod is rotating is ω. The force applied on the rod by the pivot is
View SolutionA uniform rod of mass m and length L is fixed to an axis, making an angle θ with it as shown in the figure. The rod is rotated about this axis so that the free end of the rod moves with a uniform speed ‘v’. Find the angular momentum of the rod about the axis. Is the angular momentum of the rod about point A constant?
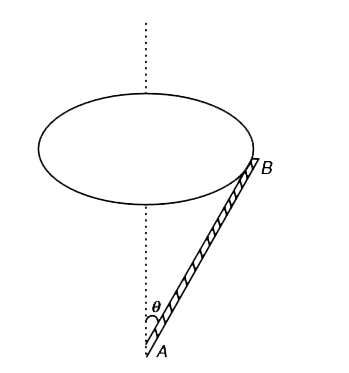
View SolutionA conical pendulum, a thin uniform rod of length l and mass m, rotates uniformly about a vertical axis with angular velocity ω(the upper end of the rod is hinged). Find the angle θ between the rod and the vertical.
View SolutionA uniform rod AB of mass m length 2a is allowed to fall under gravity with AB in horizontal. When the speed of the rod is v suddenly the end A is fixed. Find the angular velocity with which it begins to rotate.
View Solution
A uniform rod AB of mass m and length L rotates about a fixed vertical axis making a constant angle θ with it as shown in figure. The rod is rotated about this axis, so that point B the free end of the rod moves with a uniform speed V in the horizontal plane then the angular momentum of the rod about the axis is:
View Solution
