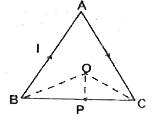
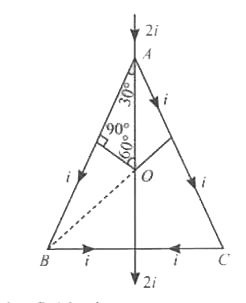
An equilateral triangle is made by uniform wires, AB, BC, CA. A current I enters at A and leaves from the mid point of BC. If the lengths of each side of the triangle is L, the magnetic field B at the centroid O of the triangle is
An equilateral triangle is made by uniform wires, AB, BC, CA. A current I enters at A and leaves from the mid point of BC. If the lengths of each side of the triangle is L, the magnetic field B at the centroid O of the triangle is

A

μ 0 4 π ( 4 I L )

B

μ 0 2 π ( 4 I L )

C

μ 0 4 π ( 2 I L )

D

zero

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:D

For AB, magnetic field B is along ⊙ ,
B = μ 0 i 4 π r ( sin 60 ∘ + sin 60 ∘ )
For AC, B is inside O x , B = μ 0 i 4 π r ( sin 60 ∘ + sin 60 ∘ ) .
For BD, is along ⊙ , B = μ 0 i 4 π r sin 60 ∘ ,
For DC, B is inside O x , B = μ 0 i 4 π r sin 60 ∘ ,
Since they are causing electric field in two exactly opposite directions eventually, they will cancel out each other effect. So, net magnetic field is zero, i.e., B net = 0
ocr_image

Updated on:21/07/2023
|
Class 12PHYSICSMOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A current of 2A is flowing in the sides of an equilateral triangle of side 9 cm. The magnetic field at the centroid of the triangle is

    A1.66×10−6T
    B1.22×10−4T
    C1.33×10−6T
    D1.44×10−4T

  • A conducting wire of length L is bent in the form of an equilateral triangle. If current I is made to pass through it, then calculate the magnetic field intensity at the centre of the triangle.

    A2√3μ0I4πL
    Bμ0I4πL
    C√3μ0I4πL
    Dzero

  • The current I ampere is flowing in an equilateral triangle of side a the magnetic field induction at the centroid will be

    A9μ0I2πa
    B5√2μ0I3πa
    C3μ0I2πa
    Dμ0I3√3πa