The sum m∑l=0(10i)(20m−i), where (pq) = 0 if p>q, is maximum when m is equal to
A
5
B
10
C
15
D
20
Video Solution
Text Solution
m∑i=0(10i)(20m−i) is the coefficient of xm in the expansion of (1+x)10(x+1)20,
⇒m∑i=0(10i)(20m−i) is the coefficient of xm in the expansion of (1+x)30
i.e. m∑i=0(10i)(20m−i)=30Cm=(30m) ...(i)
and we know that, (nr) is maximum, when
Hence, (30m) is maximum when m = 15.
|
Topper's Solved these Questions
- BINOMIAL THEOREMBOOK - IIT JEE PREVIOUS YEARCHAPTER - BINOMIAL THEOREMEXERCISE - Topic 2 Properties of Binomial Coefficent Objective Questions I (Only one correct option) (Numerical Value )
1 Video
- BINOMIAL THEOREMBOOK - IIT JEE PREVIOUS YEARCHAPTER - BINOMIAL THEOREMEXERCISE - Topic 2 Properties of Binomial Coefficent Objective Questions I (Only one correct option) (Fill in the Blanks)
1 Video
- BINOMIAL THEOREMBOOK - IIT JEE PREVIOUS YEARCHAPTER - BINOMIAL THEOREMEXERCISE - Topic 1 Binomial Expansion and General Term ( Objective Questions I) (Integer Answer Type Questions )
3 Videos
- AREABOOK - IIT JEE PREVIOUS YEARCHAPTER - AREAEXERCISE - AREA USING INTEGRATION
55 Videos
- CIRCLEBOOK - IIT JEE PREVIOUS YEARCHAPTER - CIRCLEEXERCISE - Topic 5 Integer Answer type Question
1 Video
Similar Questions
Knowledge Check
The sum m∑i=010Ci×20Cm−i(where pCq=0 if p<q)
is maximum, when m isA5B10C15D20The sum m∑i=0(10i)(20m−i) (where (pq)=0 if p<q) is maximum where m is
A5B10C15D20The sum m∑i=010Ci×20Cm−i(where pCq=0 if p<q)
is maximum, when m isA5B10C15D20
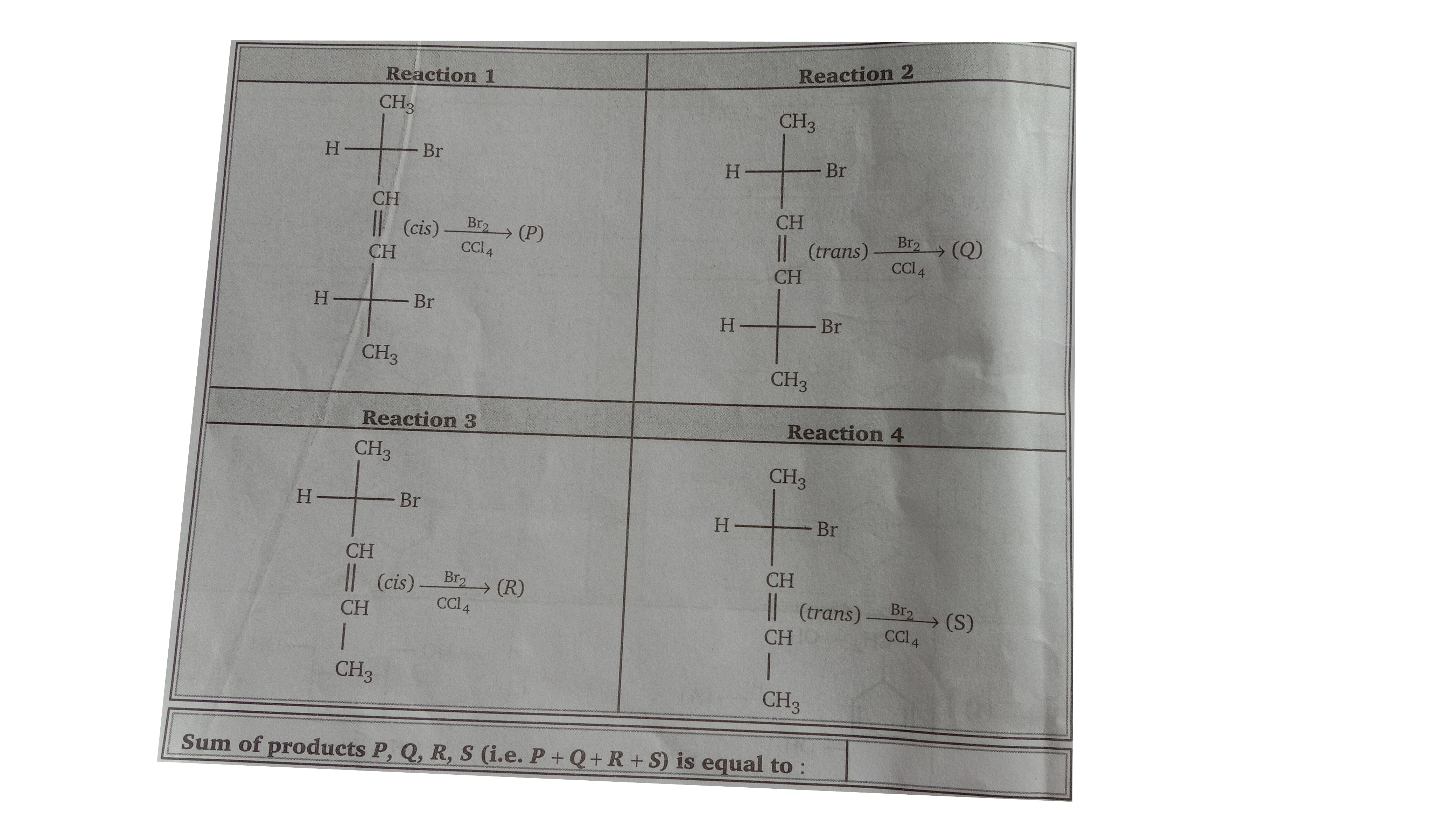
Sum of products P,Q,R,S (i.e. P+Q+R+S) is equal to:
View SolutionIf the sum of the series ∞∑m=1∞∑n−1m2n3m(n.3m+m.3n) can be expressed as pq where p and q are co-prime number, then (q−3p) is equal to
View SolutionThe sum m∑r=010Cr×20Cm−r is maximum ltbr> When m=
View SolutionFor nonnegative integers s and r , let
(sr)=s!r!(s−r)! 0 if r≤g if >s
For positive integers m and n , let
g(m,n)=m+n∑p=0f(m,n,p)(n+pp)
where for nay nonnegative integer p ,
f(m,n,p)=p∑i=0(n+ii),(n+ip),(p+np−i) Then which of the following statements is/are TRUE ?
View SolutionThe sum of (p+q)th and (p−q)th terms of an AP is equal to
View Solution