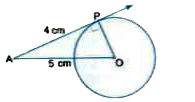
The length of tangent from a point A at a distance of 5 cm from the centre of the circle is 4 cm. What will be the radius of the circle ?
The correct Answer is:3 cm
To find the radius of the circle given the length of the tangent and the distance from the center, we can use the Pythagorean theorem. Here’s a step-by-step solution:
Step 1: Understand the problem
We have a point A outside the circle, which is 5 cm away from the center O of the circle. The length of the tangent AT from point A to the circle is 4 cm.
Step 2: Identify the right triangle
When a tangent is drawn from a point outside the circle to the point of tangency, it forms a right triangle with the radius and the line segment from the center to the point outside the circle. In this case, triangle OAT is a right triangle where:
- OA = 5 cm (distance from the center to point A)
- AT = 4 cm (length of the tangent)
- OT = r (radius of the circle, which we need to find)
Step 3: Apply the Pythagorean theorem
According to the Pythagorean theorem:
OA2=OT2+AT2
Substituting the known values:
52=r2+42
Step 4: Calculate the squares
Calculating the squares:
25=r2+16
Step 5: Solve for r²
Now, we can rearrange the equation to find r²:
r2=25−16
r2=9
Step 6: Find the radius
Taking the square root of both sides gives us:
r=√9=3 cm
Final Answer
The radius of the circle is 3 cm.
---
We have a point A outside the circle, which is 5 cm away from the center O of the circle. The length of the tangent AT from point A to the circle is 4 cm.
When a tangent is drawn from a point outside the circle to the point of tangency, it forms a right triangle with the radius and the line segment from the center to the point outside the circle. In this case, triangle OAT is a right triangle where:
- OA = 5 cm (distance from the center to point A)
- AT = 4 cm (length of the tangent)
- OT = r (radius of the circle, which we need to find)
According to the Pythagorean theorem:
OA2=OT2+AT2
Substituting the known values:
52=r2+42
Calculating the squares:
25=r2+16
Now, we can rearrange the equation to find r²:
r2=25−16
r2=9
Taking the square root of both sides gives us:
r=√9=3 cm
The radius of the circle is 3 cm.
Topper's Solved these Questions
- POST-MID TEAM TEST PAPERBOOK - VK GLOBAL PUBLICATIONCHAPTER - POST-MID TEAM TEST PAPEREXERCISE - SECTION-B
6 Videos
- POST-MID TEAM TEST PAPERBOOK - VK GLOBAL PUBLICATIONCHAPTER - POST-MID TEAM TEST PAPEREXERCISE - SECTION-C
10 Videos
- POLYNOMIALS BOOK - VK GLOBAL PUBLICATIONCHAPTER - POLYNOMIALS EXERCISE - SELF-ASSESSMENT TEST
11 Videos
- PRE-MID TERM TEST PAPERBOOK - VK GLOBAL PUBLICATIONCHAPTER - PRE-MID TERM TEST PAPEREXERCISE - SECTION-D
3 Videos
Similar Questions
Knowledge Check
The length of a tangent drawn from a point 10 cm away from the centre of the circle of radius 5 cm is
A5 cmB5√3 cmC2√3 cmD√15 cmA chord of length 60 cm is at a distance of 16 cm from the centre of a circle . What is the radius (in cm ) of the circle ?
A17B34C51D68A chord of length 120 cm is at a distance of 32 cm from the centre of a circle. What is the radius (in cm) of the circle?
A96B34C72D68
The length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
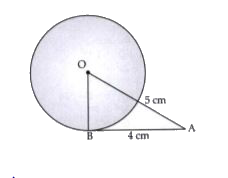
View SolutionThe length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
View SolutionFind the length of the tangent drawn from a point whose distance from the centre of a circle is 25 cm. Given that the radius of the circle is 7 cm.
View SolutionFind the length of the tangent segment from a point which is at a distance of 5 cm from the centre of the circle of radius 3cm.
View SolutionThe length of the tangent to a circle from a point P, which is 25 cm away from the centre, is 24 cm. What is the radius of the circle.
View Solution