A sodium vapour lamp is placed at the center of a large sphere that aborbs all the light reaching it. The rate at which the lamp emits energy is 100 W, assume that the emission is entirely at a wavelength of 590 nm. At what rate are photons absorbed by the sphere?
To find the rate at which photons are absorbed by the sphere, we can follow these steps:
1. Understand the Given Information:
- Power emitted by the sodium vapor lamp, P=100W
- Wavelength of emitted light, λ=590nm=590×10−9m
2. Use the Relationship Between Power, Energy, and Photons:
- The power emitted by the lamp can be expressed in terms of the energy of photons and the number of photons emitted per second:
P=Et=n⋅Ephotont
where n is the number of photons emitted per second and Ephoton is the energy of a single photon.
3. Calculate the Energy of a Single Photon:
- The energy of a single photon is given by:
Ephoton=hcλ
where:
- h (Planck's constant) =6.63×10−34J s
- c (speed of light) =3×108m/s
4. Substitute the Values to Find Ephoton:
Ephoton=(6.63×10−34J s)(3×108m/s)590×10−9m
Ephoton=1.989×10−25J m590×10−9m≈3.37×10−19J
5. Calculate the Rate of Photon Emission:
- Rearranging the power equation, we can find the number of photons emitted per second:
n=PEphoton
n=100W3.37×10−19J≈2.97×1020photons/s
6. Final Answer:
- The rate at which photons are absorbed by the sphere is approximately:
n≈2.97×1020photons/s
- Power emitted by the sodium vapor lamp, P=100W
- Wavelength of emitted light, λ=590nm=590×10−9m
- The power emitted by the lamp can be expressed in terms of the energy of photons and the number of photons emitted per second:
P=Et=n⋅Ephotont
where n is the number of photons emitted per second and Ephoton is the energy of a single photon.
- The energy of a single photon is given by:
Ephoton=hcλ
where:
- h (Planck's constant) =6.63×10−34J s
- c (speed of light) =3×108m/s
Ephoton=(6.63×10−34J s)(3×108m/s)590×10−9m
Ephoton=1.989×10−25J m590×10−9m≈3.37×10−19J
- Rearranging the power equation, we can find the number of photons emitted per second:
n=PEphoton
n=100W3.37×10−19J≈2.97×1020photons/s
- The rate at which photons are absorbed by the sphere is approximately:
n≈2.97×1020photons/s
Topper's Solved these Questions
- PHOTONS AND MATTER WAVESBOOK - RESNICK AND HALLIDAYCHAPTER - PHOTONS AND MATTER WAVESEXERCISE - Check points
3 Videos
- PHOTONS AND MATTER WAVESBOOK - RESNICK AND HALLIDAYCHAPTER - PHOTONS AND MATTER WAVESEXERCISE - PROBLEMS
50 Videos
- OSCILLATIONSBOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions
57 Videos
- RELATIVITYBOOK - RESNICK AND HALLIDAYCHAPTER - RELATIVITYEXERCISE - PRACTICE QUESTIONS (Integer Type)
5 Videos
Similar Questions
A 100W sodium lamp radiates energy uniformly in all directions. The lamp is located at the centre of a large sphere that absorbs all the sodium light which is incident on it. The wavelength of the sodium light is 589nm. (a) What is energy associated per photon with the sodium light? (b) At what rate are photons delivered to the sphere?
View SolutionA 100W sodium lamp radiates energyuniformly in all directions. The lamp is located at the centre of a large sphere that absorbs all the sodium light which is incident on it. The wavelength of the sodium light is 589 nm. (a) what is the energy per photon associated with the sodium light? (b) At what rate are the photons delivered to the sphere ?
View Solution
Knowledge Check
All bodies, no matter how hot or cold, continuously radiate photons. At a given temperature, the intensities of the electromagnetic waves emitted by an object vary from wavelength to wavelength throughout the visible, infrared, and other regions of the spectrum. Figure illustrates how the intensity per unit wavelength depends on wavelength for a perfect blackbody emitter. Although this figure can strictly be applied only to a black body, yet this will approximately discribe the behavior of many of the self radiating systems. For example, sun has an approximate temperature of 6000K. it is not a black body, it has an emissivity of nearly 0.6 But its peak almost occurs at a wave length that predicted by Wein's law. Suppose we have a bulb of power 100W. It emits only about 5W as visible light, rest is emitted as infrared radiated. Assume that the bulb filament has a surface area of 10mm^(2) (hc=1250eV−nm)

Assume that the light emitted by the bulb in the visible region is entirely of wavelength 500 nm. What is the number of photons emitted per second in the visible region?A1.25×1019B5×1019C2.5×1019D4×1019
(a) A 100 W sodium Lamp radiates energy uniformly in all directions. The Lamp is located at the centre of a large sphere , that absorbs all the sodium of a large sphere , that absorbs all the sodium light which is incident on it . The wavelength of the sodium light is 589 nm (i) What is the energy associated per photon with the sodium light ? (ii) At what rate are the photons delivered to the sphere ?
(b) Light of frequency 7.21×1014 Hz is incident on a metal surface . Electrons with a maximum speed of 6.0×105ms−1 are ejected from the surface What is the threshold frequency for photo emission of electron ?
Given , h=6.63×10−34Js,mc=9.1×10−310Kg
View SolutionWhat is the photon energy for yellow light from a highway sodium lamp at a wavelength of 589 nm?
View SolutionA 20 W lamp rated 10% emits light of wavelength y. Calculate the number of photons emitted by the lamp per second.
View SolutionA satellite in Earth orbit maintains a panel of solar cells of area 2.90m2 perpendicular to the direction of the Sun's light rays. The intensity of the light at the panel is 1.39kW/m2. (a) At what rate does solar energy arrive at the panel? (b) At what rate are solar photons absorbed by the panel? Assume that the solar radiation is mono- chromatic, with a wavelength of 550 nm, and that all the solar radiation striking the panel is absorbed. (c) How long would it take for a "mole of photons" to be absorbed by the panel?
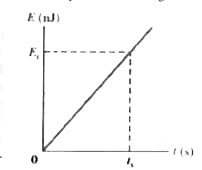
View SolutionA light detector has an absorbing area of 3.00×10−6m2 and absorbs 50% of the incident light, which is at wavelength 600 nm. The detector faces an isotropic source, 12.0 m from the source. The energy E emitted by the source versus time t is given in Fig. 37-19 Problem 28.
At what rate are photons absorbed by the detector?
View SolutionAt what rate does the Sun emit photons? For simplicity, assume that the Sun's entire emission at the rate of 3.9×1026 W is at the single wavelength of 550 nm.
View SolutionA sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.50 W cm−2. If the sphere completely absorbs the radiation falling on it, find the force exerted by the light beam on the sphere.
View Solution
