A strong magnet is placed under a horizontal conducting ring of radius r that carries current i as shown in Fig. If the magnetic field makes an angle θ with the vertical at the ring's location, what are the magnitude and direction of the resultant force on the ring?
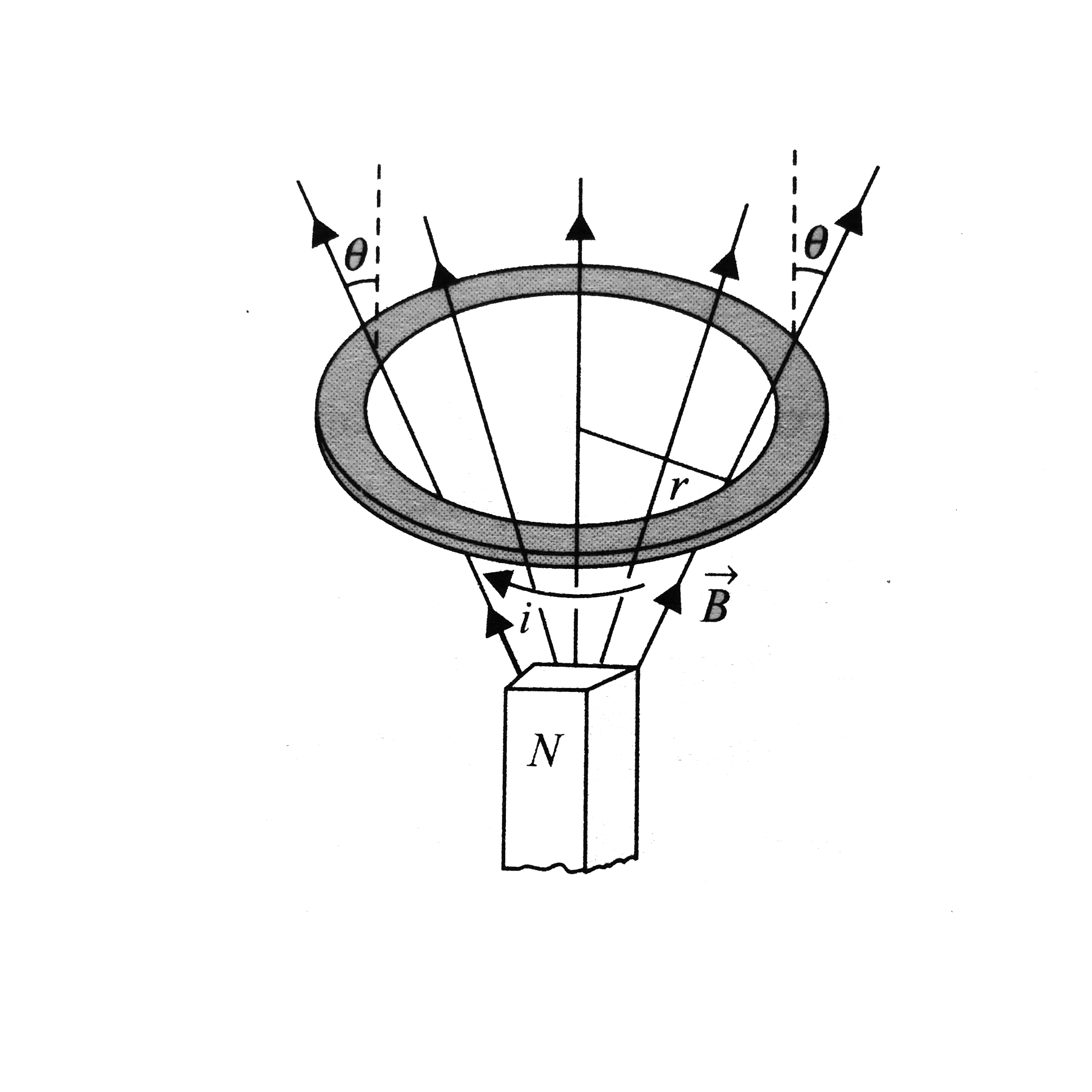
Here the wire is in a non-uniform magnetic field. The magnitude of the magnetic field at all the points of the ring is the same, but the direction is different. So, we can not say that the net force on wire is zero. We have two options: we can either divide the entire ring into small elements and integrate to find the force on the ring or
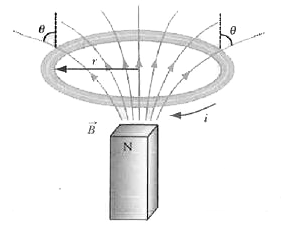
divide the magnetic field into two components and then integrate the force due to each component of the field. We choose the second option.
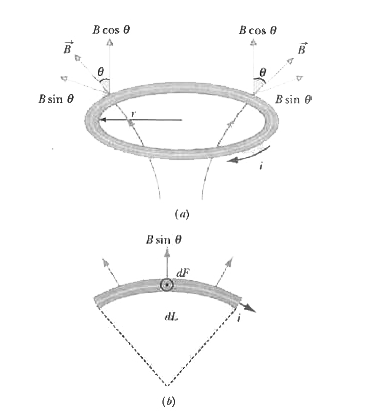
Calculation : The magnetic field at each point of the ring is having a vertical component of Bcosϕ. This field is uniform and hence the force due to this component of the magnetic field will be zero. The horizontal component of the magnetic field at each element of the rinf will be radially outward. This will produce a force on the element in vertically upward direction as can be seen from Fig.
The magnitude of this force is given by
dF=Bsinθ
The net force acting on the wire is given by
F=2πirBsinθ.
We can see that the vertical component of the magnetic field does not contribute to the net force on the wire : however, each element of the wire will be experiencing a force inward. This will tend to contract the wire. The current-carrying loop experiences a force in a nonuniform magnetic field.
Topper's Solved these Questions
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Check point
6 Videos
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Problems
49 Videos
- MAGNETIC FIELDS DUE TO CURRENTSBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FIELDS DUE TO CURRENTSEXERCISE - Practice Question (Integer Type)
4 Videos
- MOTION ALONG A STRAIGHT LINEBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )
3 Videos
Similar Questions
A conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.

View SolutionA conducting ring of radius r and resistance R is placed in region of uniform time varying magnetic field B which is perpendicular to the plane of the ring. It the magnetic field is changing at a rate α, then the current induced in the ring is
View Solution
Knowledge Check
A semicircle conducting ring of radius R is placed in the xy plane, as shown in Fig. A uniform magnetic field is set up along the x-axis. No emf, will be induced in the ring if
 Ait moves along the x-axisBit moves along the y-axisCit moves along the z-axisDit remains stationary
Ait moves along the x-axisBit moves along the y-axisCit moves along the z-axisDit remains stationaryA thin conducting ring of radius R is given a charge +Q, Fig. The electric field at the center O of the ring due to the charge on the part AKB of the ring is E. The electric field at the center due to the charge on part ACDB of the ring is
 A3E along KOBE along OKCE along KOD3E along OK
A3E along KOBE along OKCE along KOD3E along OKA battery is connected between two points AandB on the circumference of a uniform conducting ring of radius r and resistance R . One of the arcs AB of the ring subtends an angle θ at the centre . The value of the magnetic induction at the centre due to the current in the ring is
Aproportional to 2(180∘−θ)Binversely proportional to rCzero , only if θ=180∘Dzero for all values of θ
A battary is connected between two points A and B on the circumference of a uniform conducting ring of radius r and resistance R as shown in Fig. One of the arcs AB of the ring subtends angle θ at the centre. Show that that the magnetic field at the centre of the coil is zero and independent θ.
View SolutionA conducting ring of radius r is placed in a varying magnetic field perpendicular to the plane of the ring. If the rate at which the magnetic field varies is x, the electric field intensity at any point of the ring is
View SolutionA semicircular wire of radius r, carrying a current I, is placed in a magnetic field of magnitude B. The force acting on it
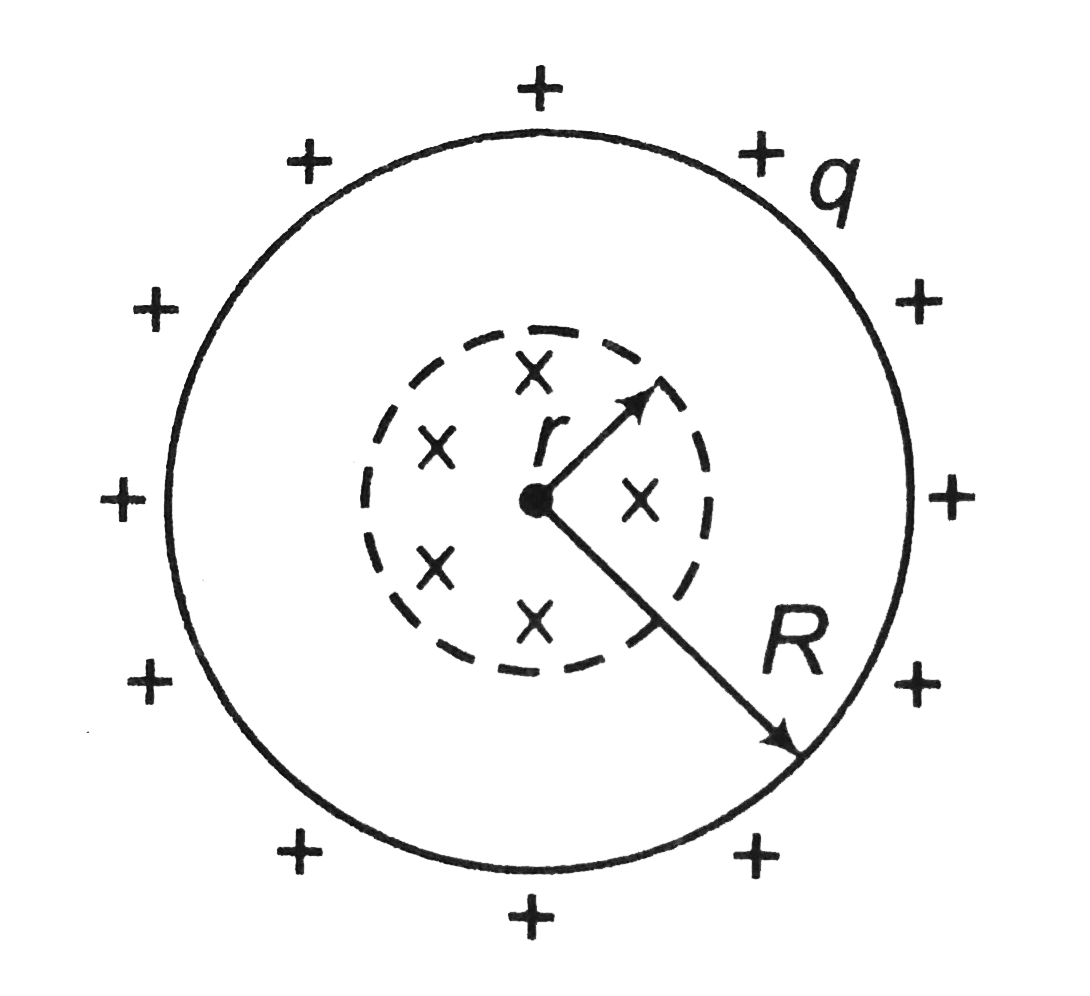
View SolutionThe figure shows a non conducting ring of radius R carrying a charge q. In a circular region of Radius r, a uniform magnetic field B perpendicular to the plane of the ring varies at a constant rate dBdt=β. The torque on the ring is
View SolutionA horizontal ring of radius r spins about its axis with an angular velocity ω in a uniform vertical magnetic field of magnitude B. The emf induced in the ring is
View Solution
