Between two junction stations A and B there are 12 intermediate stations. The total numbre of ways in which a train can be made to stop at 4 of these stations so that no two of these halting stations are consecutive, is
9C5
9C4
10C4
10C5
The correct Answer is:B
Let x1 be the number of stations before the first halting station, x2 between first and second, x3 between second and third, x4 between third and fourth and x5 on the right of the 4th stations. Then, x1≥0,x2,x3,x4≥1 such that
x1+x2+x3+x4+x5=8 ......(1)
The total number of ways is the number of solutions of the above equation.
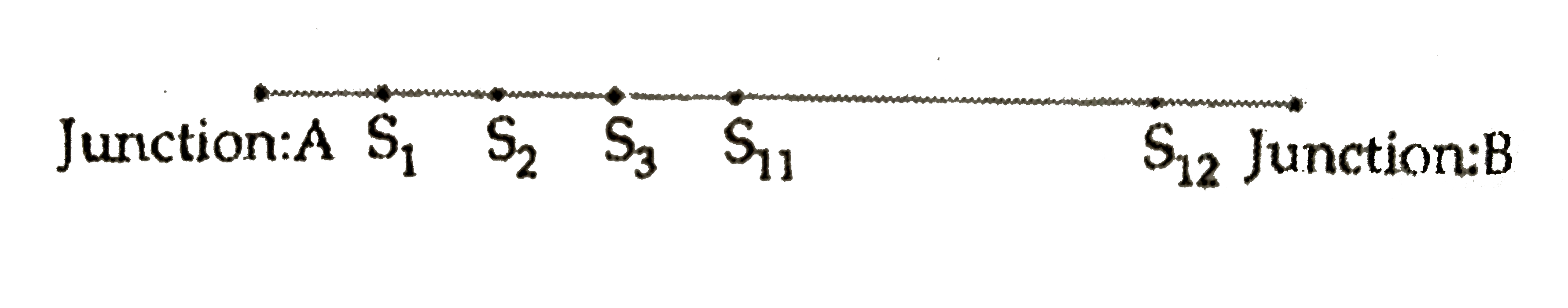
Let y2=x2−1,y3=x3−1,y4=x4−1. Then, (i) reduces to x1+y2+y3+y4+x5=5,where y2,y3,y4≥0.
The number of solutions of this equoation is 5+5−1C5−1=9C4.
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMACHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Section I - Solved Mcqs
111 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMACHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Section II - Assertion Reason Type
9 Videos
- PARABOLABOOK - OBJECTIVE RD SHARMACHAPTER - PARABOLAEXERCISE - Chapter Test
30 Videos
- PROBABILITY BOOK - OBJECTIVE RD SHARMACHAPTER - PROBABILITY EXERCISE - Chapter Test
45 Videos
Similar Questions
Between two junction stations A and B, there are 12 intermediate stations. Find the number of ways in which a train can be made to halt at 4 of these stations so that no two of these are consecutive
View SolutionThere are 10 stations between A and B. A to stop at three of these 10 station probability that no two of these stations consecutive is
View Solution
Knowledge Check
Between two junction stations A and B there are 12 intermediate stations. The number of ways in which a train can be made to stop at 4 of these stations so that no two of these halting stations are consecutive, is
A8C4B9C4C12C4−4Dnone of theseBetween two junction stations A and B, there are 12 intermediate stations. The number of ways in which a train can be made to stop at 4 of these stations so that no two of these halting stations are consecutive, is
A8C4B9C4C12C4−4Dnone of theseBetween Shirdee and Khandala, there are 10 intermediate stations. The number of ways in which a train can be made to stop at 4 of these stations so that no two of these halting stations are consecutive is :
A35B70C105D10
If there are 10 stations on a route and the train has to be stopped at 4 of them, then the number of ways in which the train can be stopped so that atleast two stopping stations are consecutive is
View SolutionThere are p intermediate stations on a railway line from one terminus to another . In how many ways a train can stop at 3 of these intermediate stations if no two of those stopping stations are to be consecutive ?
View SolutionThere are 10 stations enroute. A train has to be stopped at 3 of them. Let N be the no of ways is which the train can be stopped if atleast two of the stopping stations are consecutive. Find N
View SolutionBetween Shirdee and Khandala, there are 10 intermediate stations. The number of ways in which a train can be made to stop at 4 of these stations so that no two of these halting stations are consecutive is :
View SolutionThere are n stations between two cities A and B. A train is to stop at three of these n stations. What is the probaility that no two of these three stations are consecutive ?
View Solution
