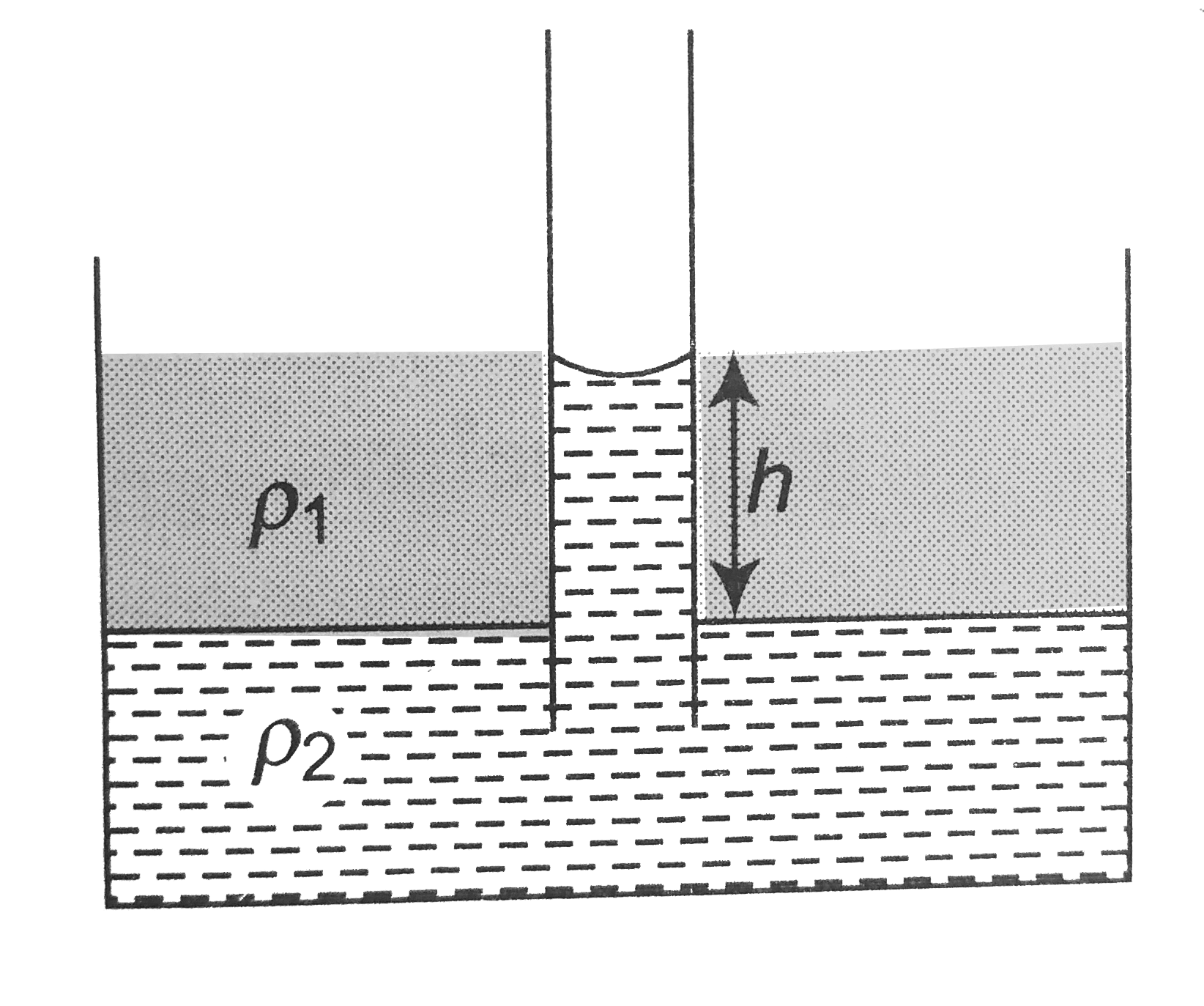
A container is partially filled with a liquid of density rho2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density rho1(rho1ltrho2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid is
A container is partially filled with a liquid of density ρ2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density ρ1(ρ1<ρ2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid is

A

1−x2x1−x2

B

1−x1x1+x2

C

x1−x2x1+x2

D

x2x1−1

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

Let V be volume of the block and p density of the block. For the first case,
ocr_image
equating (i) and (ii) , we get
x1ρ1=x2ρ1+(1−x2)ρ2
(x1−x2)ρ1=(1−x2)ρ2
ρ1ρ2=1−x2x1−x2

Updated on:21/07/2023
|
Class 12PHYSICSBULK PROPERTIES OF MATTER

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The rise of a liquid in a capillary tube is due to :

    Aosmosis
    Bsurface tension (c) viscosity
    Cviscosity
    Ddiffusion

  • The rise of a liquid in a capillary tube is due to :

    Aviscosity
    Bosmosis
    Cdiffusion
    Dsurface tension

  • The rise of liquid in a capillary tube depends on :

    Athe material of tube and nature of liquid
    Bthe length of tube
    Cthe outer radius
    Dthe inner radius of the tube