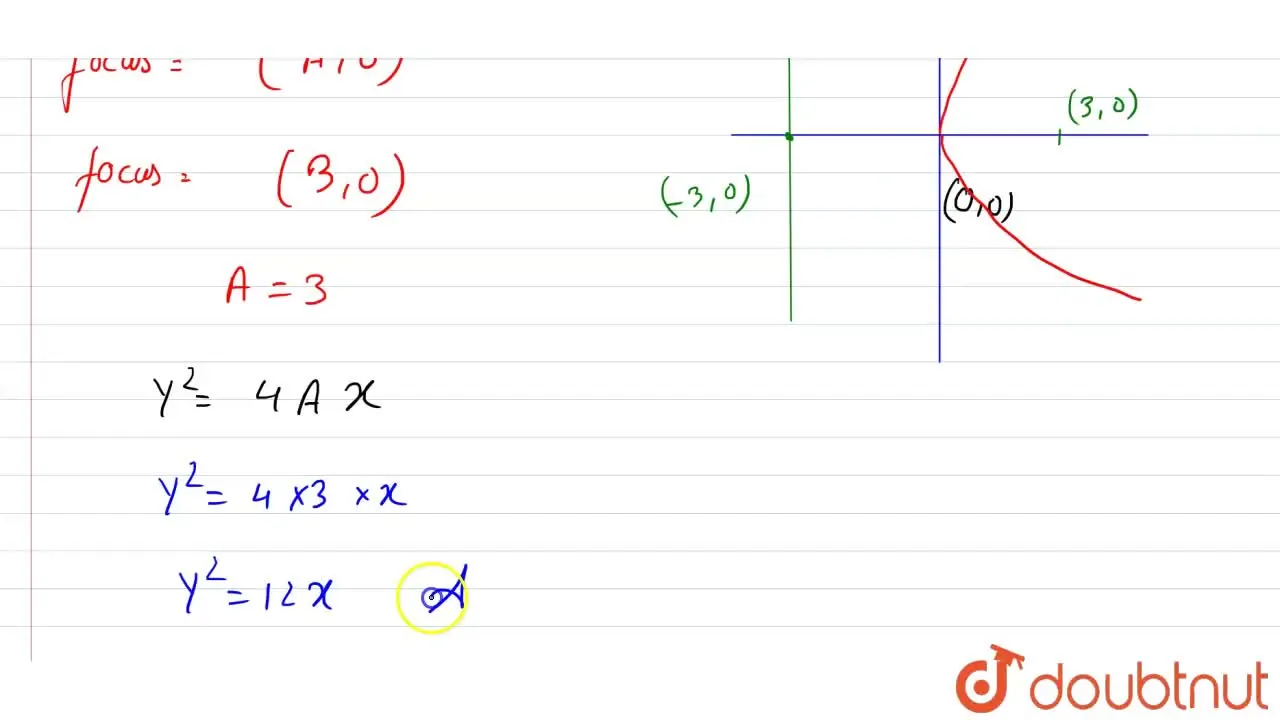
Find the equation of the parabola with focus at F(3,0) and the directrix x=-3.
Since the focus on the x-axis. So, x-axis is the axis of the parabola. Focus F(3,0) lies to the right hand side of the orgin. So, it is a right-handed parabola. Let the required equation be y2=4ax. The focus is F(a,0).So,a=3 Hence, the required equation is y2=12x.

Related Playlists
Similar Questions
Find the equation of the parabola with focus F(4, 0) and directrix x=-4.
View SolutionFind the equation of the parabola whose: focus is (3,0) and the directrix is 3x+4y=1.
View SolutionFind the equation of the parabola with focus at (3, -4) and directrix x + y - 2 = 0 .
View SolutionFind the equation of the parabola whose: focus is (0,0) and the directrix 2x−y−1=0
View SolutionFind the equation of the parabola with focus F(0,-3) and directrix y=3.
View SolutionFind the equation of the parabola with focus (2, 0) and directrix x=−2.
View SolutionFind the equation of the parabola whose focus is at (-2) and the directrix the line x−2y+3=0
View SolutionFind the equation of the parabola whose focus is (−3,2) and the directrix is x+y=4.
View SolutionFind the equation of the parabola with focus (5, 0), and directrix x=−5. Also, find the length of the latus rectum.
View SolutionFind the equation of the parabola whose focus is at (−1,1) and the directrix is x+y+1=0.
View Solution