Question

Diagonals of a trapezium $ABCD$ with $AB\mid \mid DC$ intersect each other at the point $O$. If $AB=2CD$, find the ratio of the areas of $△AOB\text{and}△COD$.

Options

A. `4:1`
B. `1:4`
C. `5:1`
D. `1:5`

Answer

A

Explanation

Its diagonals intersect each other at the poing O.
TO FIND `(ar(Delta ABC))/(ar (Delta COD))`

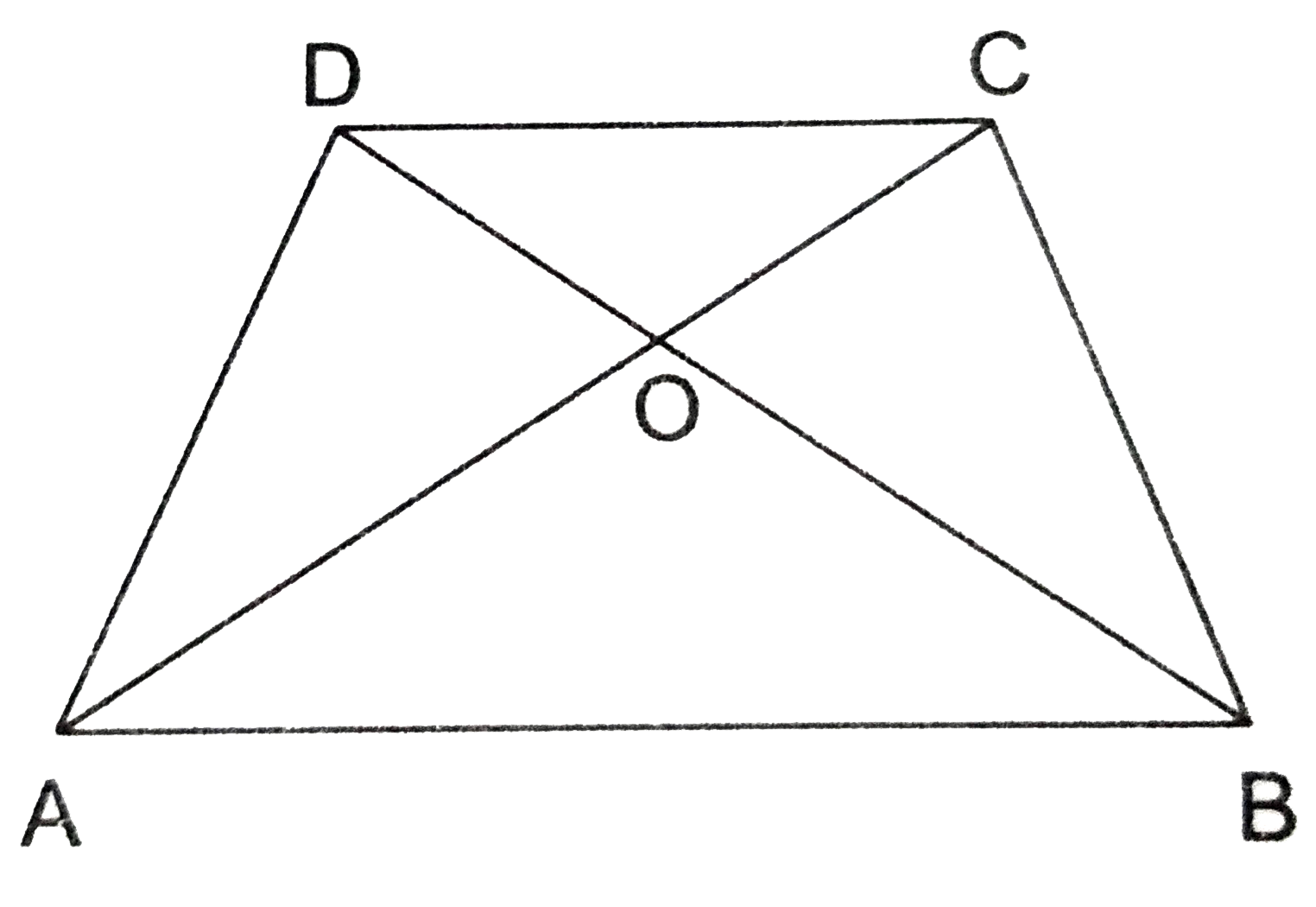
METHOD OF SOLUTION In `Delta AOB and Delta COD` , we have
`angle AOB= angle COD` [ ver. Opp. `angle`]
`angle OAB= angle OCD` [ alt. int `angle`]
`:. Delta AOB~ Delta COD` [ by AA- similartiy]
We know that the ratior of the areas of two similar triangles is equal to the ratio of the sequence of the corresponding sides.
`:.(ar(Delta ABC))/(ar (Delta COD))=(AB^(2))/(DC^(2))=((2xxDC)^(2))/(DC^(2)) " " [ :. AB= 2DC]`
`=(4xxDC^(2))/(DC^(2))=(4)/(1)`
Hence, `ar (Delta AOB) : ar (Delta COD)= 4:1`