M and N are points on the sides AC and BC respectively of a ΔABC. In ech of the fopllowing cases, state whether MN∣∣AB.
(i) CM=4.2cm.MA=2.8cm,NB=3.6cm,5.7
(ii) CB=6.92cm,CN=1.04cm,Ca=1.73cm,CM=0.26cm
(iii) CM=5.1cm,CA=6.8cm,CB=5.6cm,NB=1.4cm.
(i) We have
CMMA=4.22.8=32andCNNB=5.73.6=1912
Since CMMA≠CNNB
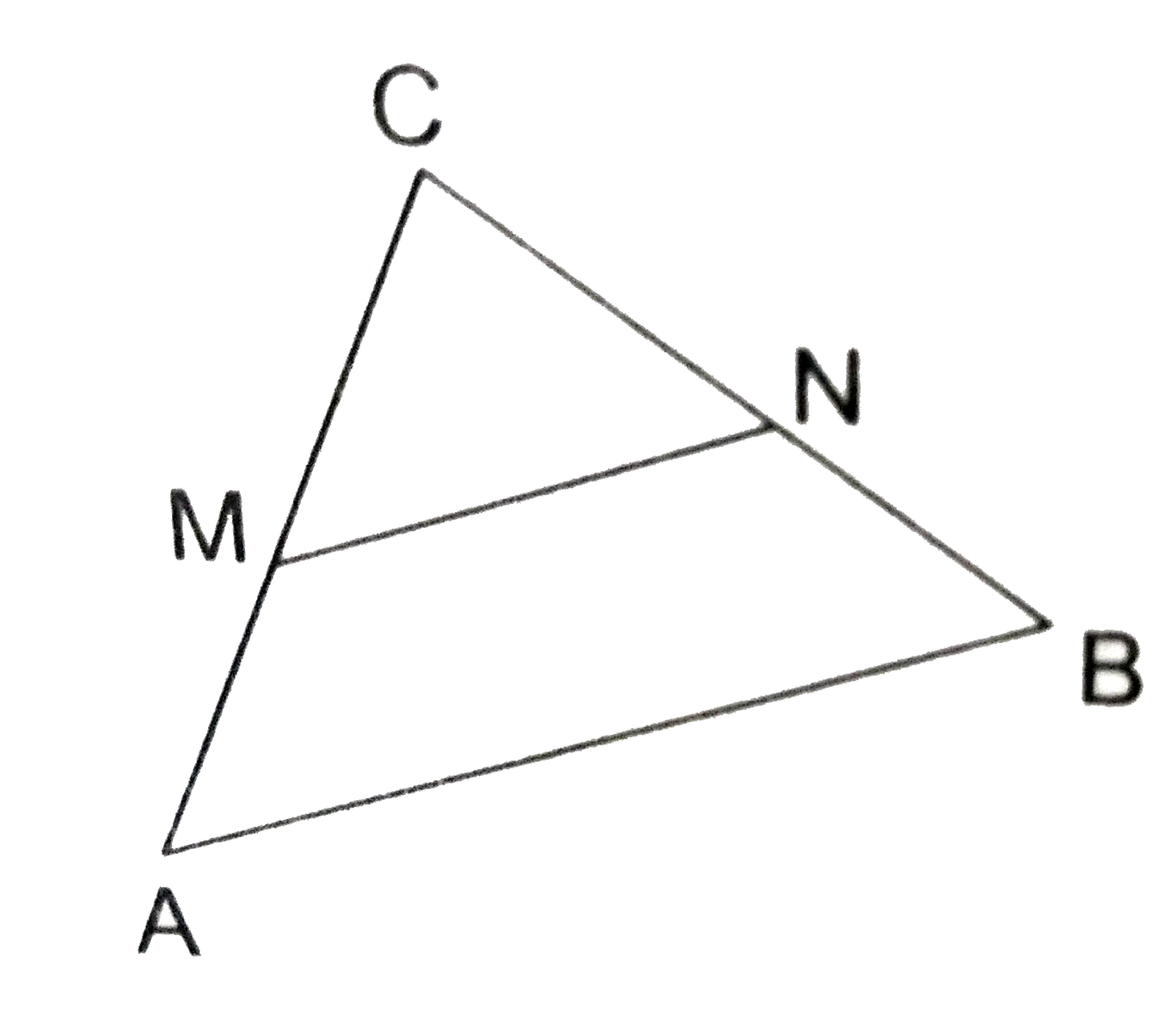
(ii) We have,
MA=CA−CM=(1.73−0.26)cm=1.47cm
and NB=CB−CN=(6.92−1.04)cm=5.88 cm.
∴CMMA=0.261.47=26147andCNNB=1.045.88=26147.
Clearly, MCMA=NCNB and so MN∣∣AB [ by the converse of Thales' theorem]
(iii) We have
MA=CA−CM=(6.8−5.1)cm=1.7 cm
and CN=CB−NB=(5.6−1.4)cm=4.2cm
∴CMNA=5.11.7=31andCNNB=4.21.4=31
Clearly, ,CMMA=CNNBandso,MN∣∣AB. [ by the converse of Thales' theorm]
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Video
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
D and E are points on the sides AB and AC respectively of ΔABC. In each of the following cases, determine whetheer DE∣∣BC or not. (i) AD=5.7cm,DB=9.5cm,Ae=4.8cmandEC=8cm. (ii) AB=11.7cm,AC=11.2cm,AD=6.5cmandAE=41.2cm, (iii) AB=10.8cm,AD=6.3cm,AC=9.6cmandEC=4cm. (iv) AD=7.2cmAE=6.4cm.AB=12cmandAC=10cm.
View SolutionM and N are points on sides AC and BC respectively of a △ABC. State whether MN||BC if CM = 4.2 cm, MA= 2.8 cm , NB = 3.6 cm, CN = 5.7 cm.
View SolutionE and F are points on the sides PQ and PR respectively of ΔPQR. For each of the following cases, state whether EF || QR:
(i) PE = 3.9 cm. EQ = 3 cm. PF = 3.6 cm and FR = 2.4
(ii) PE = 4 cm. QE = 4.5 cm. PF = S cm and RF = 9 cm
(iii) PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm
View SolutionIf D and E are points on the sides AB and AC respectively of DetlaABC such that AB=5.6cm,AD=1.4cm,AC=7.2cmandAE=1.8cm. Show that DE∣∣BC.
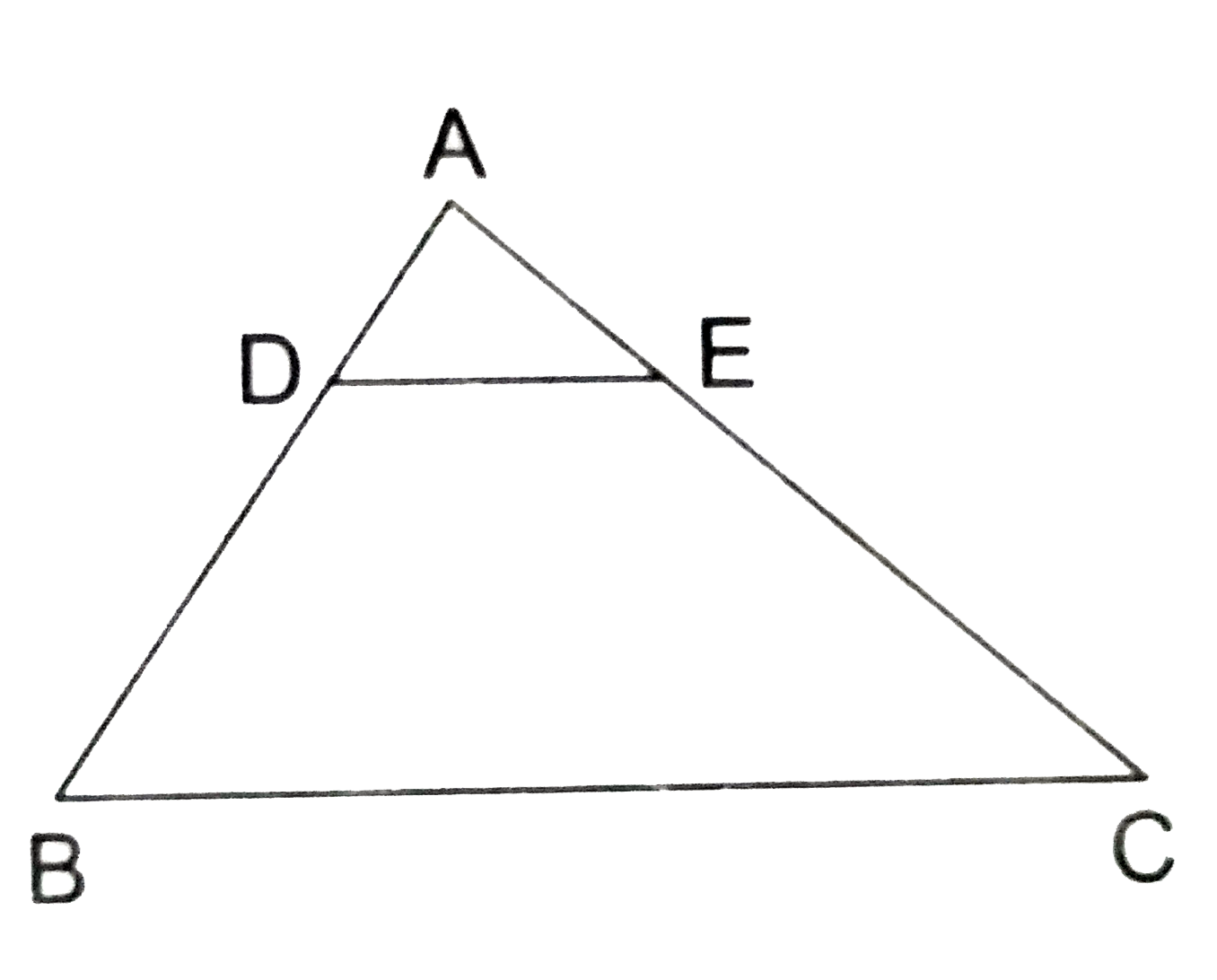
View SolutionD and E are the points on the sides AB and AC respectively of a ABC such that: AD=8cm, DB=12cm, AE=6cm and CE=9cm . Prove that BC=5/2 DE .
View SolutionD and E are points on the sides AB and AC respectively of a △ABC such that DE||BC
(i) If AD=3.6cm,AB=10cm and AE=4.5cm, find EC and AC .
(ii) If AB=13.3cm,AC=11.9cm and EC=5.1cm," find AD
(iii) If ADDB=47 and AC=6.6cm find AE
(iv) If ADAB=815 and EC=3.5cm find AE
View SolutionFind whether the following measures can be the sides of triangles.
(i) 5 cm, 7 cm, 10 cm
(ii) 3 cm, 6 cm, 5 cm
(iii) 2 cm, 7 cm, 14 cm
View SolutionName the types of following triangles :
Δ ABC with AB = 8.7 cm, AC = 7 cm and BC = 6 cm.
View SolutionIn △ABC right angled at C
( i) AB= 1.7 cm, BC= 1.5 cm, find CA (ii) CA=2.4 cm, AB = 4.0 cm, find BC
View SolutionIn which of the following cases, a right triangle cannot be constructed? 12 cm, 5 cm, 13 cm (b) 8 cm, 6 cm, 10 cm 5 cm, 9 cm 11 cm (d) None of these
View Solution
