How many different products can be obtained by multiplying two or more of the numbers 3, 5,7, 11 (without repetition)?
Video Solution
Text Solution
The correct Answer is:11
Required number of products
= number of ways of selecting 2 or 3 or 4 numbers out of 3, 5, 7, 11
=(.4C2+.4C3+.4C4)=(6+4+1)=11.
|
Topper's Solved these Questions
- COMBINATIONSBOOK - RS AGGARWALCHAPTER - COMBINATIONSEXERCISE - Exercise 9C
5 Videos
- COMBINATIONSBOOK - RS AGGARWALCHAPTER - COMBINATIONSEXERCISE - Exercise 9D
18 Videos
- COMBINATIONSBOOK - RS AGGARWALCHAPTER - COMBINATIONSEXERCISE - Exercise 9A
14 Videos
- CIRCLE BOOK - RS AGGARWALCHAPTER - CIRCLE EXERCISE - EXERCISE 21 B
18 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - RS AGGARWALCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - EXERCISE 5 G
28 Videos
Similar Questions
Knowledge Check
How many different four digit numbers can be formed using digits 1, 2, 3, 4, 5, 6, 7, 8, 9 without repetition such that the digit 5 appears exactly once?
A1243B1234C1344D1355How many different four digit numbers can be formed using digits 1, 2, 3, 4, 5, 6, 7, 8, 9 without repetition such that the digit 5 appears exactly once?
A1243B1234C1344D1355
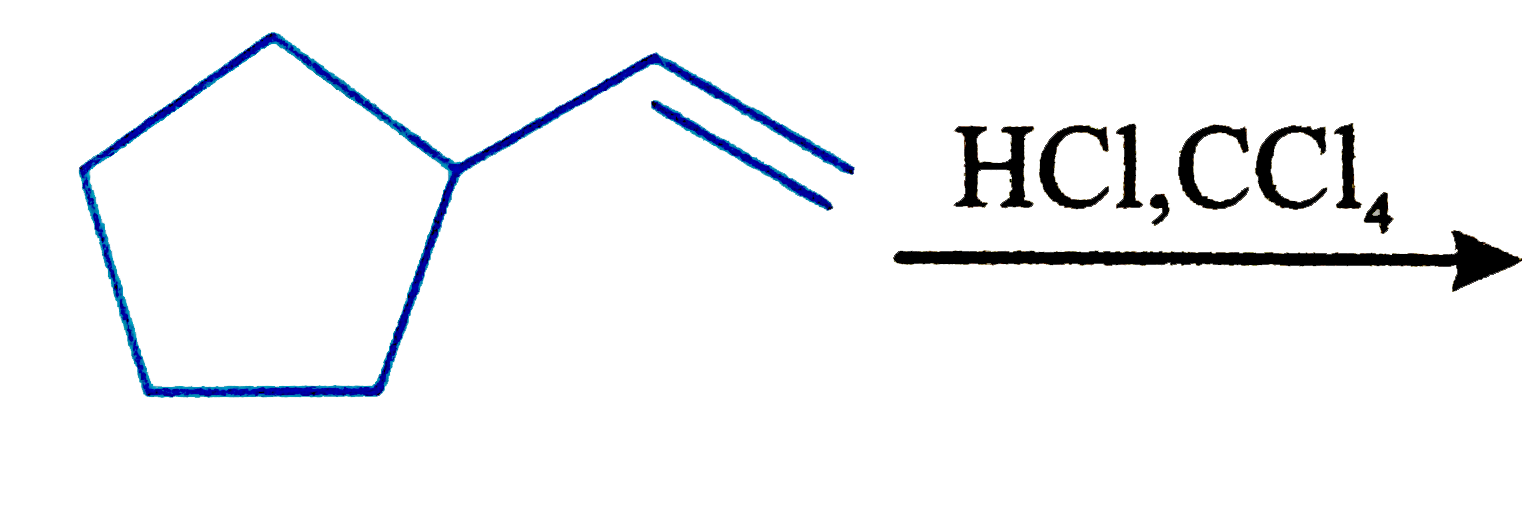
How many different products (exluding stereosiomer) can be obtained by following reaction
View SolutionHow many different five digit numbers can beformed from the digit 1,2,3,4 and 5 if the number is odd and no repetitions are allowed :
View SolutionHow many different 3-digit numbers can be formed by using the digits 0, 2, 5 without repeating any digit in the number?
View SolutionHow many different five digit numbers can beformed from the digit 1,2,3,4 and 5 if the number is even and repetitions are allowed :
View SolutionHow many number of monochlorinated products are obtained by 3-ethylpentane.
View SolutionHow many different 4-digit number can be formed with the digits 1,2,3,4 without any repetition ? Also find their sum.
View Solution
