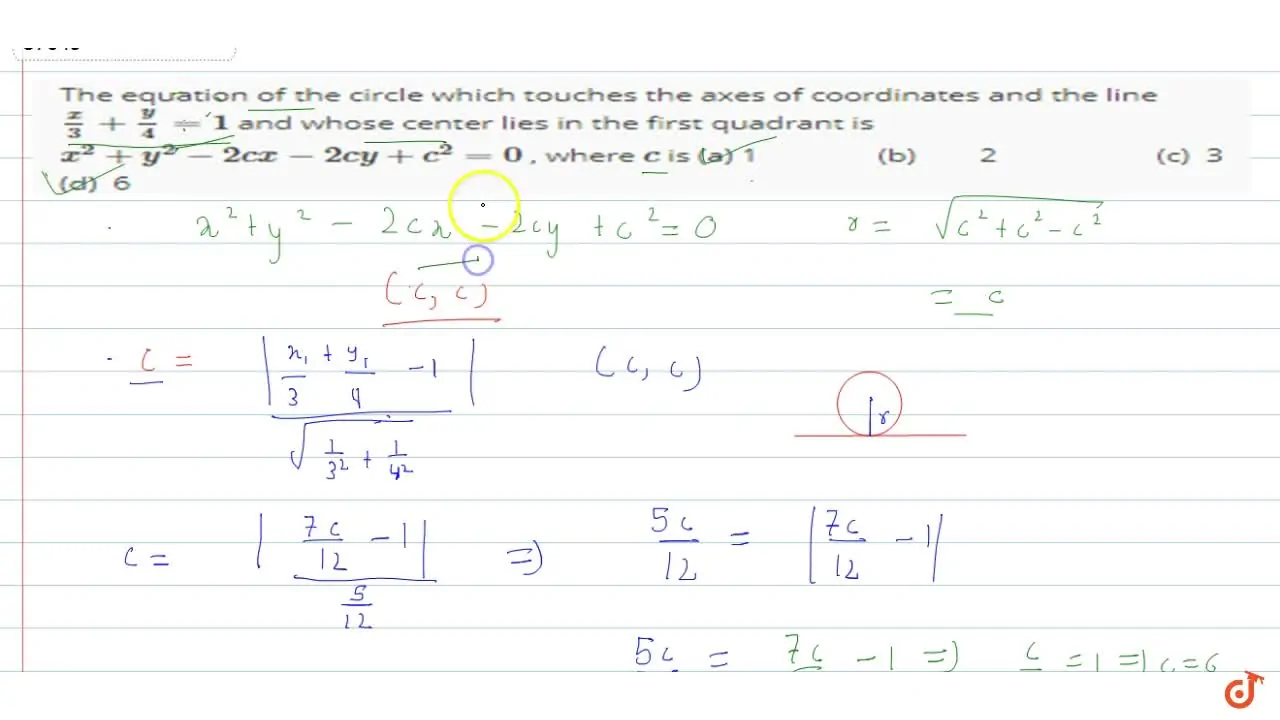
The equation of the circle which touches the axes of coordinates and

The equation to a circle whose centre lies at the point (-2,1) and which touches the line $3 x - 2 y - 6 = 0$ at (4, 3) is
A $x^{2} + y^{2} + 4 x - 2 y - 35 = 0$
B $x^{2} + y^{2} - 4 x + 2 y + 35 = 0$
C $x^{2} + y^{2} + 4 x + 2 y + 35 = 0$
D $x^{2} + y^{2} - 4 x - 2 y + 35 = 0$
Two circles are given as $x^{2} + y^{2} + 14 x - 6 y + 40 = 0$ and $x^{2} + y^{2} - 2 x + 6 y + 7 = 0$ with their centres as $C_{1}$ and $C_{2}$ . If equation of another circle whose centre $C_{3}$ lies on the line 3x+4y-16=0 and touches the circle $C_{1}$ externally and also $C_{1} C_{2} + C_{2} C_{3} + C_{3} C_{1}$ is minimum is $x^{2} + y^{2} + a x + b y + c = 0$ then the value of (a+b+c) is
A2
B8
C16
D0

The equation of the circle which touches the axes of coordinates and the line $\frac{x}{3} + \frac{y}{4} = 1$ and whose center lies in the first quadrant is $x^{2} + y^{2} - 2 c x - 2 c y + c^{2} = 0$ , where $c$ is (a) 1 (b) 2 (c) 3 (d) 6