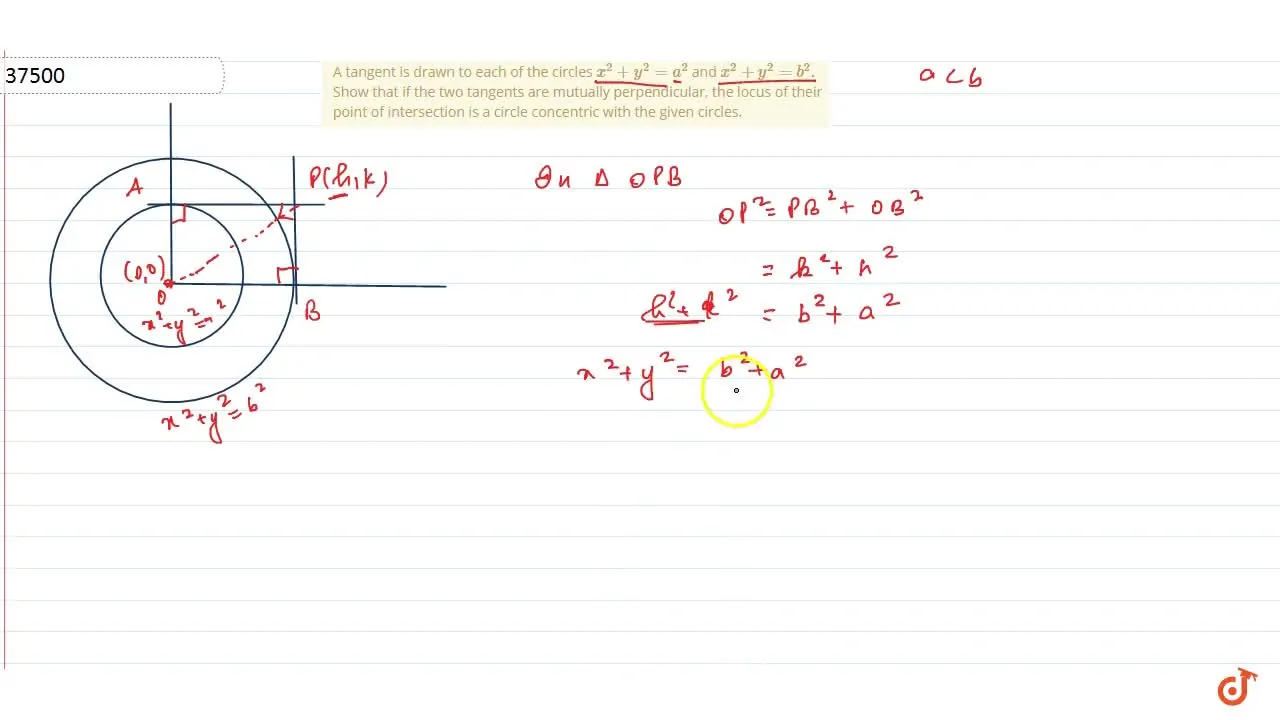
A tangent is drawn to each of the circles $x^{2} + y^{2} = a^{2}$ and $x^{2} + y^{2} = b^{2} .$ Show that if the two tangents are mutually perpendicular, the locus of their point of intersection is a circle concentric with the given circles.

Video Solution

Text Solution

Verified by Experts

In the figure, from variable point P(h,k) tangents PA and PB are drawn to two circles $x^{2} + y^{2} = a^{2}$ and $x^{2} + y^{2} = b^{2}$ . Tangents PA and PB are perpendicuarl .
Clearly, OAPB is a reactangle.
$∴ O P^{2} = O B^{2} + B P^{2} = h^{2} + k^{2} = a^{2} + b^{2}$

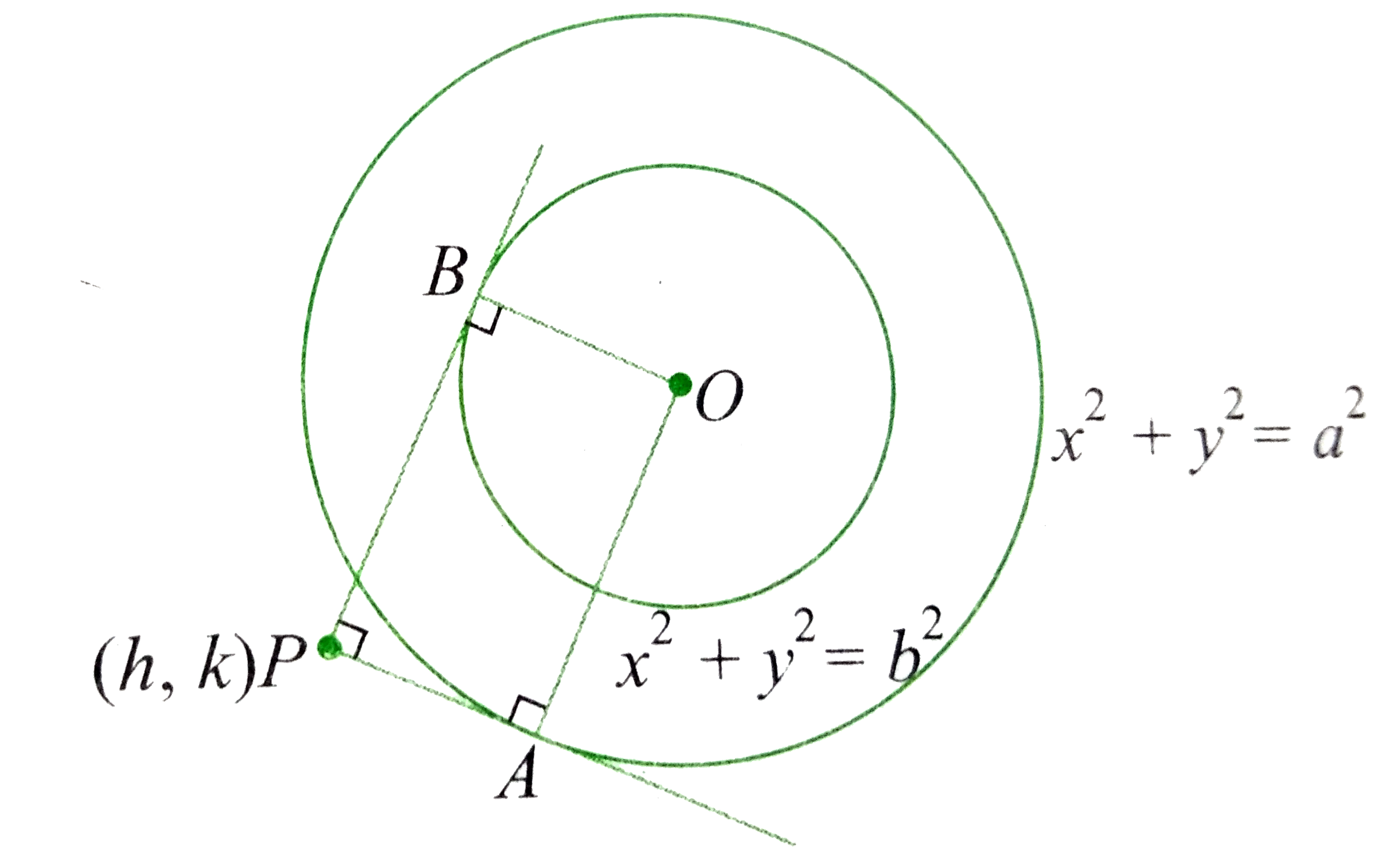
Therefore, required locus is $x^{2} + y^{2} = a^{2} + b^{2}$ , which is concentric circle with given circles.

Updated on:21/07/2023

Locus of the point of intersection of perpendicular tangents to the circle $x^{2} + y^{2} = 16$ is
A $x^{2} + y^{2} = 8$
B $x^{2} + y^{2} = 32$
C $x^{2} + y^{2} = 64$
D $x^{2} + y^{2} = 16$
Locus of the point of intersection of perpendicular tangents to the circle $x^{2} + y^{2} = 16$ is
A $x^{2} + y^{2} = 8$
B $x^{2} + y^{2} = 32$
C $x^{2} + y^{2} = 64$
D $x^{2} + y^{2} = 16$
Locus of the point of intersection of perpendicular tangents to the circle $x^{2} + y^{2} = 16$ is
A $x^{2} + y^{2} = 8$
B $x^{2} + y^{2} = 32$
C $x^{2} + y^{2} = 64$
D $x^{2} + y^{2} = 16$