If a circle passes through the points of intersection of the coordinate axes with the lines λx−y+1=0 and x−2y+3=0, then the value of λ is............
The correct Answer is:λ=2
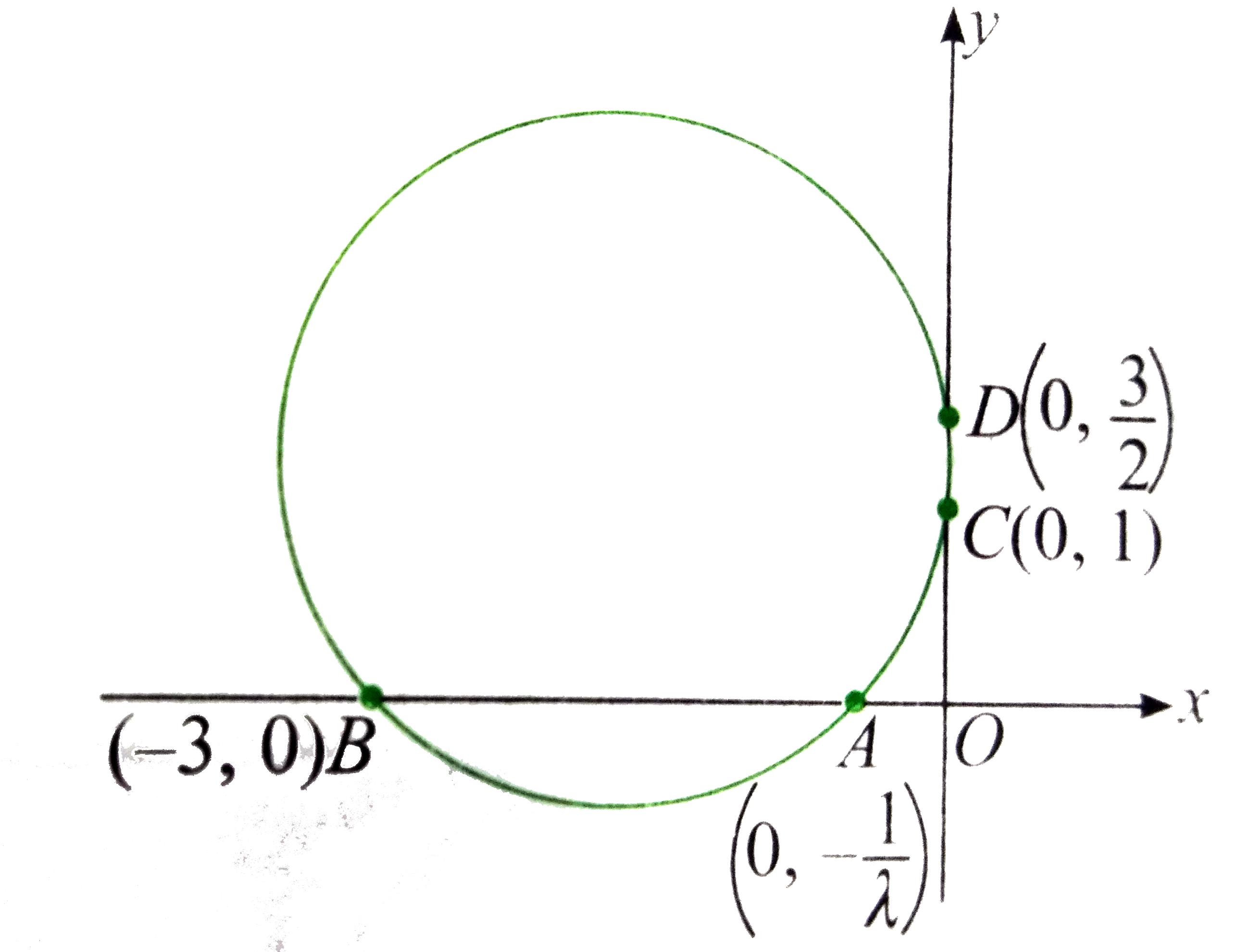
Line λx−y+1 meets x-axis at A(−1/λ,0) and y-axis D(0,1).
Line x−2y+3=0 meets x-axis at B(−3,0) and y-axis at D(0,3/2).
Since points B,A,C and D lie on a circle, we must have
OA×OB=OC×OD
⇒1λ×3=1×32
⇒λ=2
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.4
14 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.5
12 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.2
5 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
If a circle passes through the points of intersection of the coordinate axes with the lines λx−y+1=0 and x−2y+3=0, then the value of λ is............
View SolutionIf a circle passes through the points ofintersection ofthe axes with the lines λx−y+1=0andx−2y+3=0,λ>0 and radius ofthe circle is KL√2 then (K+L) is
View Solution
Knowledge Check
If a circle passes through the points of intersection of the co-ordinate axes with the lines λx−y+1=0andx−2y+3=0, then the value of λ is
A2B1/3C6D3If a circle passes through the points of intersection of the lines 2x−y+1=0 and x+λy−3=0 with the axes of reference then the value of λ is :
A1/2B2C1D−2The straight line passing through the point of intersection of the straight line x+2y−10=0and2x+y+5=0 is
A5x−4y=0B5x+4y=0C4x−5y=0D4x+5y=0
If a circle passes through the points of intersection of the lines 2x−y+1=0andx+λy−3=0 with the axes of reference then the value of λ is
View SolutionIf a circle passes through the point of intersection of the lines x+y+1=0 and x+λy−3=0 with the coordinate axis, then value of λ is
View SolutionIf a circle passes through the points where the lines 3kx−2y−1=0 and 4x−3y+2=0 meet the coordinate axes then k=
View SolutionIf the straight line ax+y+6=0 passes through the point of| intersection of the lines x+y+4=0and2x+3y+10=0,find α.
View SolutionIf the line ky=x+1 passes through the point on intersection of the two lines 2x-3y+5=0 and 3x+2y+1=0, then :k=
View Solution
