Show, by vector methods, that the angularbisectors of a triangle are concurrent and find an expression for the position vector of the point of concurrency in terms of the position vectors of the vertices.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $I = \frac{α \to a + β \to b + γ \to c}{α + β + γ}$

Let AD be the angular bisector of angle A. Let BC , AC and AB are $α, β$ and $γ$ respectively . Then $, \frac{B D}{D C} = \frac{γ}{β}$
Hence position vector of $D = \frac{γ \to c + β \to b}{γ + β}$ . On AD , there lies a point I which divides it in ratio $γ + β : α$
Now position vector of $I = \frac{α \to a + β \to b + γ \to c}{α + β + γ}$
Which is symmetric in $\to a, \to b, \to c α, β and γ$

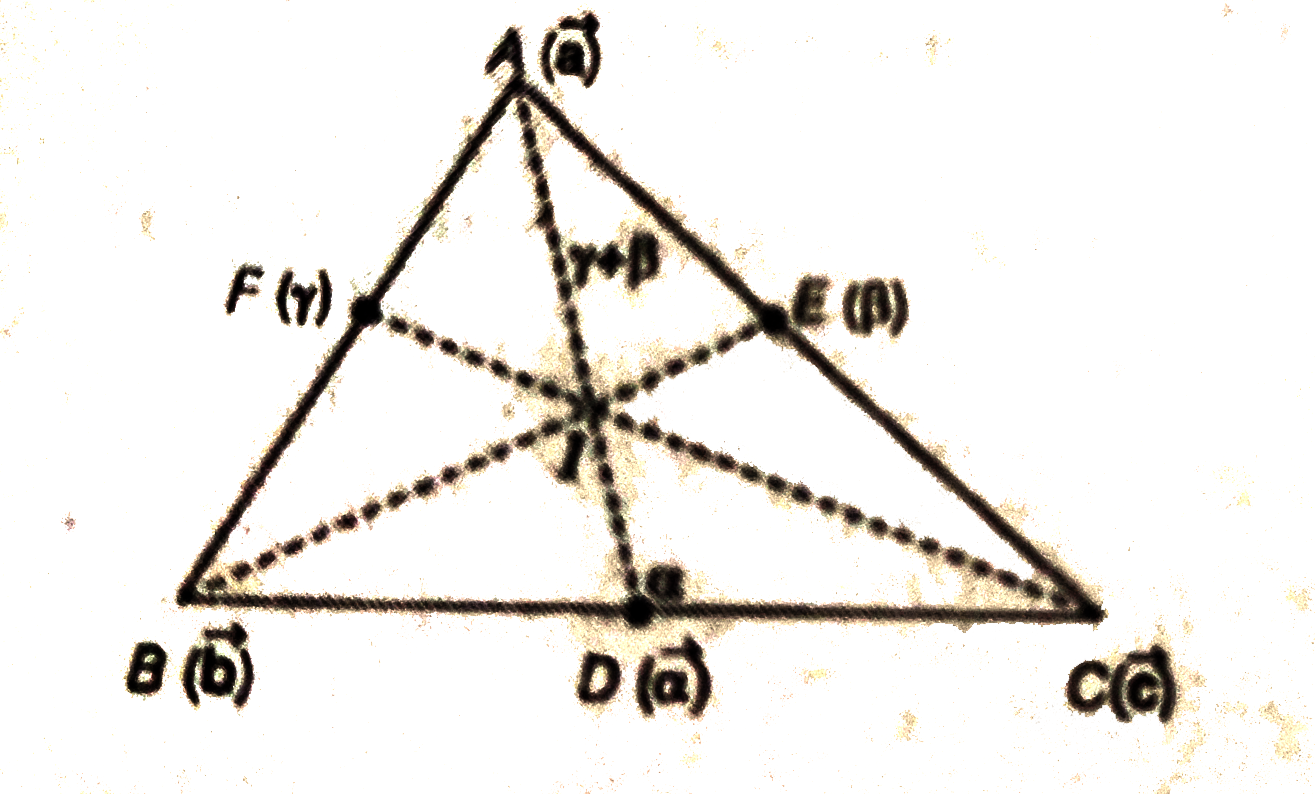
Hence I lies on every angle bisector and angle bisectors are concurrent .
Here $α = ∣ ∣ \to b - \to c ∣ ∣, β = | \to a - \to c |, γ = ∣ ∣ \to a - \to b ∣ ∣ .$

|

Updated on:21/07/2023

Class 12 MATHS VECTOR ALGEBRA

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If a and b are position vector of two points A,B and C divides AB in ratio 2:1, then position vector of C is
A $\frac{a + 2 b}{3}$
B $\frac{2 a + b}{3}$
C $\frac{a + 2}{3}$
D $\frac{a + b}{2}$

IIT JEE PREVIOUS YEAR-VECTOR ALGEBRA-Scalar Product of two vectors (Analytical & Descriptive Questions)

Home
Ask Doubt
Profile