Show that the perpendicular bisectors of the sides of a triangle are concurrent.
To show that the perpendicular bisectors of the sides of a triangle are concurrent, we will follow a systematic approach. Here’s a step-by-step solution:
Step 1: Define the Triangle
Let triangle ABC have the following coordinates:
- A(0,0)
- B(a,0)
- C(0,b)
Step 2: Find the Midpoints of the Sides
Next, we calculate the midpoints of the sides of the triangle:
- Midpoint D of side AB:
D=(0+a2,0+02)=(a2,0)
- Midpoint E of side BC:
E=(a+02,0+b2)=(a2,b2)
- Midpoint F of side CA:
F=(0+02,b+02)=(0,b2)
Step 3: Find the Slopes of the Sides
Now, we find the slopes of the sides:
- Slope of AB (horizontal line):
mAB=0
- Slope of BC:
mBC=b−00−a=−ba
- Slope of CA:
mCA=0−b0−0 (undefined, vertical line)
Step 4: Find the Slopes of the Perpendicular Bisectors
The slopes of the perpendicular bisectors are the negative reciprocals of the slopes of the sides:
- Slope of the perpendicular bisector of AB:
mAD=undefined (vertical line)
- Slope of the perpendicular bisector of BC:
mBE=ab
- Slope of the perpendicular bisector of CA:
mCF=0
Step 5: Write the Equations of the Perpendicular Bisectors
Using point-slope form y−y1=m(x−x1):
- Equation of the perpendicular bisector of AB (vertical line):
x=a2
- Equation of the perpendicular bisector of BC:
y−b2=ab(x−a2)
- Equation of the perpendicular bisector of CA (horizontal line):
y=b2
Step 6: Solve the Equations to Find the Point of Concurrency
To find the point of concurrency, we can solve the equations of the perpendicular bisectors:
1. From the equation of the perpendicular bisector of CA:
y=b2
2. Substitute y=b2 into the equation of the perpendicular bisector of BC:
b2−b2=ab(x−a2)
This simplifies to:
0=ab(x−a2)
Thus, x=a2.
Step 7: Conclusion
The point of concurrency of the perpendicular bisectors of the triangle is:
(a2,b2)
This shows that the perpendicular bisectors of the sides of triangle ABC are concurrent.
Let triangle ABC have the following coordinates:
- A(0,0)
- B(a,0)
- C(0,b)
Next, we calculate the midpoints of the sides of the triangle:
- Midpoint D of side AB:
D=(0+a2,0+02)=(a2,0)
- Midpoint E of side BC:
E=(a+02,0+b2)=(a2,b2)
- Midpoint F of side CA:
F=(0+02,b+02)=(0,b2)
Now, we find the slopes of the sides:
- Slope of AB (horizontal line):
mAB=0
- Slope of BC:
mBC=b−00−a=−ba
- Slope of CA:
mCA=0−b0−0 (undefined, vertical line)
The slopes of the perpendicular bisectors are the negative reciprocals of the slopes of the sides:
- Slope of the perpendicular bisector of AB:
mAD=undefined (vertical line)
- Slope of the perpendicular bisector of BC:
mBE=ab
- Slope of the perpendicular bisector of CA:
mCF=0
Using point-slope form y−y1=m(x−x1):
- Equation of the perpendicular bisector of AB (vertical line):
x=a2
- Equation of the perpendicular bisector of BC:
y−b2=ab(x−a2)
- Equation of the perpendicular bisector of CA (horizontal line):
y=b2
To find the point of concurrency, we can solve the equations of the perpendicular bisectors:
1. From the equation of the perpendicular bisector of CA:
y=b2
2. Substitute y=b2 into the equation of the perpendicular bisector of BC:
b2−b2=ab(x−a2)
This simplifies to:
0=ab(x−a2)
Thus, x=a2.
The point of concurrency of the perpendicular bisectors of the triangle is:
(a2,b2)
This shows that the perpendicular bisectors of the sides of triangle ABC are concurrent.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The point at which the perpendicular bisectors of the sides of a triangle intersect is known as ________.
Acircum-centreBorthocentereCcentroidDin-centreThe point at which the perpendicular bisectors of the sides of a triangle intersect is known as ________.
Acircum-centreBorthocenterCcentroidDin-center
Prove that the internal bisectors of the angles of a triangle are concurrent
View SolutionProve that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
View SolutionConsider the following statements :
1. The point of intersection of the perpendicular bisectors of the sides of a triangle may lie outside the triangle.
2. The point of intersection of the perpendiculars drawn from the vertices to the opposite sides of a triangle may lie on two sides.
Which of the above statements is/are correct ?
View SolutionThe number of triangles that can be formed by the perpendiculars bisectors of the sides of a triangle is
View SolutionThe equations of the perpendicular bisectors of the sides AB&AC of a triangle ABC are x−y+5=0&x+2y=0. find the equation of the bc
View SolutionA, B and C are three points on a circle. Prove that the perpendicular bisectors of AB, BC and CA are concurrent.
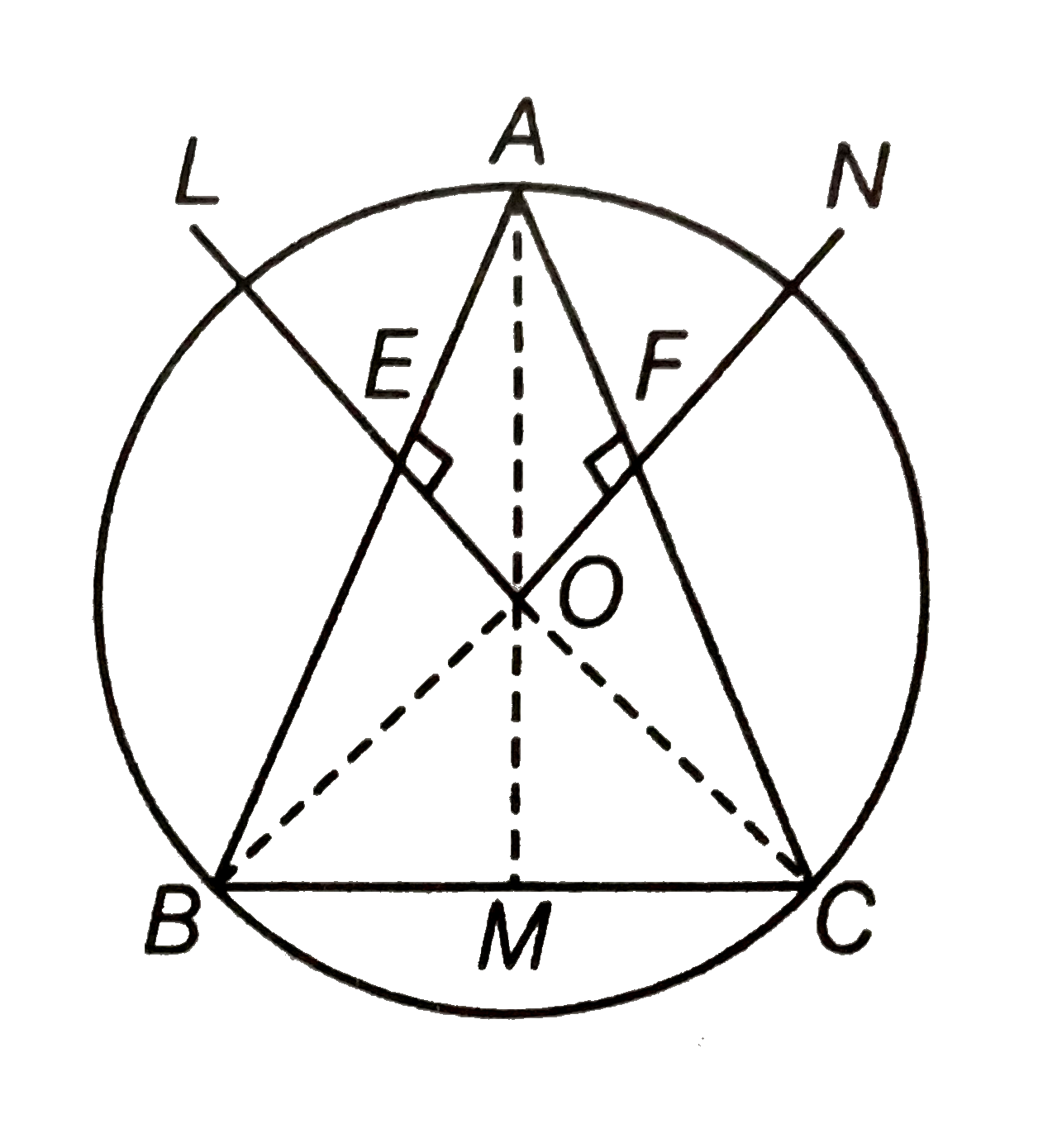
View Solution
