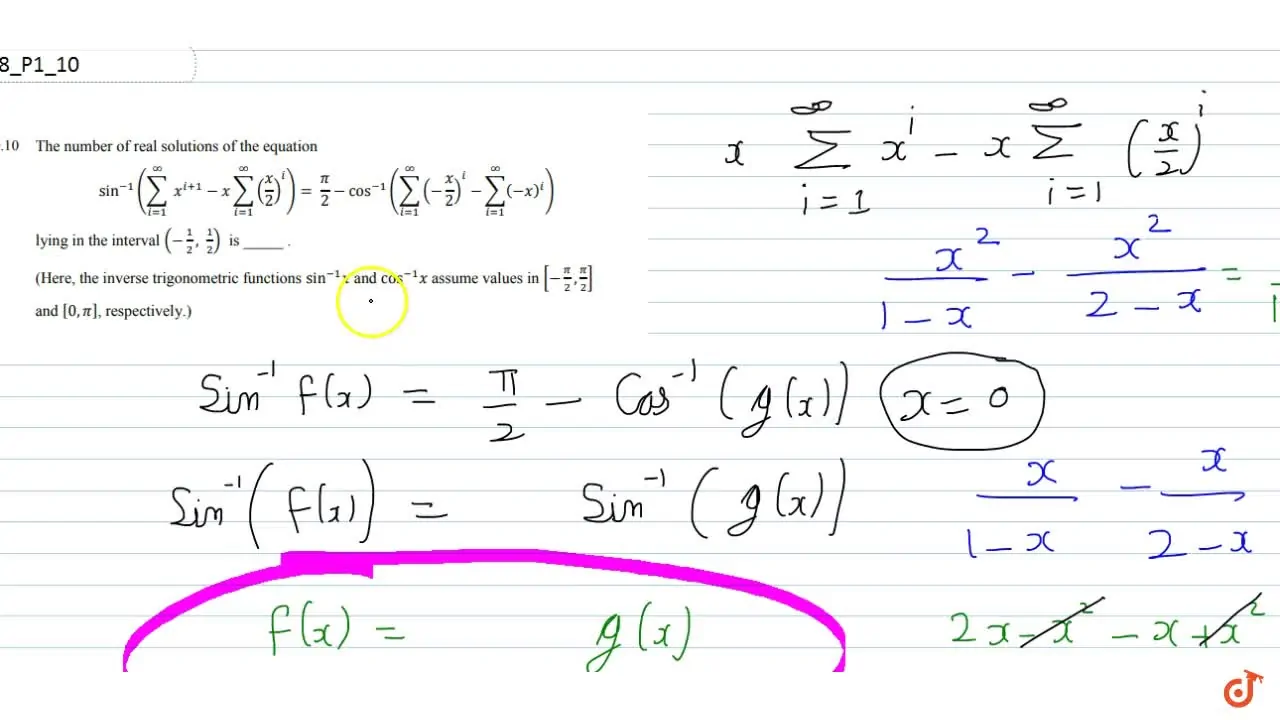
The number of real solutions of the equation
${sin}^{- 1} (\infty \sum i = 1 x^{i + 1} - x \infty \sum i = 1 {(\frac{x}{2})}^{i}) = \frac{π}{2} - {cos}^{- 1} (\infty \sum i = 1 {(- \frac{x}{2})}^{i} - \infty \sum i = 1 {(- x)}^{i})$ lying in the interval $(- \frac{1}{2}, \frac{1}{2})$ is ............... .
(Here, the inverse trigonometric function ${sin}^{- 1} x and {cos}^{- 1} x$ assume values in $[- \frac{π}{2}, \frac{π}{2}] and [0, π]$ , respectively.)

Video Solution

Text Solution

Verified by Experts

The correct Answer is:2

We have ,
${sin}^{- 1} (\infty \sum i = 1 x^{i + 1} - x \infty \sum i = 1 {(\frac{x}{2})}^{i})$
$= \frac{π}{2} - {cos}^{- 1} (\infty \sum i = 1 {(\frac{- x}{2})}^{i} - \infty \sum i = 1 {(- x)}^{i})$
$\Rightarrow {sin}^{- 1} [\frac{x^{2}}{1 - x} - \frac{x \cdot \frac{x}{2}}{1 - \frac{x}{2}}]$
$= \frac{π}{2} - {cos}^{- 1} ⎡ ⎣ \frac{\frac{- x}{2}}{1 + \frac{x}{2}} - \frac{(- x)}{1 + x} ⎤ ⎦$
$[∵ \infty \sum i = 1 x^{i + 1} = x^{2} + x^{3} + x^{4} + ... = \frac{x^{2}}{1 - x}]$

using sum of infinite terms of GP
$\Rightarrow {sin}^{- 1} [\frac{x^{2}}{1 - x} - \frac{x}{2 + x}]$
$\Rightarrow {sin}^{- 1} [\frac{x^{2}}{1 - x} - \frac{x^{2}}{2 - x}] = {sin}^{- 1} (\frac{x}{1 + x} - \frac{x}{2 + x})$
$[∵ {sin}^{- 1} x = \frac{π}{2} - {cos}^{- 1} x]$
$\Rightarrow \frac{x^{2}}{1 - x} - \frac{x^{2}}{2 - x} = \frac{x}{1 + x} - \frac{x}{2 + x}$
$\Rightarrow x^{2} (\frac{2 - x - 1 + x}{(1 - x) (2 - x)}) = x \frac{(2 + x - 1 - x)}{(1 + x) (2 + x)}$
$\Rightarrow \frac{x}{2 - 3 x + x^{2}} = \frac{1}{2 + 3 x + x^{2}} or x = 0$
$\Rightarrow x^{3} + 3 x^{2} + 2 x = x^{2} - 3 x + 2$
$\Rightarrow x^{3} + 2 x^{2} + 5 x - 2 = 0 or x - 0$
Let $f (x) = x^{3} + 2 x^{2} + 5 x - 2$
$f' (x) = 3 x^{2} + 4 x + 5$
$f' (x) > 0, \forall \in R$
$∴ x^{3} + 2 x^{2} + 5 x - 2$ has only one real roots
Therefore, total number of real solution is 2.

Updated on:21/07/2023

|

Class 12 MATHS FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

The sum of solutions of the equation $2 {sin}^{- 1} \sqrt{x^{2} + x + 1} + {cos}^{- 1} \sqrt{x^{2} + x} = \frac{3 π}{2}$ is :
A0
B-1
C1
D2
If $2 n \sum i = 1 {cos}^{- 1} x_{i} = 0$ , then $2 n \sum i = 1 x_{i}$ is :
An
B2n
C $\frac{n (n + 1)}{2}$
DNone of these
If $\infty \sum r = 1 \frac{1}{{(2 r - 1)}^{2}} = \frac{π^{2}}{8}$ then the value of $\infty \sum r = 1 \frac{1}{r^{2}}$ is
A $\frac{π^{2}}{8} + \frac{1}{4} x$
B $\frac{π^{2}}{4} + \frac{1}{3} x$
C $\frac{π^{2}}{8} - \frac{1}{4} x$
D $\frac{π^{2}}{4} + \frac{2}{3} x$

IIT JEE PREVIOUS YEAR-INVERSE CIRCULAR FUNCTIONS -PROPERTIES OF INVERSE FUNCTIONS (NUMERICAL VALUE BASED)

The number of real solutions of the equation sin ^(-1)( sum(i=1)^(oo...
08:16

Home
Ask Doubt
Profile