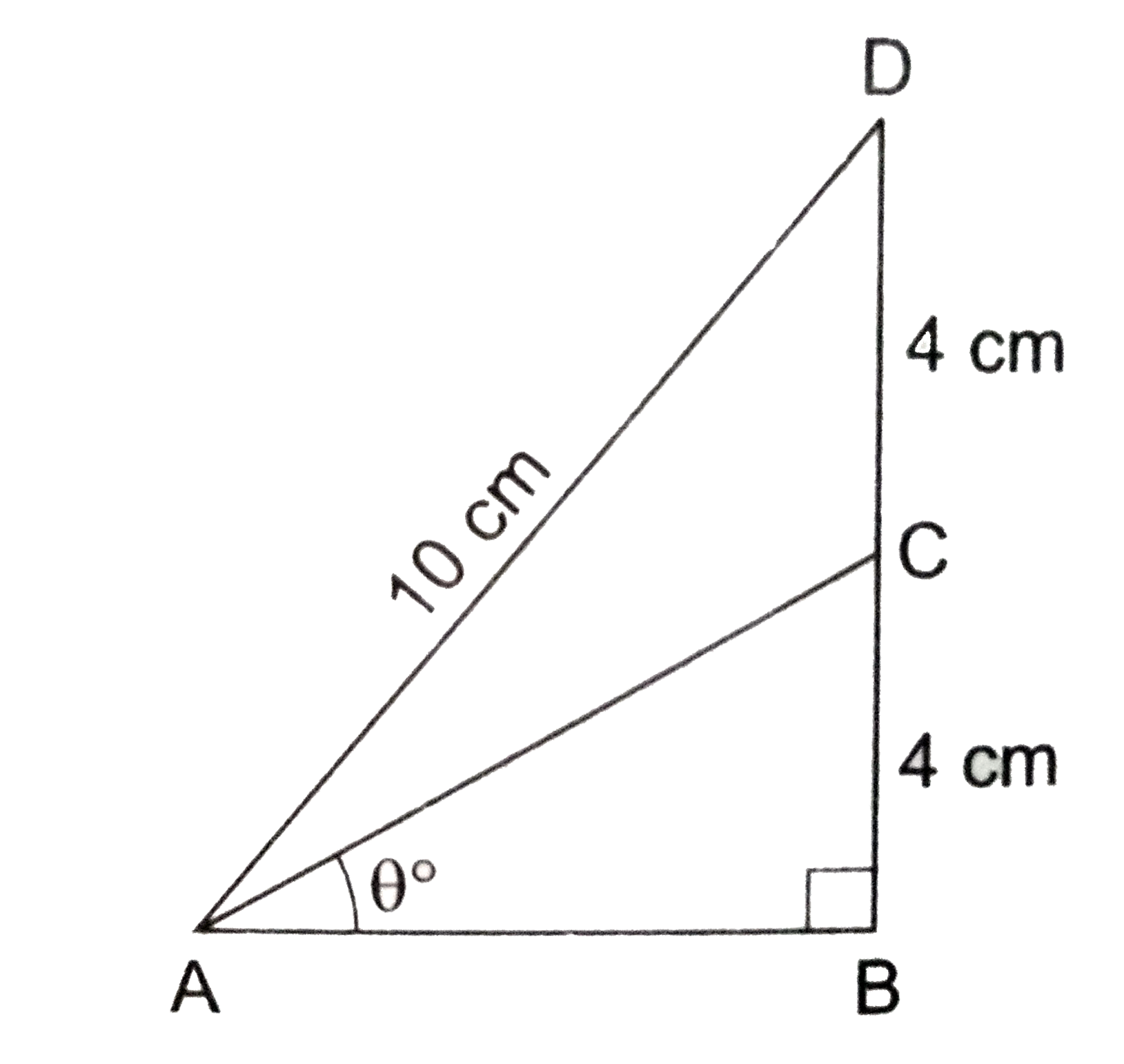
In the adjoining figure , ∠B=90∘,∠BAC=θ∘,BC=CD=4cmandAD=10cm, Find (i) sinθand(ii)cosθ.
The correct Answer is:(i)sinθ=2√313 (ii)cosθ=3√1313
AB2=(AD2−BD2)=36cm2
∴AB=6cm
AC2=(AB2+BC2)=52cm2
∴AC=2√13cm.
thus ,AB=6cmAndAC=2√13cm.
Topper's Solved these Questions
- TRIGONOMETRIC RATIOSBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC RATIOSEXERCISE - Exercise 10
35 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
- TRIGONOMETRIC RATIOS OF COMPLEMENTARY ANGLES BOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC RATIOS OF COMPLEMENTARY ANGLES EXERCISE - Exercise 12
15 Videos
Similar Questions
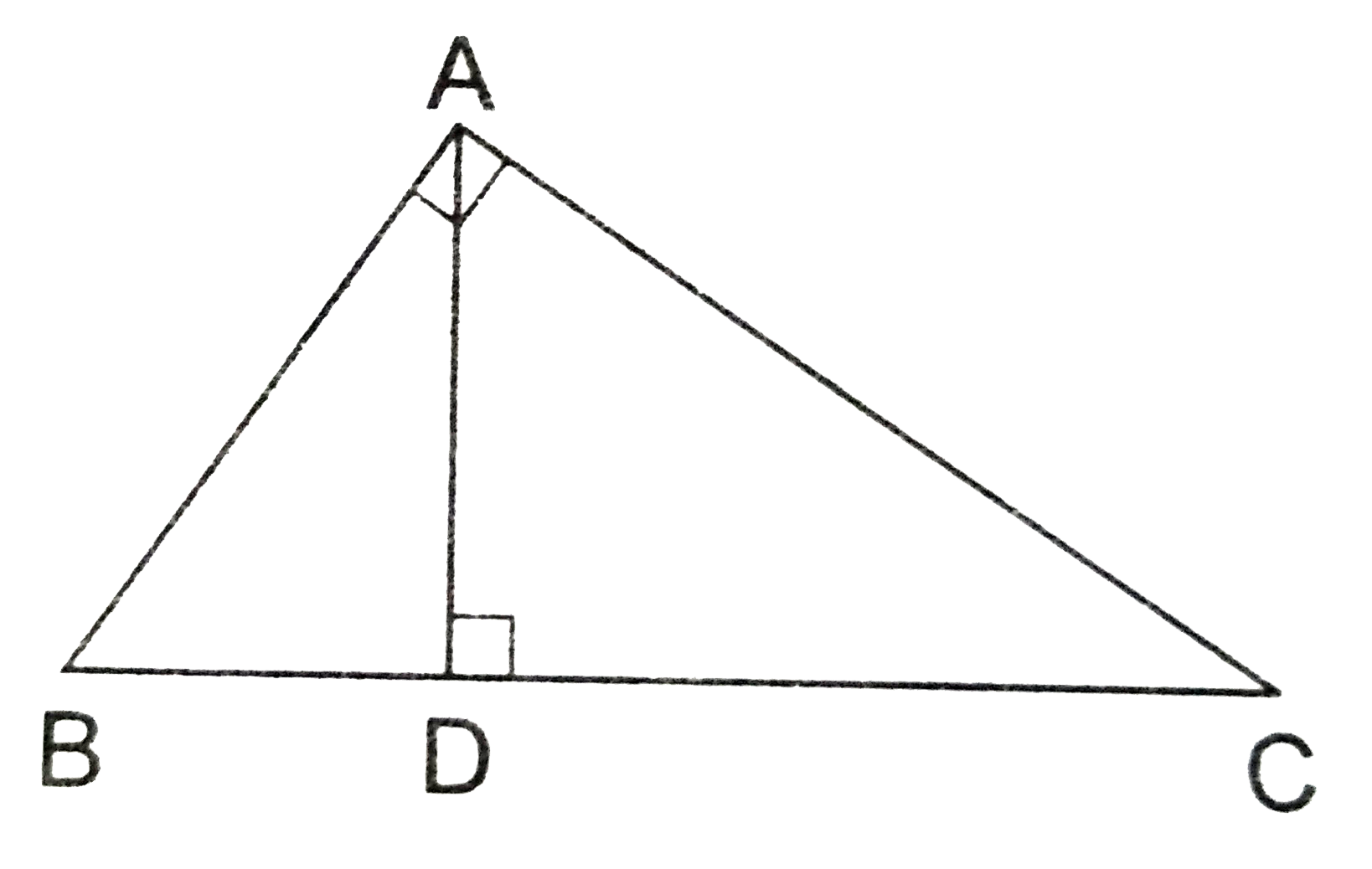
In the given figure, ∠BAC=90∘andAD⊥BC.then,
View SolutionIn the adjoining figure , AM =BM and ∠B=90∘. If ∠BCM=θ, then find the values of the following :
(i) sinθ (ii) tanθ (iii) secθ
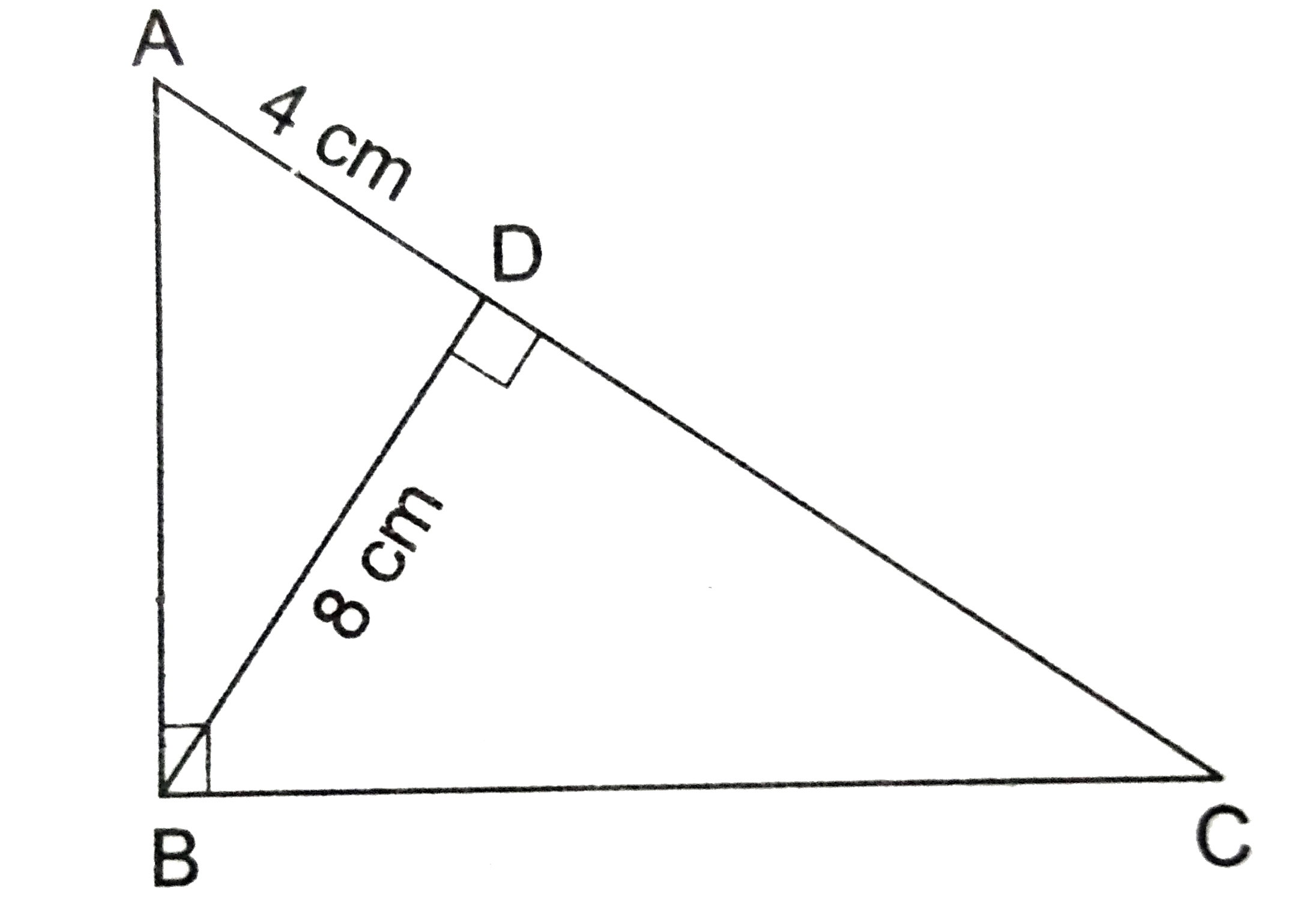
View SolutionIn the given figure, ∠ABC=90∘andBD⊥AC. If BD=8cm,AD=4cm find CD.
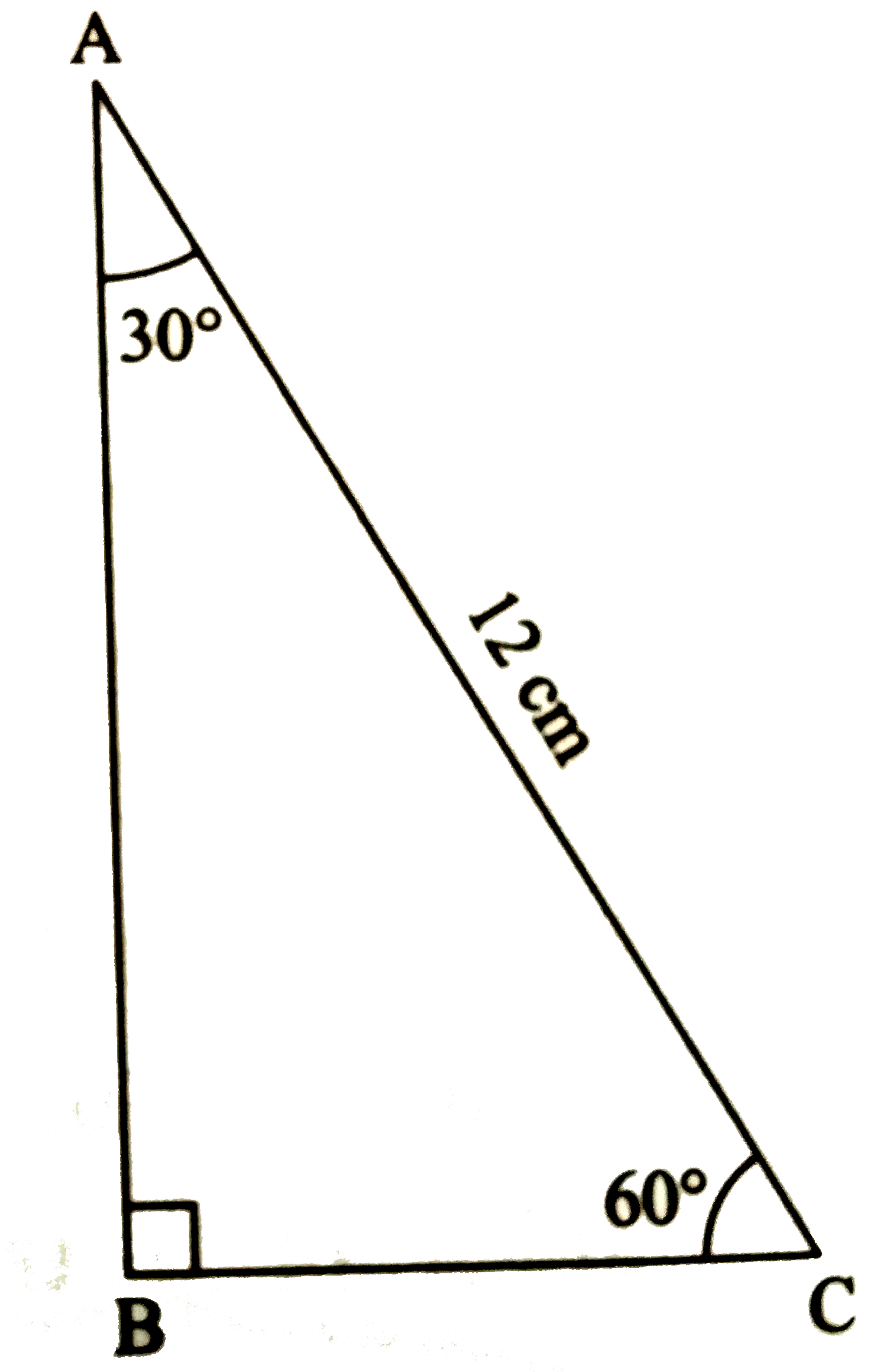
View SolutionIn the figure , AC =12 cm ,
∠ABC=90∘, ∠BAC=30∘ ,
then find AB and BC.
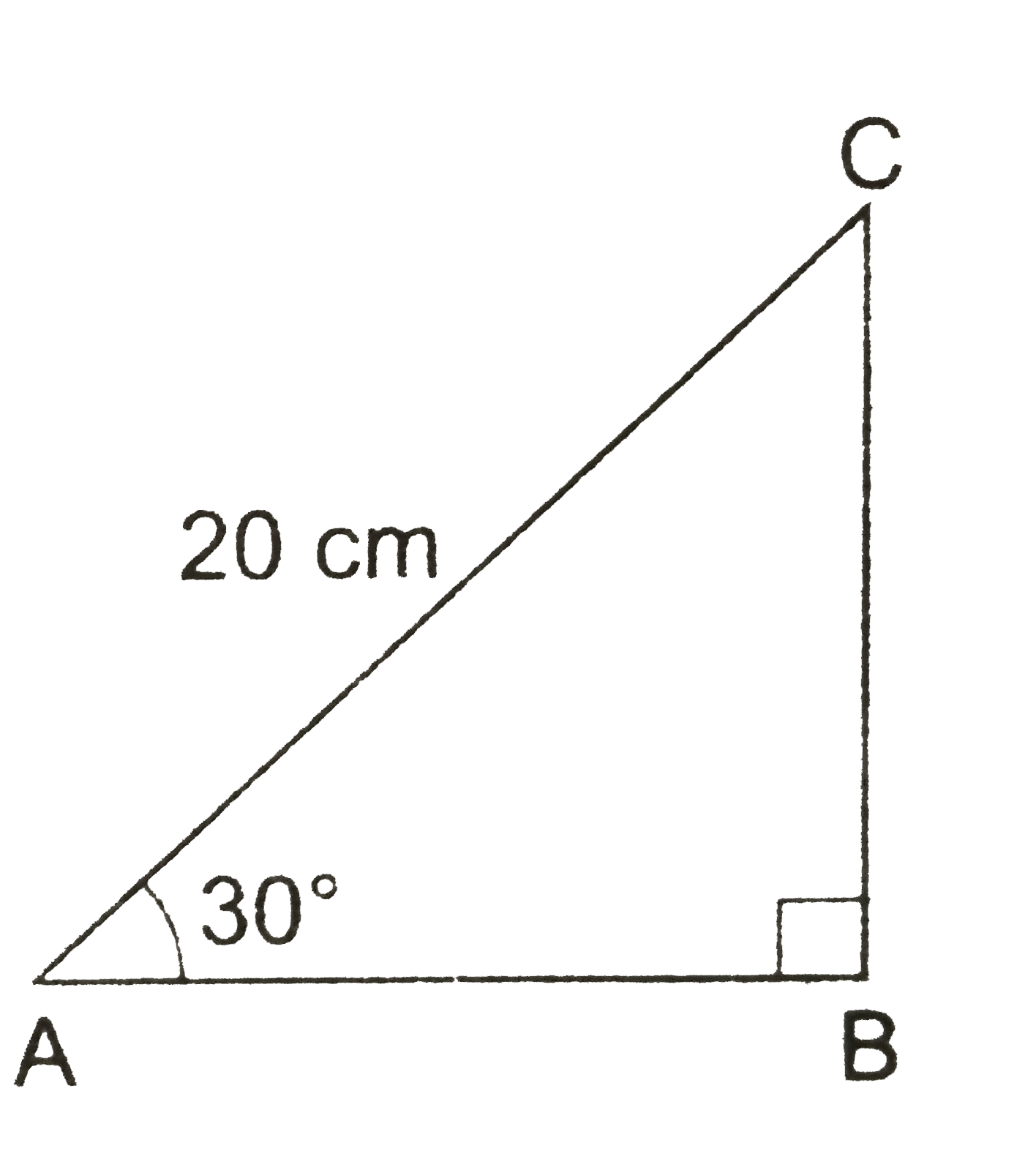
View SolutionIn the adjoining figure, ΔABC is a right-angled triangle in which ∠B=90∘, ∠A=30∘ and AC=20cm. Find (i)BC, (ii)AB.
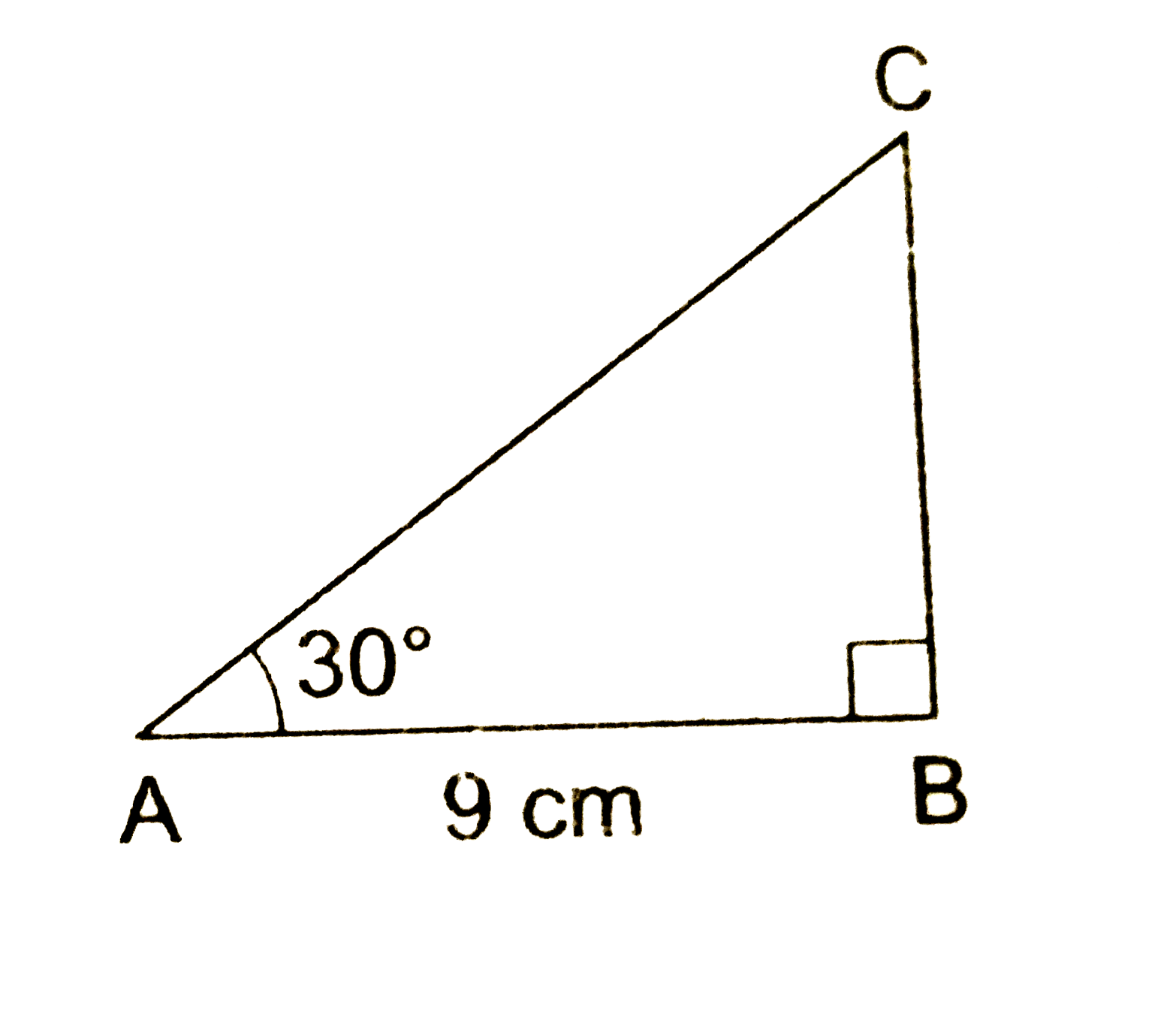
View SolutionIn the adjoining figure, ΔABC is right-angled at B. If ∠A=30∘ and AB=9cm, find (i)BC and (ii)AC.
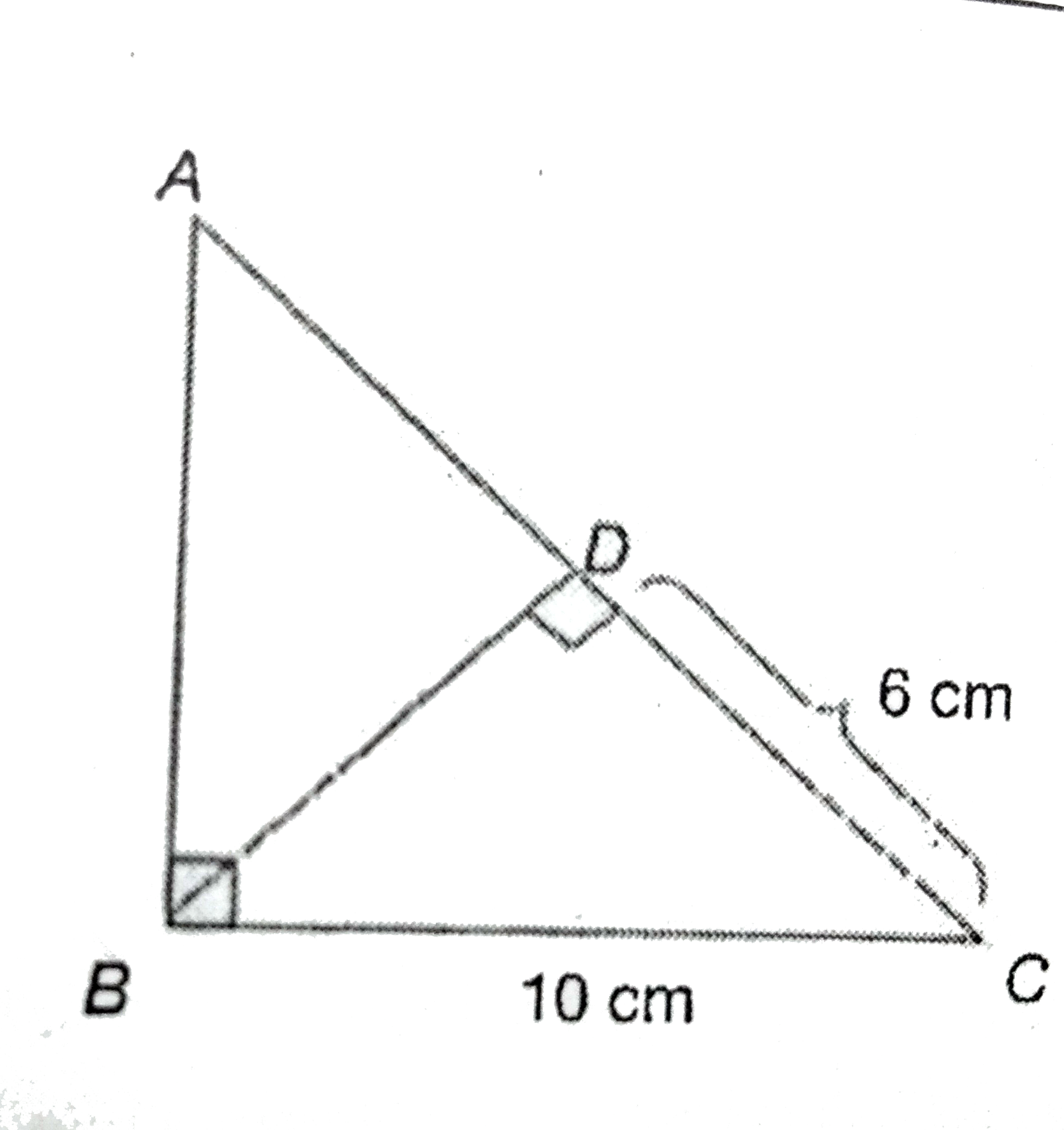
View SolutionIn the following figure, ∠ABC=90∘,BC=10cm,CD=6cm , then AD=______________.
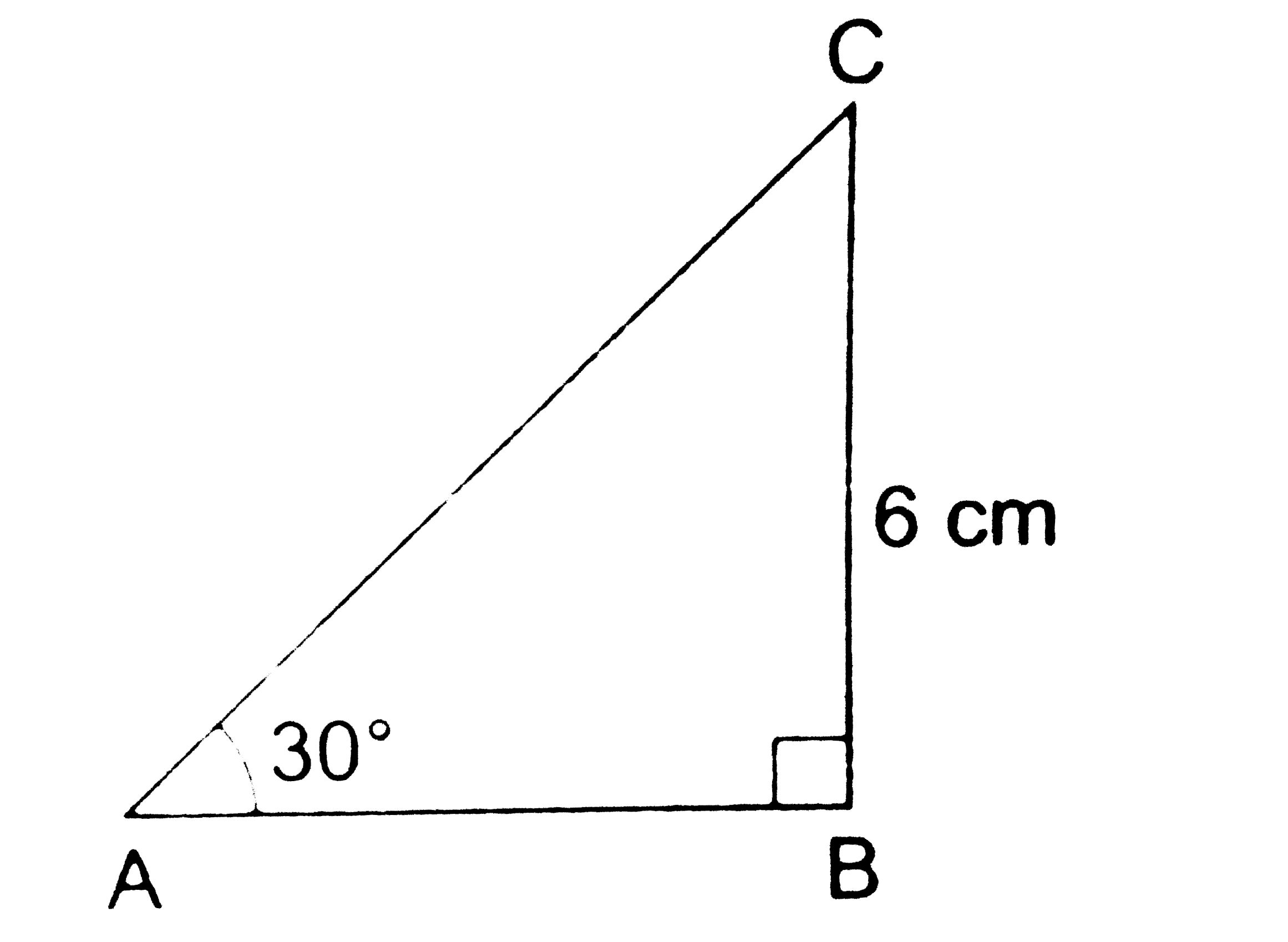
View SolutionIn the adjoining figure, ΔABC is right-angled at B and ∠A=30∘ . If BC=6cm, find (i)AB, (ii)AC.
View SolutionIn a ΔABC,∠B=90∘,AB=24cmandBC=7cm.
Find (i)sinA(ii)cosA(iii)sinC(iv)cosC.
View SolutionIn a right triangle ABC right angled at B , ∠ACB=θ , AB=2cm and BC=1 cm . Find the value of sin2θ+tan2θ .
View Solution
