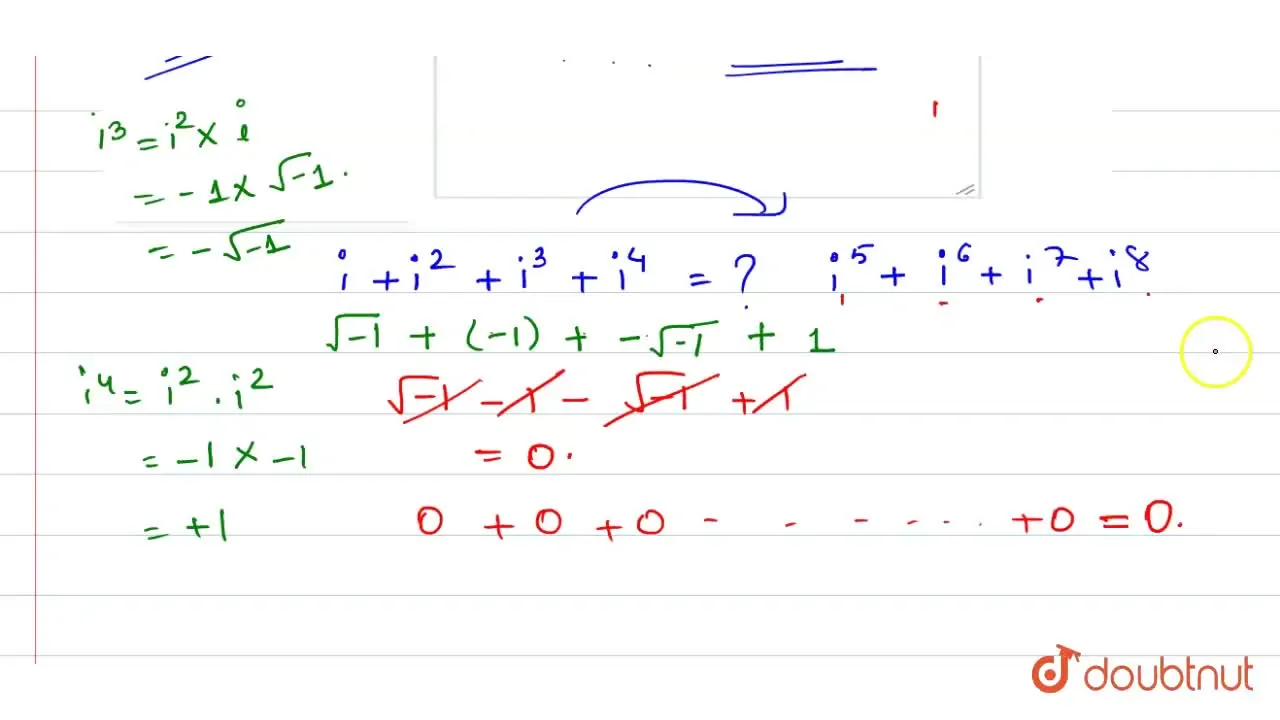
Find the sum (i+i2+i3+i4+..... up to 400 terms).
Video Solution
Text Solution
The correct Answer is:0
Given sum =(i+i2+i3+i4)+(i5+i6_i7+i8)+.......
=(i+i2+i3+1)+i5(1+i+i2+i3)+....=0. [∵(1+i+i2+i3)=0]
|
Updated on:21/07/2023
Related Playlists
- BOOK - RS AGGARWALCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - EXERCISE 5 F14videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - RS AGGARWALCHAPTER - COMBINATIONSEXERCISE - Exercise 9D18videos
COMBINATIONS
- BOOK - RS AGGARWALCHAPTER - CONDITIONAL IDENTITIES INVOLVING THE ANGLES OF A TRIANGLE EXERCISE - EXERCISE 1612videos
CONDITIONAL IDENTITIES INVOLVING THE ANGLES OF A TRIANGLE
Similar Questions
The value of i2+i4+i6+i8....upto (2n+1) terms , where i2 = -1, is equal to:
View SolutionFind the value of i+i2+i3+i4
View SolutionFind the value of i2+(−i)4−i6
View SolutionFind the value of i2+(−i)4−i6.
View SolutionThe sum of the series i+2i2+3i3+... up to 200 terms equals
View SolutionWrite the sum of the series i+i2+i3+....... upto 1000 terms.
View SolutionFind the sum of the following APs : (i) 2, 7, 12, . . . , to 10 terms.
View SolutionFind 6∑i=12.3i
View SolutionFind the value of (1+i)(1+i2)(1+i3)(1+i4)
View SolutionIf i2=−1,
then the sum i+i2+i3+... upto 1000 terms is equal to
a. 1
b. −1
c. i
d. 0View Solution