A class has 175 students, The following description gives the number of students studying one or more of the subjects in this class, Mathematics 100, physics 70, chemistry 46, Mathematics and physics 30, mathematics and chemistry 28, physics and chemistry 23, mathematics, physics and chemistry alone 18,
(i) how many students are enrolled in mathematics alone, physics alone and children alone,
(ii) the number of students who have not offered any of these subjects.
The correct Answer is:(i) 60,35,13
(ii) 32
To solve the problem, we will use the principle of inclusion-exclusion to determine the number of students studying each subject alone and those not studying any subjects.
Given Data:
- Total students (N) = 175
- Students studying Mathematics (M) = 100
- Students studying Physics (P) = 70
- Students studying Chemistry (C) = 46
- Students studying both Mathematics and Physics (M ∩ P) = 30
- Students studying both Mathematics and Chemistry (M ∩ C) = 28
- Students studying both Physics and Chemistry (P ∩ C) = 23
- Students studying all three subjects (M ∩ P ∩ C) = 18
Step 1: Calculate Students Enrolled in Each Subject Alone
(i) Students Enrolled in Mathematics Alone
To find the number of students studying Mathematics alone (denoted as M_only), we use the formula:
Monly=M−(M∩P)−(M∩C)+(M∩P∩C)
Substituting the values:
Monly=100−30−28+18
Monly=100−58+18
Monly=60
(ii) Students Enrolled in Physics Alone
To find the number of students studying Physics alone (denoted as P_only), we use the formula:
Ponly=P−(M∩P)−(P∩C)+(M∩P∩C)
Substituting the values:
Ponly=70−30−23+18
Ponly=70−53+18
Ponly=35
(iii) Students Enrolled in Chemistry Alone
To find the number of students studying Chemistry alone (denoted as C_only), we use the formula:
Conly=C−(M∩C)−(P∩C)+(M∩P∩C)
Substituting the values:
Conly=46−28−23+18
Conly=46−51+18
Conly=13
Summary of Students Enrolled Alone
- Students studying Mathematics alone = 60
- Students studying Physics alone = 35
- Students studying Chemistry alone = 13
Step 2: Calculate the Number of Students Not Enrolled in Any Subject
To find the number of students who have not offered any of these subjects, we first need to calculate the total number of students studying at least one subject using the principle of inclusion-exclusion:
NM∪P∪C=M+P+C−(M∩P)−(M∩C)−(P∩C)+(M∩P∩C)
Substituting the values:
NM∪P∪C=100+70+46−30−28−23+18
NM∪P∪C=216−81+18
NM∪P∪C=153
Now, to find the number of students who have not offered any subjects:
Nnot_offered=N−NM∪P∪C
Substituting the values:
Nnot_offered=175−153
Nnot_offered=22
Final Answers
1. Students enrolled in Mathematics alone = 60
2. Students enrolled in Physics alone = 35
3. Students enrolled in Chemistry alone = 13
4. Students who have not offered any of these subjects = 22
- Total students (N) = 175
- Students studying Mathematics (M) = 100
- Students studying Physics (P) = 70
- Students studying Chemistry (C) = 46
- Students studying both Mathematics and Physics (M ∩ P) = 30
- Students studying both Mathematics and Chemistry (M ∩ C) = 28
- Students studying both Physics and Chemistry (P ∩ C) = 23
- Students studying all three subjects (M ∩ P ∩ C) = 18
To find the number of students studying Mathematics alone (denoted as M_only), we use the formula:
Monly=M−(M∩P)−(M∩C)+(M∩P∩C)
Substituting the values:
Monly=100−30−28+18
Monly=100−58+18
Monly=60
To find the number of students studying Physics alone (denoted as P_only), we use the formula:
Ponly=P−(M∩P)−(P∩C)+(M∩P∩C)
Substituting the values:
Ponly=70−30−23+18
Ponly=70−53+18
Ponly=35
To find the number of students studying Chemistry alone (denoted as C_only), we use the formula:
Conly=C−(M∩C)−(P∩C)+(M∩P∩C)
Substituting the values:
Conly=46−28−23+18
Conly=46−51+18
Conly=13
- Students studying Mathematics alone = 60
- Students studying Physics alone = 35
- Students studying Chemistry alone = 13
To find the number of students who have not offered any of these subjects, we first need to calculate the total number of students studying at least one subject using the principle of inclusion-exclusion:
NM∪P∪C=M+P+C−(M∩P)−(M∩C)−(P∩C)+(M∩P∩C)
Substituting the values:
NM∪P∪C=100+70+46−30−28−23+18
NM∪P∪C=216−81+18
NM∪P∪C=153
Nnot_offered=N−NM∪P∪C
Substituting the values:
Nnot_offered=175−153
Nnot_offered=22
1. Students enrolled in Mathematics alone = 60
2. Students enrolled in Physics alone = 35
3. Students enrolled in Chemistry alone = 13
4. Students who have not offered any of these subjects = 22
Topper's Solved these Questions
- SETSBOOK - RS AGGARWALCHAPTER - SETSEXERCISE - EXERCISE-1H
15 Videos
- SETSBOOK - RS AGGARWALCHAPTER - SETSEXERCISE - EXERCISE-1F
6 Videos
- RelationsBOOK - RS AGGARWALCHAPTER - RelationsEXERCISE - EXERCISE 2E
12 Videos
- SOLUTION OF TRIANGLESBOOK - RS AGGARWALCHAPTER - SOLUTION OF TRIANGLESEXERCISE - EXERCISE 18 B
3 Videos
Similar Questions
A class has 175 students. The following data shows the number of students obtaining one or more subjects. Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18. How many students have offered Mathematics alone (a) 35 (c) 60 (b) 48 (d) 22
View SolutionA class has 175 students. The following data shows the number of students obtaining one or more subjects. Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18. How many students have offered Mathematics alone (a) 35 (c) 60 (b) 48 (d) 22
View Solution
Knowledge Check
In the class of 175 students, the following data shows the number of students opting one or more subjects.
Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18.
Than, number of students opted Mathematics alone isA35B48C60D20A class has 172 students. The following data shows the number of students opting one or more subjects: Mathematics 125, Physics 95, Chemistry 65 Mathematics and Physics 55
Mathematics and Chemistry 53
Physics and Chemistry 48 Mathematics, Physics and Chemistry 43
The number of students who opted only Physics isA35B45C29D37In a class of 175 students the following data shows the number of students opting one or more subjects Mathematics 100, physics 70, chemistry 28, physics and Chemistry 23, mathematics physics and chemistry 18. how many students have offered mathematics alone ?
A35B48C60D22
A class has 175 students. The following data shows the number of students obtaining one or more subjects. Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18. How many students have offered Mathematics alone (a) 35 (c) 60 (b) 48 (d) 22
View SolutionA class has 175 students. The following data shows the number of students obtaining one or more subjects : Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics & Physics & Chemistry 18. How many students have offered Mathematics alone ?
View SolutionA class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
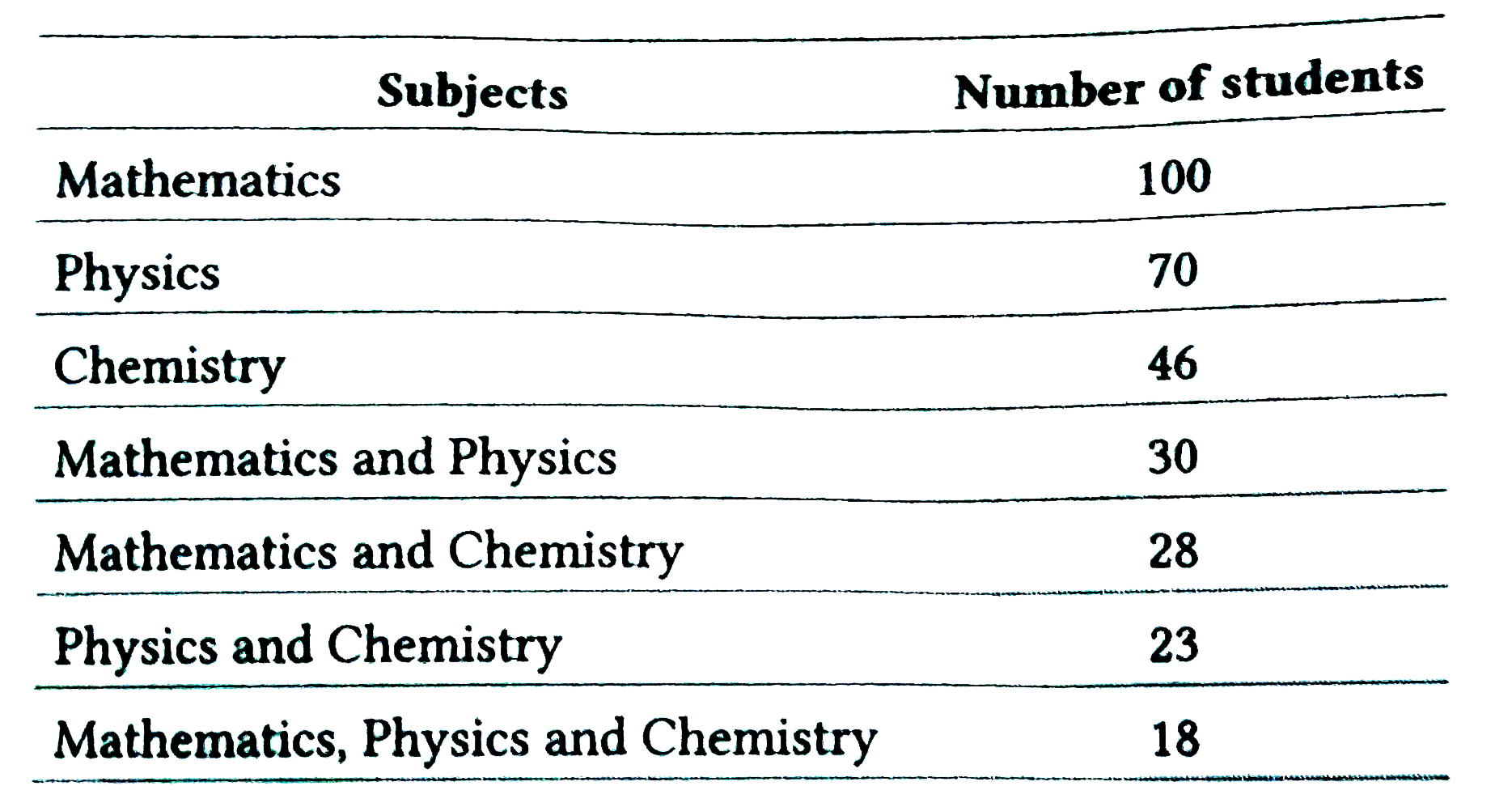
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View SolutionIn a class of 55 students the number of students studying subjects are , 23 iin Mathematics 24.in physics 19in chemisty 12 in Mathematics and physics ,9 in Mathematics and chemistry ,7 in physics and chemistry and 4 in all the three subjects.
the number of students who have taken ecactly one subject is
View SolutionIn a class of 75 students, the number of students studying different Subjects are 43 in Mathematics 44 in Physics, 39 in Chemistry, 32 in Mathematics and Physics, 29 in Mathematics and Chemistry 27 in Physics and Chemistry and 24 in all three subjects. The number of students who have taken exactly one subject is
View Solution
