function is
One One
Many One
Not a function
None of these
The correct Answer is:A
To determine whether the function f:R→R defined by f(x)=x3 is one-to-one and onto, we will follow these steps:
Step 1: Show that the function is one-to-one (injective)
A function f is one-to-one if for every a,b∈R, f(a)=f(b) implies a=b.
1. Assume f(a)=f(b).
a3=b3
2. Taking the cube root of both sides, we have:
a=b
3. Since a=b whenever f(a)=f(b), the function f(x)=x3 is one-to-one.
Step 2: Show that the function is onto (surjective)
A function f is onto if for every y∈R, there exists an x∈R such that f(x)=y.
1. Let y be any real number. We need to find x such that:
f(x)=y⟹x3=y
2. Solving for x, we find:
x=y1/3
3. Since y can be any real number, y1/3 is also a real number. Therefore, for every y∈R, there exists an x∈R such that f(x)=y.
Conclusion
Since f(x)=x3 is both one-to-one and onto, we conclude that the function f:R→R defined by f(x)=x3 is a bijection.
---
a3=b3
2. Taking the cube root of both sides, we have:
a=b
3. Since a=b whenever f(a)=f(b), the function f(x)=x3 is one-to-one.
f(x)=y⟹x3=y
2. Solving for x, we find:
x=y1/3
3. Since y can be any real number, y1/3 is also a real number. Therefore, for every y∈R, there exists an x∈R such that f(x)=y.
Topper's Solved these Questions
- FUNCTIONSBOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - Objective Questions
39 Videos
- FUNCTIONSBOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - Exercise 2A
17 Videos
- DIFFERENTIATIONBOOK - RS AGGARWALCHAPTER - DIFFERENTIATIONEXERCISE - Exercise 10I
51 Videos
- FUNDAMENTAL CONCEPTS OF 3-DIMENSIONAL GEOMETRYBOOK - RS AGGARWALCHAPTER - FUNDAMENTAL CONCEPTS OF 3-DIMENSIONAL GEOMETRYEXERCISE - Exercise
18 Videos
Similar Questions
Knowledge Check
The function f:R→R,f(x)=x2 is
Ainjective but not surjectiveBsurjective but not injectiveCinjective as well as surjectiveDneither injective nor surjective.The function f:R→R given by f(x)=x2+x is
Aone-one nad ontoBone-one and intoCmany-one and ontoDmany one and intoStatement-1: The function f:R→R defined by f(x)=x3+4x−5 is a bijection.
Statement-2: Every odd degree has at least one real root.A1B2C3D4
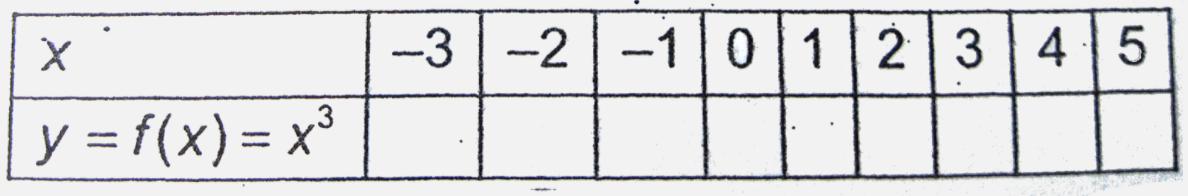
Define the function f:R→R by y=f(x)=x3,x∈R. Complete the table given below by using this definition. What is the domain and range of this function ? Draw the graph of f.
View SolutionThe function f:R→R defined by f(x) = 4x + 7 is
View SolutionIf g(y) is inverse of function f:R→R given by f(x)=x+3, then g(y)=
View Solutionif the function f:R→R given be f(x)=x3+ax2+5x+sin2x is invertible then
View SolutionSet of values of 'a' for which the function f:R→R, given by f(x)=x3+(a+2)x2+3ax+10 is one-one is given by :
View Solution
