A 60 Hz AC voltage of 160 V impressed across an LR-circuit results in a current of 2A. If the power dissipation is 200 W, calculate the maximum value of the back emf (in volt) arising in the inductance.
To solve the problem step by step, we will follow the process outlined in the video transcript.
Step 1: Identify Given Values
- Frequency (f) = 60 Hz
- RMS Voltage (V_RMS) = 160 V
- RMS Current (I_RMS) = 2 A
- Power Dissipation (P) = 200 W
Step 2: Calculate Impedance (Z)
Using the formula for impedance in an AC circuit:
Z=VRMSIRMS
Substituting the known values:
Z=160V2A=80Ω
Step 3: Calculate Power Factor (cos φ)
The power in an AC circuit is given by:
P=VRMS⋅IRMS⋅cosϕ
Rearranging for the power factor:
cosϕ=PVRMS⋅IRMS
Substituting the known values:
cosϕ=200W160V⋅2A=200320=0.625
Step 4: Calculate Resistance (R)
Using the relationship between impedance, resistance, and inductive reactance:
Z=√R2+X2L
We can also express the power factor as:
cosϕ=RZ
Thus, we can find R:
R=Z⋅cosϕ=80Ω⋅0.625=50Ω
Step 5: Calculate Inductive Reactance (X_L)
Using the impedance formula:
Z2=R2+X2L
Substituting the known values:
802=502+X2L
Calculating:
6400=2500+X2L
X2L=6400−2500=3900
XL=√3900≈62.45Ω(rounded to 62.5Ω)
Step 6: Calculate Maximum Value of Back EMF (V_L)
The voltage across the inductance is given by:
VL=IRMS⋅XL
Substituting the known values:
VL=2A⋅62.5Ω=125V
Final Answer
The maximum value of the back EMF arising in the inductance is 125 V.
---
- Frequency (f) = 60 Hz
- RMS Voltage (V_RMS) = 160 V
- RMS Current (I_RMS) = 2 A
- Power Dissipation (P) = 200 W
Using the formula for impedance in an AC circuit:
Z=VRMSIRMS
Substituting the known values:
Z=160V2A=80Ω
The power in an AC circuit is given by:
P=VRMS⋅IRMS⋅cosϕ
Rearranging for the power factor:
cosϕ=PVRMS⋅IRMS
Substituting the known values:
cosϕ=200W160V⋅2A=200320=0.625
Using the relationship between impedance, resistance, and inductive reactance:
Z=√R2+X2L
We can also express the power factor as:
cosϕ=RZ
Thus, we can find R:
R=Z⋅cosϕ=80Ω⋅0.625=50Ω
Using the impedance formula:
Z2=R2+X2L
Substituting the known values:
802=502+X2L
Calculating:
6400=2500+X2L
X2L=6400−2500=3900
XL=√3900≈62.45Ω(rounded to 62.5Ω)
The voltage across the inductance is given by:
VL=IRMS⋅XL
Substituting the known values:
VL=2A⋅62.5Ω=125V
The maximum value of the back EMF arising in the inductance is 125 V.
Topper's Solved these Questions
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 22 : (ELECTROMAGNETIC WAVES)
15 Videos
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 23 : (RAY OPTICS AND OPTICAL INSTRUMENTS)
15 Videos
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 20 : (ELECTROMAGNETIC INDUCTION)
15 Videos
- ATOMSBOOK - DISHA PUBLICATIONCHAPTER - ATOMSEXERCISE - EXERCISE-2: CONCEPT APPLICATOR
30 Videos
- COMMUNICATION SYSTEM BOOK - DISHA PUBLICATIONCHAPTER - COMMUNICATION SYSTEM EXERCISE - EXERCISE-2 : Concept Applicator
30 Videos
Similar Questions
An inductor of inductance 5 H is connected across an alternating power supply of 220 volts . The frequency of source is 50 Hz. Calculate the maximum value of curent in the inductor .
View SolutionA coil is series with a 20μF capacitor across a 230V,50Hz supply. The current taken by the circuit is 8A and the power consumed is 200W. Calculate the inductance of the coil if the current in the circuit is
(a) leading
(b) lagging
View Solution
Knowledge Check
In an AC circuit with voltage V and current I, the power dissipated is
AVIB12VIC1√2VIDDepened on the phase between V and IIn an a.c. circuit with voltage V and current I, the power dissipated is
AVIB12VIC1√2VIDdepends on the phase between V and I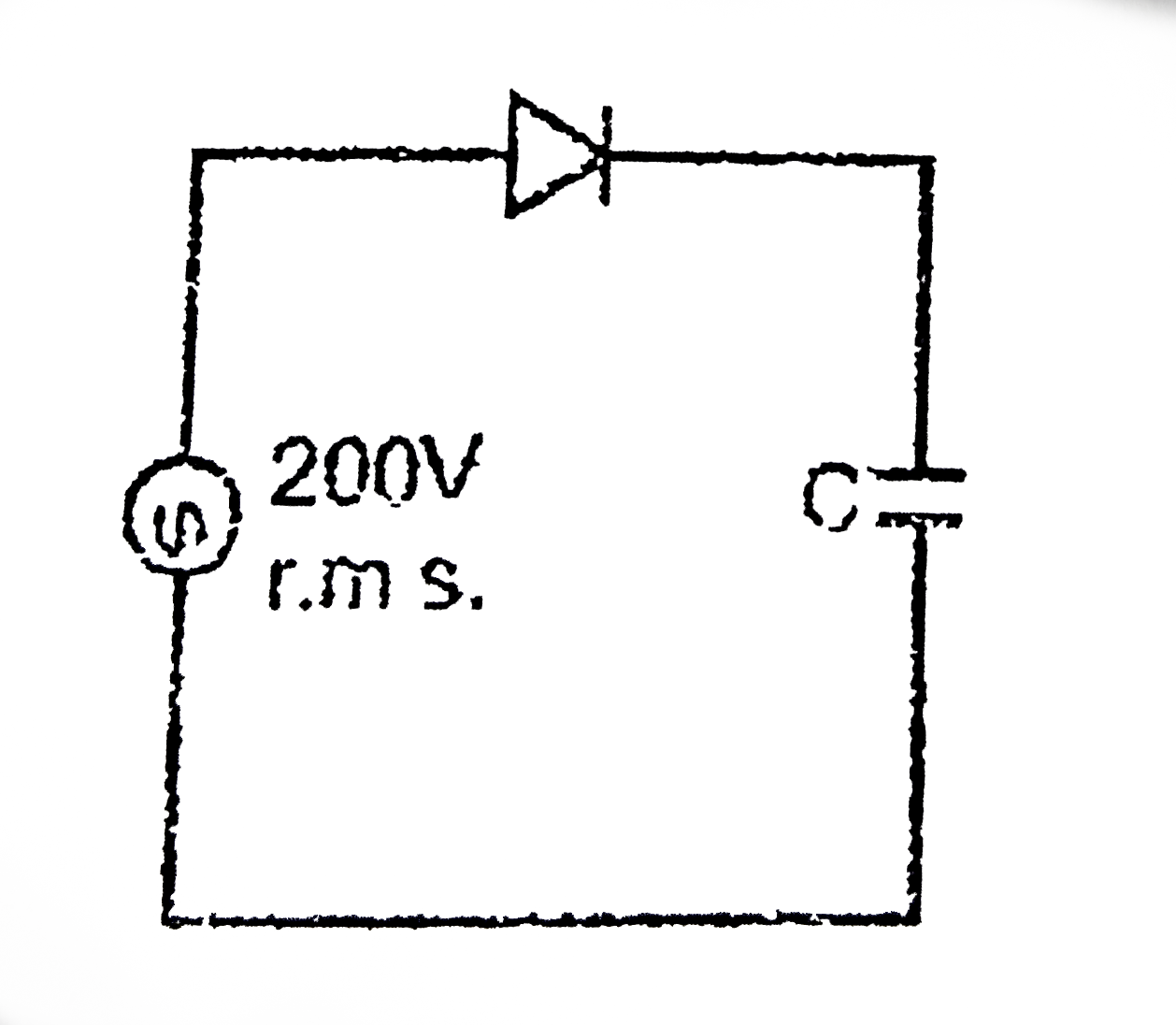
In the figure an A.C of rms voltage 200 volt is appled to the circuit containing diode and the capacitor and it is being rectified. The maximum potential across the capacitor C in volt will beA500B200C283D141
An a.c. voltage of 100 V, 50 Hz is connected across a 20 Ω resistance and a 2 mH inductor in series. Calculate (1) impedance of the circuit, (ii) rms current in the circuit.
View SolutionA 2 uF capacitor, 100 Ω resistor and 8 H inductor are connected in series with an a.c. source. Find the frequency of the a.c. source for which the current drawn in the circuit is maximum. If the peak value of emf of the source is 200 V, calculate the
(i) maximum current, and (ii) inductive and capacitive reactance of the circuit at resonance.
View SolutionA 2 uF capacitor, 100 Ω resistor and 8 Hinductor are connected in series with an a.c. source. Find the frequency of the a.c. source for which the current drawn in the circuit is maximum. If the peak value of emf of the source is 200 V, calculate the (i) maximum current, and (ii) inductive and capacitive reactance of the circuit at resonance.
View SolutionA resistor and an inductor are connected in series across an AC power supply . The value of current drawn from the source is 2 A . Calculate the net impedance of circuit if voltage across inductor and resistor are 100 V and 80 V respectively.
View Solution(a) Prove that the current flowing through an ideal inductor connected across a.c. source, lags the voltage in phase by π2.
(b) An inductor of self inductance 100 mH, and a bulb are connected in series with a.c. sources of rms voltage 10 V, 50 z. It is found that effective voltage of the circuit leads the current in phase by π4.
Calculate the inductance of the inductor used and average power dissipated in the circuit, if a current of 1 A flows in the circuit.
View Solution
