A, B and C are the three points on a circle such that the angle subtendsed by the chords AB and AC at the centre O are 90∘ and 110∘ respectively. ∠BAC is equal to
70∘
80∘
90∘
100∘
The correct Answer is:B
To solve the problem, we need to find the angle ∠BAC given the angles subtended by the chords AB and AC at the center O of the circle.
1. Understand the Given Angles:
- The angle subtended by chord AB at the center O is 90∘.
- The angle subtended by chord AC at the center O is 110∘.
2. Identify the Angles at the Center:
- Let ∠AOB=90∘ (angle at center for chord AB).
- Let ∠AOC=110∘ (angle at center for chord AC).
3. Use the Properties of Angles in a Circle:
- The angle subtended at the circumference by a chord is half the angle subtended at the center by the same chord. Therefore:
- ∠ACB=12∠AOB=12×90∘=45∘
- ∠ABC=12∠AOC=12×110∘=55∘
4. Apply the Triangle Angle Sum Property:
- In triangle ABC, the sum of angles is 180∘:
∠A+∠B+∠C=180∘
- Substituting the known angles:
∠BAC+∠ABC+∠ACB=180∘
∠BAC+55∘+45∘=180∘
5. Calculate ∠BAC:
- Combine the known angles:
∠BAC+100∘=180∘
- Solve for ∠BAC:
∠BAC=180∘−100∘=80∘
Conclusion:
The angle ∠BAC is 80∘.
- The angle subtended by chord AB at the center O is 90∘.
- The angle subtended by chord AC at the center O is 110∘.
- Let ∠AOB=90∘ (angle at center for chord AB).
- Let ∠AOC=110∘ (angle at center for chord AC).
- The angle subtended at the circumference by a chord is half the angle subtended at the center by the same chord. Therefore:
- ∠ACB=12∠AOB=12×90∘=45∘
- ∠ABC=12∠AOC=12×110∘=55∘
- In triangle ABC, the sum of angles is 180∘:
∠A+∠B+∠C=180∘
- Substituting the known angles:
∠BAC+∠ABC+∠ACB=180∘
∠BAC+55∘+45∘=180∘
- Combine the known angles:
∠BAC+100∘=180∘
- Solve for ∠BAC:
∠BAC=180∘−100∘=80∘
The angle ∠BAC is 80∘.
Similar Questions
A, B and C are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 80∘ and 120∘, respectively. The value of ∠BAC is:
A, B तथा C एक वृत्त पर स्थित तीन ऐसे बिंदु हैं कि जीवाओं AB तथा AC के द्वारा केंद्र पर अंतरित कोण क्रमशः 80∘ तथा 120∘ हैं | कोण BAC का मान क्या है ?
View SolutionA,BandC are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 90∘a n d 110∘, respectively. Determine ∠BAC
View Solution
Knowledge Check
A, B and C are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 80∘ and 120∘, respectively. The value of ∠BAC is:
A, B तथा C एक वृत्त पर स्थित तीन ऐसे बिंदु हैं कि जीवाओं AB तथा AC के द्वारा केंद्र पर अंतरित कोण क्रमशः 80∘ तथा 120∘ हैं | कोण BAC का मान क्या है ?A70∘B80∘C90∘D100∘A, B and C are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 80∘ and 120∘, respectively. The value of ∠BAC is:
A, B तथा C एक वृत्त पर स्थित तीन ऐसे बिंदु हैं कि जीवाओं AB तथा AC के द्वारा केंद्र पर अंतरित कोण क्रमशः 80∘ तथा 120∘ हैं | कोण BAC का मान क्या है ?A85∘B75∘C80∘D70∘A, B and C are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 110°∘and 130∘ respectively. Then the value of ∠BAC is :
A75∘B70∘C60∘D65∘
A,B and C are three points on a circle such that the angles subtended by the chord AB and AC at the centre O are 110∘ and 130∘ respectively. Then the value of ∠BAC is:
A, B और C एक वृत्त पर स्थित तीन ऐसे बिंदु हैं कि जीवा AB और AC के द्वारा केंद्र O पर अंतरित किये गए कोण क्रमशः 110∘ तथा 130∘ हैं| ∠BAC का मान है :
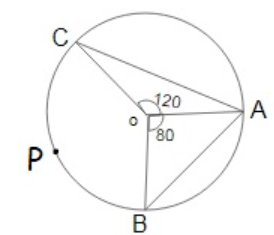
View SolutionIn Figure, A,B,C are three points on a circle such that the angles sub center O are 80∘and120∘ respectively. Determine ∠BAC and the degree measure of arc BPC
View SolutionA, B and C are three points on a circle such that the angles subtended by the chord AB and AC at the centre O are 110° and 130°, respectively. The value of ∠BAC is:
View SolutionA,B and C are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 90∘ and 110∘ respectively . Further suppose that the centre O lies on the interior ∠BAC. The ∠BAC is
View SolutionA,B and C are three points on a circle such that the angles subtended by the chords AB and AC at the centre O are 90∘ and 110∘ respectively . Further suppose that the centre O lies on the interior ∠BAC. The ∠BAC is
View Solution
