A stone is dropped form 100 m high cliff. Another stone is thrown upward from ground with a velocity 10m/s. At what time both the stones will meet each other ?
To solve the problem of when the two stones meet, we can break it down into a series of steps using the equations of motion.
1. Identify the Variables:
- Let the height of the cliff be h=100m.
- The initial velocity of the stone dropped from the cliff u1=0m/s.
- The initial velocity of the stone thrown upward u2=10m/s.
- The acceleration due to gravity g=9.81m/s2 (we can approximate this as 10m/s2 for simplicity).
2. Displacement of the First Stone (Dropped Stone):
- The displacement S1 of the stone dropped from the cliff after time t can be calculated using the equation of motion:
S1=u1t+12gt2
- Since u1=0:
S1=12gt2=12×10×t2=5t2
3. Displacement of the Second Stone (Thrown Upward):
- The displacement S2 of the stone thrown upward after time t is given by:
S2=u2t−12gt2
- Substituting u2=10:
S2=10t−12×10×t2=10t−5t2
4. Setting Up the Equation:
- The two stones will meet when the sum of their displacements equals the height of the cliff:
S1+S2=100
- Substituting the expressions for S1 and S2:
5t2+(10t−5t2)=100
- Simplifying this:
10t−5t2=100
5t2−10t+100=0
5. Rearranging the Equation:
- Rearranging gives us:
5t2−10t−100=0
- Dividing through by 5:
t2−2t−20=0
6. Solving the Quadratic Equation:
- We can use the quadratic formula t=−b±√b2−4ac2a:
- Here, a=1,b=−2,c=−20.
t=−(−2)±√(−2)2−4⋅1⋅(−20)2⋅1
t=2±√4+802
t=2±√842
t=2±2√212
t=1±√21
7. Finding the Positive Time:
- Since time cannot be negative, we take:
t=1+√21≈5.58s
Conclusion:
The two stones will meet each other approximately at t≈5.58s.
- Let the height of the cliff be h=100m.
- The initial velocity of the stone dropped from the cliff u1=0m/s.
- The initial velocity of the stone thrown upward u2=10m/s.
- The acceleration due to gravity g=9.81m/s2 (we can approximate this as 10m/s2 for simplicity).
- The displacement S1 of the stone dropped from the cliff after time t can be calculated using the equation of motion:
S1=u1t+12gt2
- Since u1=0:
S1=12gt2=12×10×t2=5t2
- The displacement S2 of the stone thrown upward after time t is given by:
S2=u2t−12gt2
- Substituting u2=10:
S2=10t−12×10×t2=10t−5t2
- The two stones will meet when the sum of their displacements equals the height of the cliff:
S1+S2=100
- Substituting the expressions for S1 and S2:
5t2+(10t−5t2)=100
- Simplifying this:
10t−5t2=100
5t2−10t+100=0
- Rearranging gives us:
5t2−10t−100=0
- Dividing through by 5:
t2−2t−20=0
- We can use the quadratic formula t=−b±√b2−4ac2a:
- Here, a=1,b=−2,c=−20.
t=−(−2)±√(−2)2−4⋅1⋅(−20)2⋅1
t=2±√4+802
t=2±√842
t=2±2√212
t=1±√21
- Since time cannot be negative, we take:
t=1+√21≈5.58s
The two stones will meet each other approximately at t≈5.58s.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE (INTEGER TYPE QUESTIONS )
9 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)
12 Videos
Similar Questions
A stone is dropped from a height h simultaneously another stone is thrown up from the ground with such a velocity so that it can reach a height of 4h. The time when two stones cross each other is √(hkg) where k=_____
View SolutionA stone is dropped from a height h. Simultaneously another stone is thrown up from the ground with such a velocity that it can reach a height of 4 h. Find the time when two stones cross each other.
View Solution
Knowledge Check
A stone is dropped from a height h. simultaneously, another stone is thrown up from the ground which reaches a height 4h. The two stones will cross each other after time:-
A√h8gB√8ghC√2ghD√h2gA stone is dropped from a height h. Simultaneously, another stone is thrown up from the ground which reaches a height 4 h. The two stones cross each other after time
A→v/2andv/2B0 and v / 2C0 and 0D→v/2and0A stone is dropped from the top of a 400m high tower. At the same time another stone is projected vertically upwards from the ground with a speed of 50m/s. The two stones will cross each other after a time
A2 sB4 sC6 sD8 s
A stone is dropped from the top of a tower 200 m high. At the same time, another stone is projected vertically upwards from the ground with a velocity of 40m/s. Calcuate when and where the two stones will mest.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stone will meet.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25ms.Calculate when and where the two stones will meet.
View Solution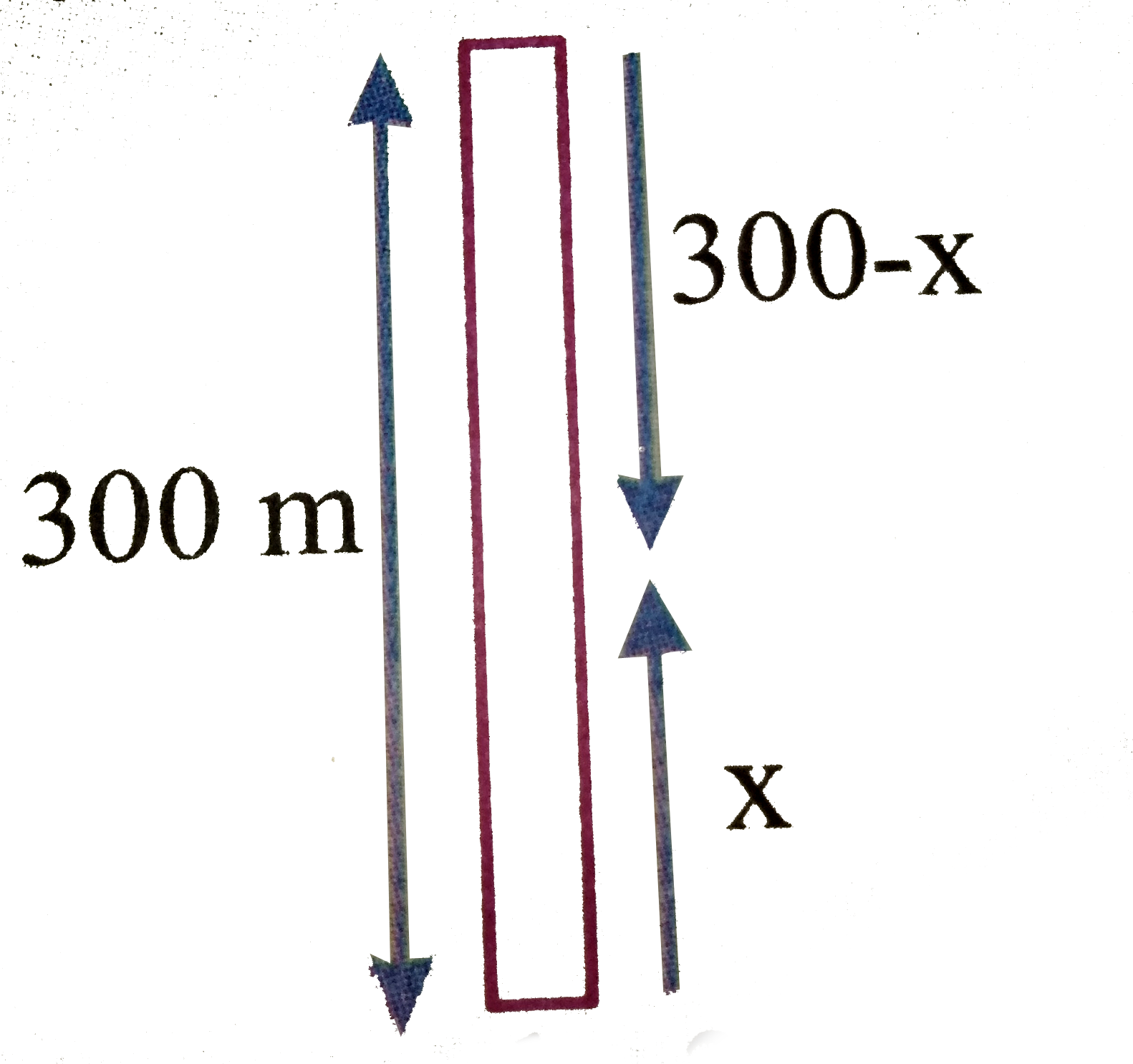
A stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100m/s. Find when and where the two stones meet?
View SolutionA stone is dropped from the top of a tall cliff and n seconds later another stone is thrown vertically downwards with a velocity u. Then the second stone overtakes the first, below the top of the cliff at a distance given by
View Solution
