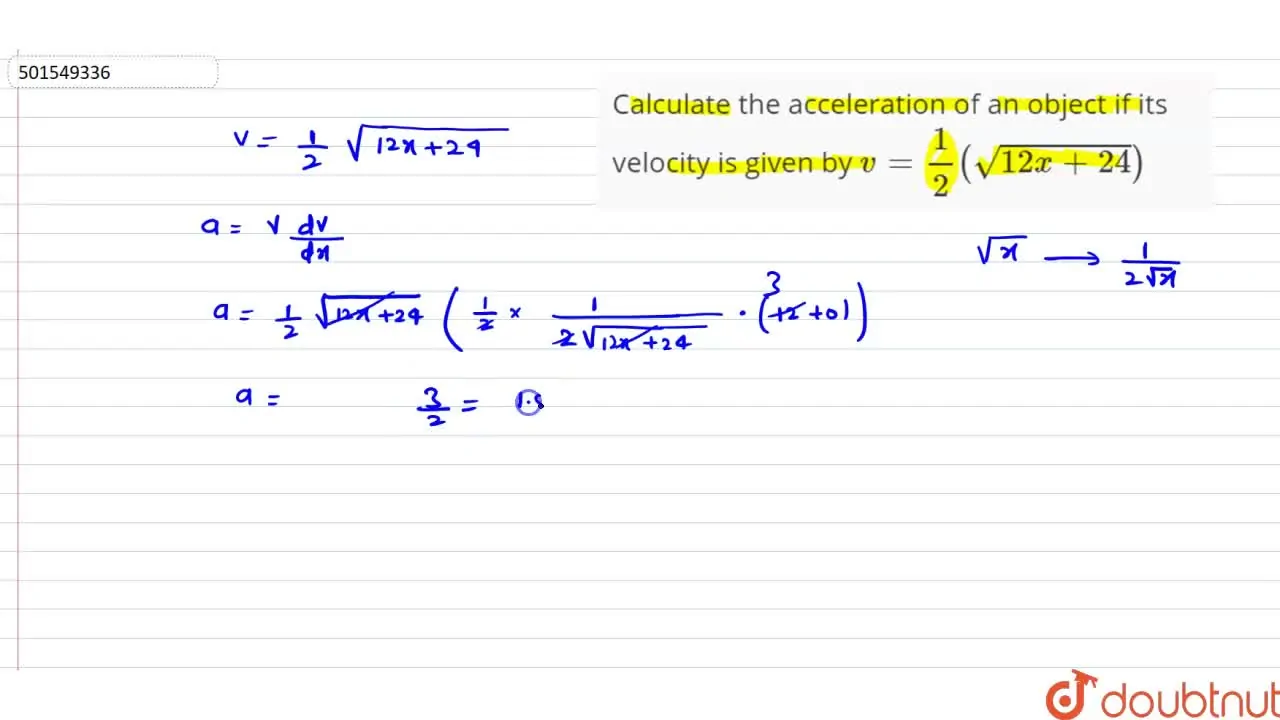
Calculate the acceleration of an object if its velocity is given by v=12(√12x+24)
Acceleration can be calculated as:
a=dvdt=dvdx×dxdt=vdvdx
From equation,
v=12(√12x+24)
⇒v2=12(12x+24)
⇒2vdvdx=14×12
⇒a=vdvdx=128=1.5ms−2
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - REVISION EXERCISE (LONG ANSWER QUESTIONS)5videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - REVISION EXERCISE (NUMERICAL PROBLEMS )7videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - REVISION EXERCISE (FILL IN THE BLANKS)10videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test15videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)12videos
OSCILLATIONS
Similar Questions
Calculate the acceleration of an object if its velocity is given by v=(√12t−2).
View SolutionThe acceleration (a) of an object varies as a function of its velocity (v) as a=λ√v where λ is a constant.If at t=0,v=0, then the velocity as a function of time (t) is given as
View SolutionThe acceleration (a) of an object varies as a function of its velocity (v) as a=λ√v where λ is a constant.If at t=0,v=0 ,then the velocity as a function of time (t) is given as (A)34λ2t3 (B)14λt (C)14λ2t2 (D)14λ2t
View Solution" The displacement x of a particle at the instant when its velocity v is given by "v=sqrt(3x)+16" .Find its acceleration "
View SolutionThe velocity-time graph of an object is given below. Calculate the average acceleration of the object in the time interval of 4 s to 6 s.
 View Solution
View SolutionThe displacement x of a particle at the instant when its velocity is v is given by v=√3x+16. Its initial velociyt is
View Solution[" The displacement "x" of a particle at the "],[" instant when its velocity "v" is given by "],[v=sqrt(3x+16)" .Find its acceleration "],[[" (A) "1.5" units "],[" (B) "2.5" units "],[" (C) "3.5" units "],[" (D) "4.5" units "]]
View SolutionThe velocity function of an object moving along a straight line is given by v(t)=At2+Bt. If at t=2s, the velocity is 3 m/s and acceleration is 0.500m/s2, find the value of the constant A.
View SolutionThe velocity time relation of a particle is given by v =(3t2−2t−1)m/s Calculate the position and acceleration of the particle when velocity of the particle is zero . Given the initial position of the particle is 5m .
View SolutionGive an example of a case in which an object's velocity is zero but its acceleration is not. Can an object's velocity ever be in a direction other than the direction of its acceleration? Explain.
View Solution