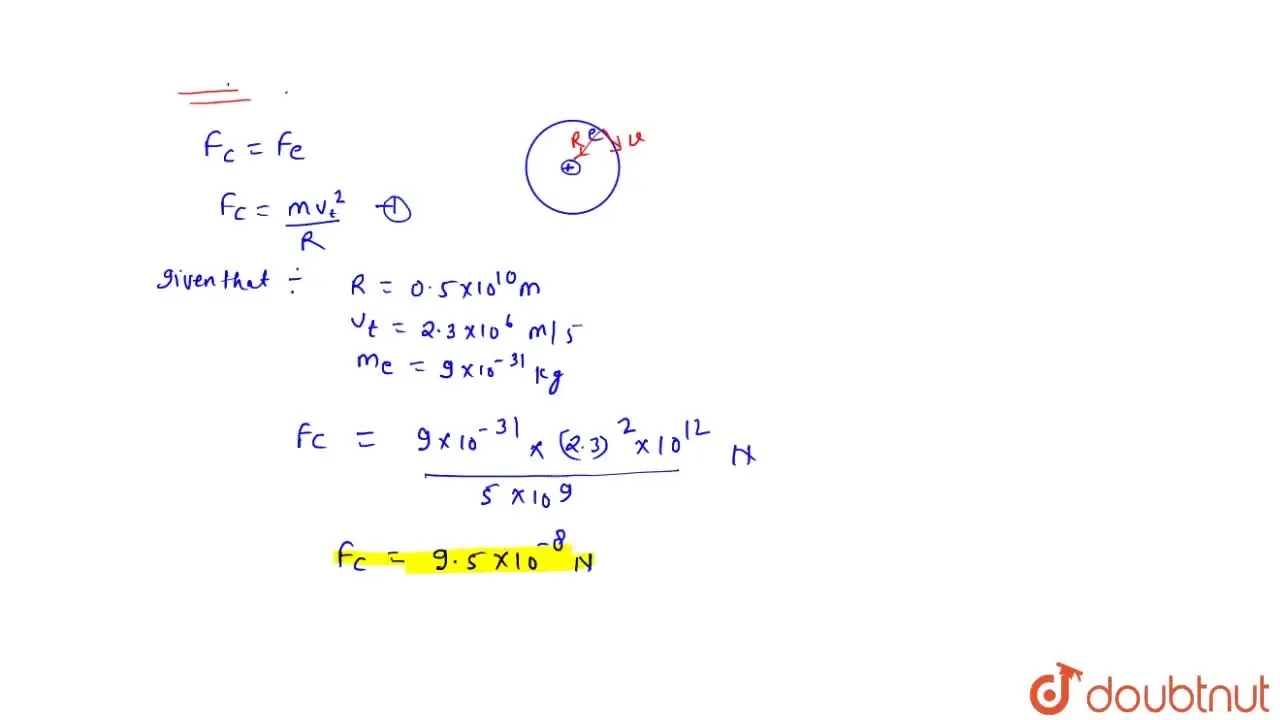
In the Bohr model of the hydrogen atom an electron is pictured rotating in a circle (with a radius of 0.5×10−10m) about the positive nucleus of the atom. The centripetal force is furnished by the electric attraction of the positive nucleus for the negative electron. How large is this force if the electron is moving with a speed of 2.3×106m/s?
(The mass of an electron is 9×10−31kg)
The correct Answer is:9.5×10−8N
Step by step video, text & image solution for In the Bohr model of the hydrogen atom an electron is pictured rotating in a circle (with a radius of 0.5xx10^(-10)m) about the positive nucleus of the atom. The centripetal force is furnished by the electric attraction of the positive nucleus for the negative electron. How large is this force if the electron is moving with a speed of 2.3xx10^(6)m/s? (The mass of an electron is 9xx10^(-31)kg) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(SINGLE CORRECT CHOICE TYPE)40videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(MORE THAN ONE CORRECT CHOICE TYPE)6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - CHECK POINTS6videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)3videos
CIRCUITS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
Similar Questions
In the Bohr model of hydrogen atom, the electron is pictured to rotate in a circular orbit of radius 5×10−11m, at a speed 2.2×106 m/ s. What is the current associated with electron motion?
View SolutionIn the Bohr model of hydrogen atom, the electron is pictured to rotate in a circular orbit of radius 5×10−11m, at a speed 2.2×106 m/ s. What is the current associated with electron motion?
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionAn electron is moving in an orbit in hydrogen atom of radius 0.5×10−10 m. Calculate the time period of electron about the nucleus
View SolutionThe electron in the hydrogen atom is moving with a speed at 2.3×106m/sin an orbit of radius 0.53 Å.The magnetic of the revolving electron is
View SolutionThe de broglie wavelength of an electron moving with a speed of 6.6×105m/s is of the order of (h=6.6×10−34Js and me=9×10−31kg)
View SolutionAn electron is moving in a circular orbit of radius 5.3×10−11 metre around the atomic nucleus at a rate of 6.6×1015 revolutions per second. The centripetal force acting on the electron will be - (The mass of the electron is 9.1×10−31kg)
View SolutionIn the Bohr model of hydrogen atom, the electron is treated as a particle going in a circle with the centre at the proton. The proton itself is assumed to be fiex in an inertial frame. The centripetal force is provided byi the Coloumb attraction. In the ground state, the electron goes round the proton in a circle of radius 5.3×10−11m. Find the speed of the electron in the ground state. Mass of the electron =9.1×10−31kg and charge of the electron =1.6×10−19C.
View SolutionThe mass of an electron at rest is 9.1×10−31 kg. the mass of an electron, when it is moving with a speed of 2.4×108 m/s is (c=3×108m/s)
View SolutionThe mass of an electron at rest is 9.1×10−31 kg. The energy of electron when it moves with speed of 1.8×108 m/s is
View Solution