A cone full of water, is placed on its side on a horizontal table, the thrust on its base is x times the weight of the contained fluid, where 2α is the vertical angle of the cone. Find the value of x.
3cosα
3sinα
2sinα
2cosα
The correct Answer is:B
To solve the problem, we need to determine the value of x such that the thrust on the base of a cone full of water placed on its side is x times the weight of the contained fluid.
1. Understanding the Geometry of the Cone:
- The cone is placed on its side, and we denote the vertical angle of the cone as 2α. Thus, the half-angle at the base of the cone is α.
- The radius r of the base of the cone can be related to the height h of the cone using the tangent function:
r=htanα
2. Calculating the Volume of the Cone:
- The volume V of the cone can be expressed as:
V=13πr2h
- Substituting r=htanα:
V=13π(htanα)2h=13πh3tan2α
3. Calculating the Weight of the Fluid:
- The weight W of the fluid contained in the cone is given by:
W=ρVg=ρ(13πh3tan2α)g
- Therefore, the weight of the fluid becomes:
W=13πρgh3tan2α
4. Calculating the Thrust on the Base:
- The thrust T on the base of the cone is given by the product of pressure and area. The pressure at the base due to the height of the fluid is:
P=ρgh
- The area A of the base is:
A=πr2=π(htanα)2=πh2tan2α
- Thus, the thrust T can be calculated as:
T=P⋅A=(ρgh)⋅(πh2tan2α)=πρgh3tan2α
5. Relating Thrust to Weight:
- We need to find x such that:
T=xW
- Substituting the expressions for T and W:
πρgh3tan2α=x(13πρgh3tan2α)
- Simplifying this equation:
1=x3
- Therefore, we find:
x=3
Final Answer:
The value of x is 3.
- The cone is placed on its side, and we denote the vertical angle of the cone as 2α. Thus, the half-angle at the base of the cone is α.
- The radius r of the base of the cone can be related to the height h of the cone using the tangent function:
r=htanα
- The volume V of the cone can be expressed as:
V=13πr2h
- Substituting r=htanα:
V=13π(htanα)2h=13πh3tan2α
- The weight W of the fluid contained in the cone is given by:
W=ρVg=ρ(13πh3tan2α)g
- Therefore, the weight of the fluid becomes:
W=13πρgh3tan2α
- The thrust T on the base of the cone is given by the product of pressure and area. The pressure at the base due to the height of the fluid is:
P=ρgh
- The area A of the base is:
A=πr2=π(htanα)2=πh2tan2α
- Thus, the thrust T can be calculated as:
T=P⋅A=(ρgh)⋅(πh2tan2α)=πρgh3tan2α
- We need to find x such that:
T=xW
- Substituting the expressions for T and W:
πρgh3tan2α=x(13πρgh3tan2α)
- Simplifying this equation:
1=x3
- Therefore, we find:
x=3
The value of x is 3.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDS BOOK - DISHA PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-2 CONCEPT APPLICATOR
24 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - DISHA PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-2 CONCEPT APPLICATOR
24 Videos
- MAGNETISM AND MATTERBOOK - DISHA PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE-2:CONCEPT APPLICATOR
31 Videos
- MOTION IN A PLANE BOOK - DISHA PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Exercise -2 : CONCEPT APPLICATOR
28 Videos
Similar Questions
Knowledge Check
If the lateral surface of right circular cone is 2 times its base , then the semi-vertical angle of the cone must be :
A15∘B30∘C45∘D60∘If the lateral surface of right circular cone is 2 times its base , then the semi-vertical angle of the cone must be :
A15∘B30∘C45∘D60∘A conic surface is placed in a uniform electric field E as shown in figure. Such that the field is perpendicular to the surface on the side AB. The base of the cone is of radius R, and the height of the cone is h. The angle of the cone is θ.

Find the magnitude of the flux that enters the cone's curved surface from the left side. Do not count the outgoing flux (θ<45∘).AER[hcosθ+π(R/2)sinθ]BER[hcosθ+πR/2sinθ]CER[hcosθ+πRsinθ]Dnone of these
A hollow cone floats with its axis vertical upto one third of its height in a liquid in a liquid of relative density 0.8 and with its vertex submerged. When another liquid of relative density ρ is filled in it upto one third of its height, the cone floats upto half its vertical height. The height of the cone is 0.10m and the radius of the circular base is 0.05m. Find the specific gravity ρ is given.
View SolutionA cone made of paper has height 3h and vertical angle 2α. It contains two other cones of height 2h and h and vertical angle 4α and 6α. Find the ratio of the two volume in between the cones.
View Solution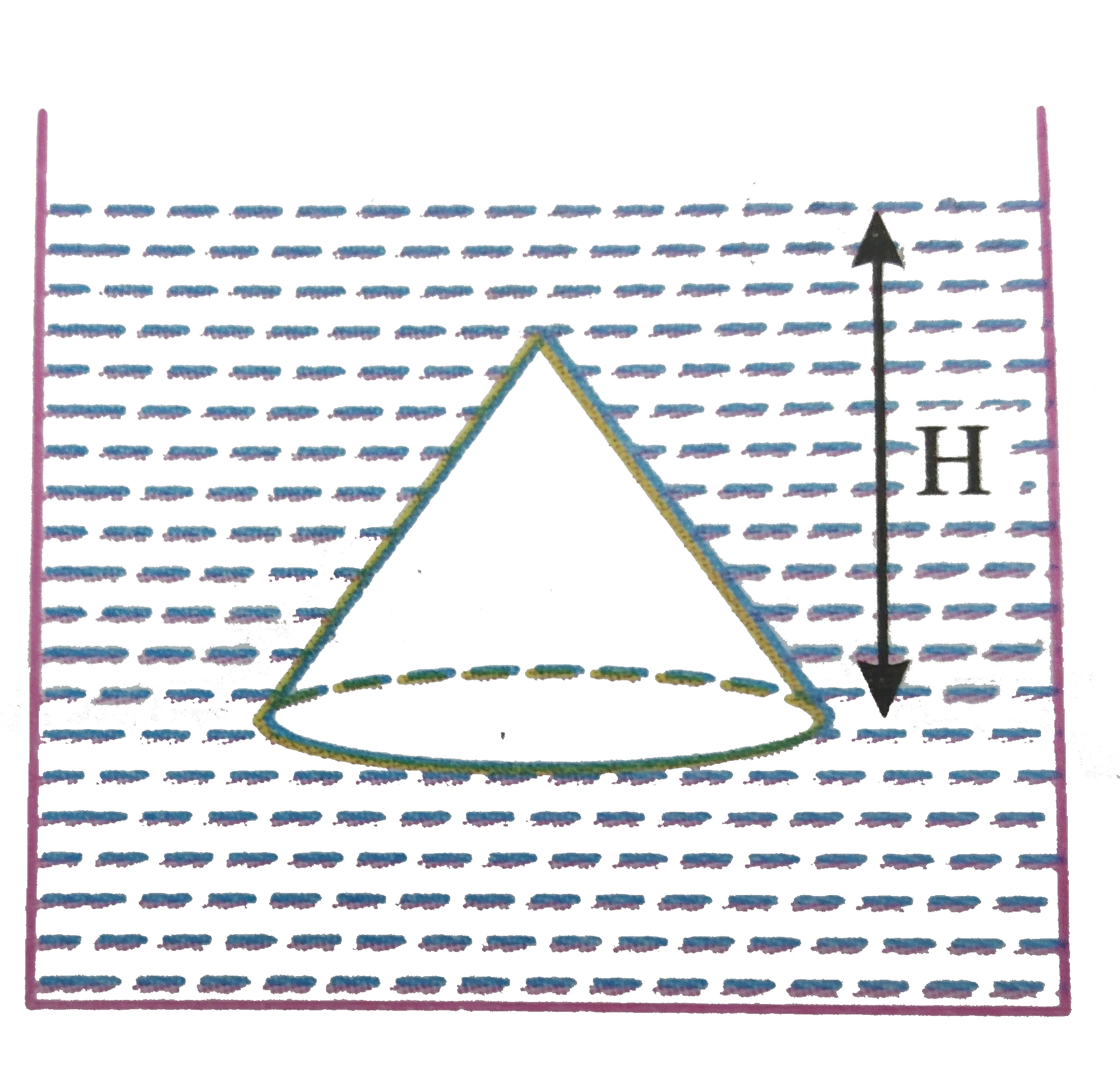
A cone of radius r and height r is under a liquid of density d.its base is parallel to the free surface of the liquid at a depth H from it as shown.What is the net force due to liquid on its curved surface? (neglect atmospheric pressure)
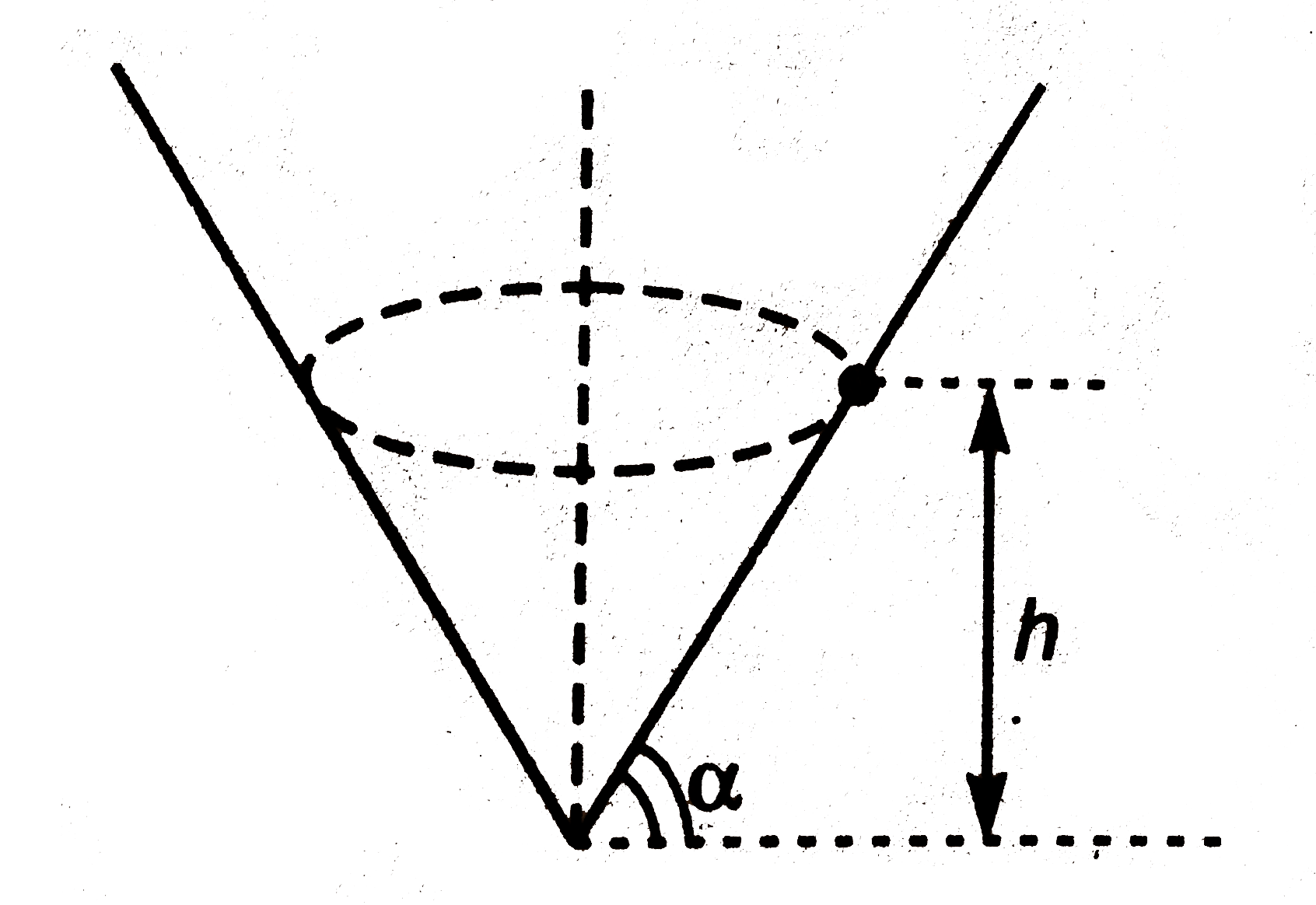
View SolutionA particle is describing circular motion in a horizontal plane in contact with the smooth surface of a fixed rigid circular cone with its axis vertical and vertex down. The height of the plane of motion above the vertex is h and the semi-vertical angle of the cone is α The period of revolution of the particle
View SolutionA conical cup when filled with ice - cream forms a hemispherical shape on its open end . Find the approximate volume of the ice - cream ,if the radius of the base of the cone is 3.5 cm and the vertical height of the cone is 7cm.
View Solution
