A comet moves in an elliptical orbit with an eccentricity of e = 0.20 around a star. The distance between the perihelion and the aphelion is 1.0×108 km. If the speed of the comet at perihelion is 81 km/s, then speed of the comet at the aphelion is :
182 km/s
36 km /s
121.5 km/s
54 km/s
The correct Answer is:D
To solve the problem, we need to find the speed of the comet at aphelion given its speed at perihelion and the distance between the perihelion and aphelion.
Step 1: Understand the terms
- Perihelion (r₁): The point in the orbit where the comet is closest to the star.
- Aphelion (r₂): The point in the orbit where the comet is farthest from the star.
- The distance between perihelion and aphelion is given as 1.0×108 km.
Step 2: Relate perihelion and aphelion distances
The distance between the perihelion and aphelion can be expressed as:
d=r2−r1
where d is the distance between perihelion and aphelion.
Step 3: Express perihelion and aphelion in terms of semi-major axis (a) and eccentricity (e)
For an elliptical orbit:
- The perihelion distance r1=a(1−e)
- The aphelion distance r2=a(1+e)
Thus, the distance between perihelion and aphelion can be expressed as:
d=r2−r1=a(1+e)−a(1−e)=2ae
Step 4: Solve for semi-major axis (a)
Given that d=1.0×108 km and e=0.20:
1.0×108=2a(0.20)
1.0×108=0.4a
a=1.0×1080.4=2.5×108 km
Step 5: Calculate perihelion and aphelion distances
Now we can find r1 and r2:
r1=a(1−e)=2.5×108(1−0.20)=2.5×108×0.80=2.0×108 km
r2=a(1+e)=2.5×108(1+0.20)=2.5×108×1.20=3.0×108 km
Step 6: Use conservation of angular momentum
The angular momentum L at perihelion and aphelion is conserved:
L=m⋅r1⋅v1=m⋅r2⋅v2
where v1 is the speed at perihelion and v2 is the speed at aphelion.
Since mass m cancels out:
r1⋅v1=r2⋅v2
Step 7: Solve for speed at aphelion
Substituting the known values:
(2.0×108)⋅(81)=(3.0×108)⋅v2
162×108=3.0×108⋅v2
v2=162×1083.0×108=54 km/s
Final Answer
The speed of the comet at aphelion is 54 km/s.
- Perihelion (r₁): The point in the orbit where the comet is closest to the star.
- Aphelion (r₂): The point in the orbit where the comet is farthest from the star.
- The distance between perihelion and aphelion is given as 1.0×108 km.
The distance between the perihelion and aphelion can be expressed as:
d=r2−r1
where d is the distance between perihelion and aphelion.
For an elliptical orbit:
- The perihelion distance r1=a(1−e)
- The aphelion distance r2=a(1+e)
d=r2−r1=a(1+e)−a(1−e)=2ae
Given that d=1.0×108 km and e=0.20:
1.0×108=2a(0.20)
1.0×108=0.4a
a=1.0×1080.4=2.5×108 km
Now we can find r1 and r2:
r1=a(1−e)=2.5×108(1−0.20)=2.5×108×0.80=2.0×108 km
r2=a(1+e)=2.5×108(1+0.20)=2.5×108×1.20=3.0×108 km
The angular momentum L at perihelion and aphelion is conserved:
L=m⋅r1⋅v1=m⋅r2⋅v2
where v1 is the speed at perihelion and v2 is the speed at aphelion.
r1⋅v1=r2⋅v2
Substituting the known values:
(2.0×108)⋅(81)=(3.0×108)⋅v2
162×108=3.0×108⋅v2
v2=162×1083.0×108=54 km/s
The speed of the comet at aphelion is 54 km/s.
Topper's Solved these Questions
- GRAVITATIONBOOK - DISHA PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - EXERCISE-2
30 Videos
- GRAVITATIONBOOK - DISHA PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - EXERCISE-2
30 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - DISHA PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE 2: CONCEPT APPLICATOR
24 Videos
- JEE MAINS- 2019 (HELD ON :9TH APRIL 2019 (SHIFT-I))BOOK - DISHA PUBLICATIONCHAPTER - JEE MAINS- 2019 (HELD ON :9TH APRIL 2019 (SHIFT-I))EXERCISE - QUESTIONS
30 Videos
Similar Questions
The mean orbital radius of the Earth around the Sun is 1.5×108km. Estimate the mass of the Sun.
View SolutionThe point of an elliptical orbit closest to the Sun is called the perihelion, and the point most distant from it is called aphelion (Fig). Denoting the distance from the perihelion to the Sun by r0 and the velocity of the planet at the perihelion by v0 , find the radius of curvature of the orbit at the perihelion and at the aphelion, the distance from the aphelion to the Sun, and the velocity of the planet at the aphelion.
Prove that the motion of a planet in an elliptical orbit is only possible, if its total energy is negative.

View Solution
Knowledge Check
A planet revolves about the sun in elliptical orbit. The arial velocity (dAdt) of the planet is 4.0×1016m2/s. The least distance between planet and the sun is 2×1012m. Then the maximum speed of the planet in km/s is -
A10B20C40DNone of theseA comet (assumed to be in an elliptical orbit around the sun) is at a distance of 0.4 AU from the sun at the perihelion. If the time period of the comet is 125 years, what is the aphelion distance ? AU : Astronomical Unit.
A50 AUB25 AUC49.6 AUD24.6 AUThe distance between two stations is 20 km. If a train moves with a constant speed of 60 km h−1, then the time taken by the train to reach the next station is _____.
A2 hourB20 minuteC20 secondD40 minute
The maximum and minimum distances of a comet from the Sun are 1.6×1012m and 8.0×1010mrespectively. If the speed of the comet at the nearest point is 6×104ms−1, the speed at the farthest point is:
View SolutionA satelite revolves around a planet in an elliptical orbit. Its maximum and minimum distances from the planet are 1.5×107 m and 0.5×107 m respectively. If the speed of the satellite at the farthest point be 5×103 m/s, calculate the speed at the nearest point.
View SolutionA comet is going around the sun in an elliptical orbit with a period of 64 year. The closest approach of the comet to the sun is 0.8 AU [AU = astronomical unit]. Calculate the greatest distance of the comet form the sun.
View SolutionTwo planets of equal mass orbit a much massive star (figure). Planet m1 moves in circular orbit of radius 1×108km with period 2yr. Planet m2 moves in an elliptical orbit with closed distance r1=1×108km and farthest distance r2=1.8×108km, as shows.
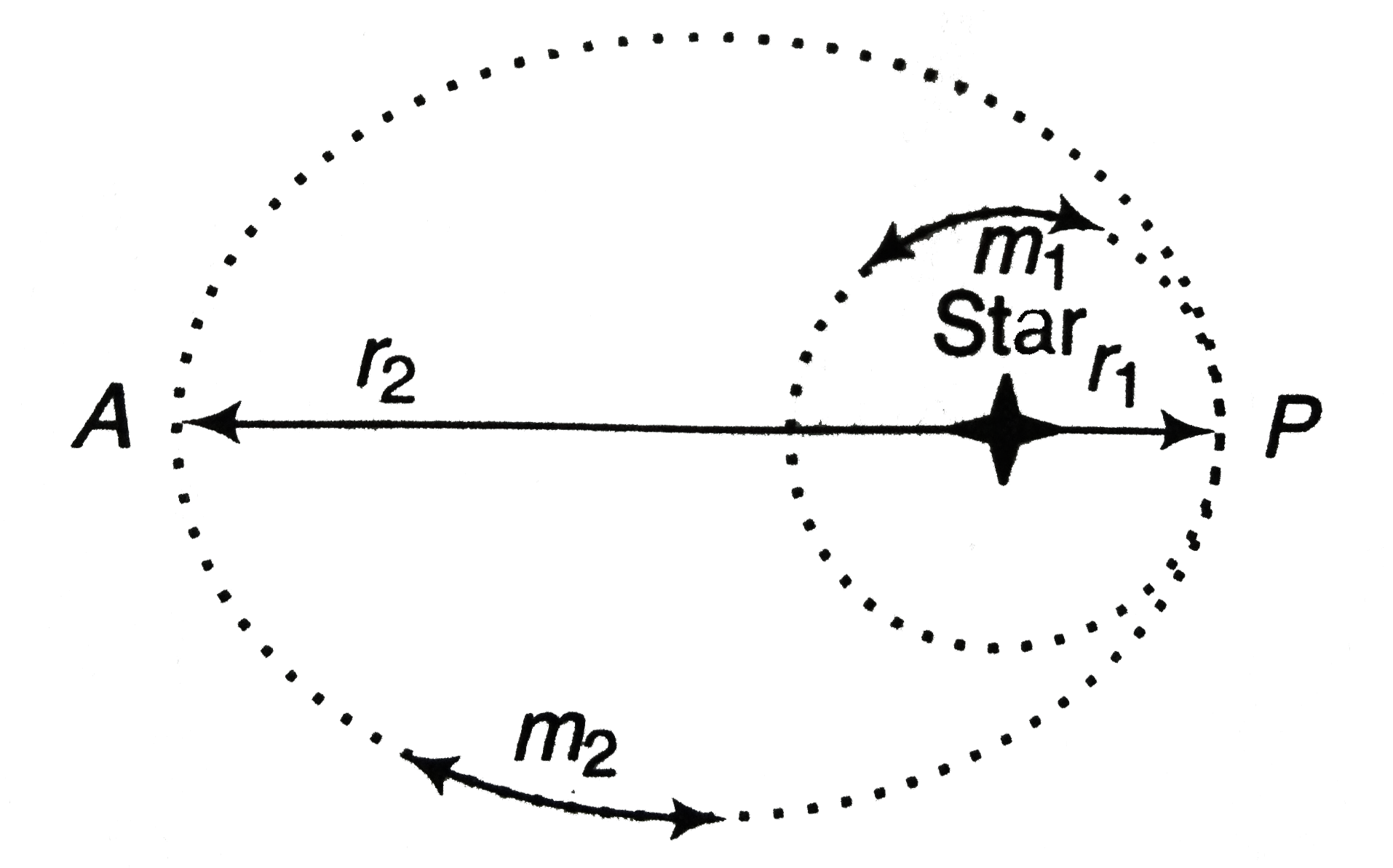
(a) Using the fact that the mean radius of an elliptical orbit is the length of the semi-major axis, find the period of m2's orbit.
(b) Which planet has the greater speed at point P? Which has the greater total energy?
(c ) Compare the speed of planet m2 at P with that at A.
View SolutionThe orbit of Pluto is much more eccentric than the orbits of the other planets. That is, instead of being nearly circular, the orbit is noticeably elliptical. The point in the orbit nearest to the sun is called the perihelion and the point farthest from the sun is called the aphelion.

As Pluto moves from the perihelion to the aphelion, the work done by gravitational pull of Sun on Pluto is
View Solution
