The least coefficient of friction for an inclined plane inclined at angle with horizontal in order that a solid cylinder will roll down without slipping is
The correct Answer is:C
To find the least coefficient of friction for a solid cylinder rolling down an inclined plane without slipping, we can follow these steps:
1. Identify Forces Acting on the Cylinder:
- The gravitational force acting downwards: Fg=mg.
- The component of gravitational force acting down the incline: Fdown=mgsinα.
- The normal force acting perpendicular to the incline: N=mgcosα.
- The frictional force acting up the incline: Ff.
2. Apply Newton's Second Law:
- For linear motion along the incline:
mgsinα−Ff=ma
- Here, a is the linear acceleration of the center of mass of the cylinder.
3. Relate Linear Acceleration to Angular Acceleration:
- The frictional force causes a torque about the center of the cylinder:
τ=Ff⋅r
- The moment of inertia I of a solid cylinder about its axis is given by:
I=12mr2
- The angular acceleration α is related to the linear acceleration a by:
a=rα
4. Write the Torque Equation:
- Using the relation for torque:
Ff⋅r=Iα
- Substituting I and α:
Ff⋅r=12mr2⋅ar
- Simplifying gives:
Ff=12ma
5. Substitute Ff in the Linear Motion Equation:
- Replace Ff in the linear motion equation:
mgsinα−12ma=ma
- Rearranging gives:
mgsinα=32ma
- Thus, we can express a:
a=2gsinα3
6. Find the Frictional Force:
- Now substitute a back to find Ff:
Ff=12ma=12m⋅2gsinα3=mgsinα3
7. Relate Frictional Force to Normal Force:
- The maximum static friction force is given by:
Ff≤μsN
- Substituting N=mgcosα:
mgsinα3≤μs(mgcosα)
- Dividing through by mg (assuming m≠0):
sinα3≤μscosα
8. Solve for the Coefficient of Friction:
- Rearranging gives:
μs≥sinα3cosα
- This can be simplified using the tangent function:
μs≥13tanα
Final Result:
The least coefficient of friction required for a solid cylinder to roll down an inclined plane without slipping is:
μs=13tanα
- The gravitational force acting downwards: Fg=mg.
- The component of gravitational force acting down the incline: Fdown=mgsinα.
- The normal force acting perpendicular to the incline: N=mgcosα.
- The frictional force acting up the incline: Ff.
- For linear motion along the incline:
mgsinα−Ff=ma
- Here, a is the linear acceleration of the center of mass of the cylinder.
- The frictional force causes a torque about the center of the cylinder:
τ=Ff⋅r
- The moment of inertia I of a solid cylinder about its axis is given by:
I=12mr2
- The angular acceleration α is related to the linear acceleration a by:
a=rα
- Using the relation for torque:
Ff⋅r=Iα
- Substituting I and α:
Ff⋅r=12mr2⋅ar
- Simplifying gives:
Ff=12ma
- Replace Ff in the linear motion equation:
mgsinα−12ma=ma
- Rearranging gives:
mgsinα=32ma
- Thus, we can express a:
a=2gsinα3
- Now substitute a back to find Ff:
Ff=12ma=12m⋅2gsinα3=mgsinα3
- The maximum static friction force is given by:
Ff≤μsN
- Substituting N=mgcosα:
mgsinα3≤μs(mgcosα)
- Dividing through by mg (assuming m≠0):
sinα3≤μscosα
- Rearranging gives:
μs≥sinα3cosα
- This can be simplified using the tangent function:
μs≥13tanα
The least coefficient of friction required for a solid cylinder to roll down an inclined plane without slipping is:
μs=13tanα
Topper's Solved these Questions
- SYSTEM OF PARTICLES & ROTATIONAL MOTION Book:DISHA PUBLICATIONChapter:SYSTEM OF PARTICLES & ROTATIONAL MOTION Exercise:EXERCISE-2 : CONCEPT APPLICATOR
Explore 28 Videos
- SYSTEM OF PARTICLES & ROTATIONAL MOTION Book:DISHA PUBLICATIONChapter:SYSTEM OF PARTICLES & ROTATIONAL MOTION Exercise:EXERCISE-2 : CONCEPT APPLICATOR
Explore 28 Videos
- SEMICONDUCTOR ELECTRONICS : METERIALS, DEVICES AND SIMPLE CIRCUITSBook:DISHA PUBLICATIONChapter:SEMICONDUCTOR ELECTRONICS : METERIALS, DEVICES AND SIMPLE CIRCUITSExercise:EXERCISE -2: CONCEPT APPLICATOR
Explore 25 Videos
- THERMAL PROPERTIES OF MATTER Book:DISHA PUBLICATIONChapter:THERMAL PROPERTIES OF MATTER Exercise:Exercise-2 (Concept Applicator)
Explore 28 Videos
Similar Questions
A hollow sphere rolls down a rough inclined plane whose angle of inclination is θ, with horizontal. For the hollow sphere to undergo pure rolling, without slipping, the condition is [μ = coefficient of friction between the hollow sphere and inclined plane]
View SolutionA solid cylinder with base radius jis placed on top of an inclined plane of length land slope angle α (Fig). The cylinder rolls down without slipping. Find the speed of the centre of mass of the cylinder at the bottom of the plane, if the coefficient of rolling friction is k. Can rolling friction be neglected? Do the calculation for the following conditions: l=1m,α=30∘,r=10cm,k=5×10−4m . What would be the speed if, in the absence of friction, the cylinder slides down?
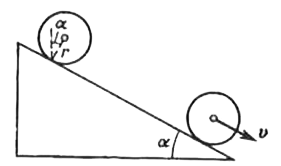
View Solution
Knowledge Check
A uniform sphere of mass m and radius r rolls without slipping down a inclined plane, inclined at an angle 45∘ to the horizontal . Find the magnitude of frictional coefficient at which slipping is absent :
A13B27C15D17If a solid cylinder rolls down an inclined plane, then its:
Arotational energy is 1/3 and the translational energy 2/3 of its total K.E.Btranslational energy is 1/3 and rotational energy is 2/3 of its total K.E.Crotational and translational energies are equal.Dentire energy is rotational K.E.A block is at rest on an inclined plane making an angle α with the horizontal . As the angle α of the incline is increased the block starts slipping when the angle of inclination becomes θ. The coefficient of static friction between the block and the surface of the inclined plane is or A body starts sliding down at an angle θ to the horizontal. Then the coefficient of friction is equal to
AsinθBcosθCtanθDIndependent of θ
An inclined plane makes an angle of 60∘ with horizontal. A disc rolling down this inclined plane without slipping has a linear acceleration equal to
View SolutionA block is at rest on an inclined plane making an angle α with the horizontal. As the angle of the incline is increased the block start slipping when the angle of inclination becomes θ then coefficient of friction is equal to
View SolutionA solid cylinder of mass m rolls without slipping down an inclined plane making an angle θ with the horizontal. The frictional force between the cylinder and the incline is
View SolutionA sphere of mass M rolls without slipping on the inclined plane of inclination θ. What should be the minimum coefficient of friction, so that the sphere rolls down without slipping ?
View SolutionA homogeneous ball is placed on a plane making an angle θ with the horizontal. At what values of the coefficient of friction μ the ball roll down the plane without slipping ?
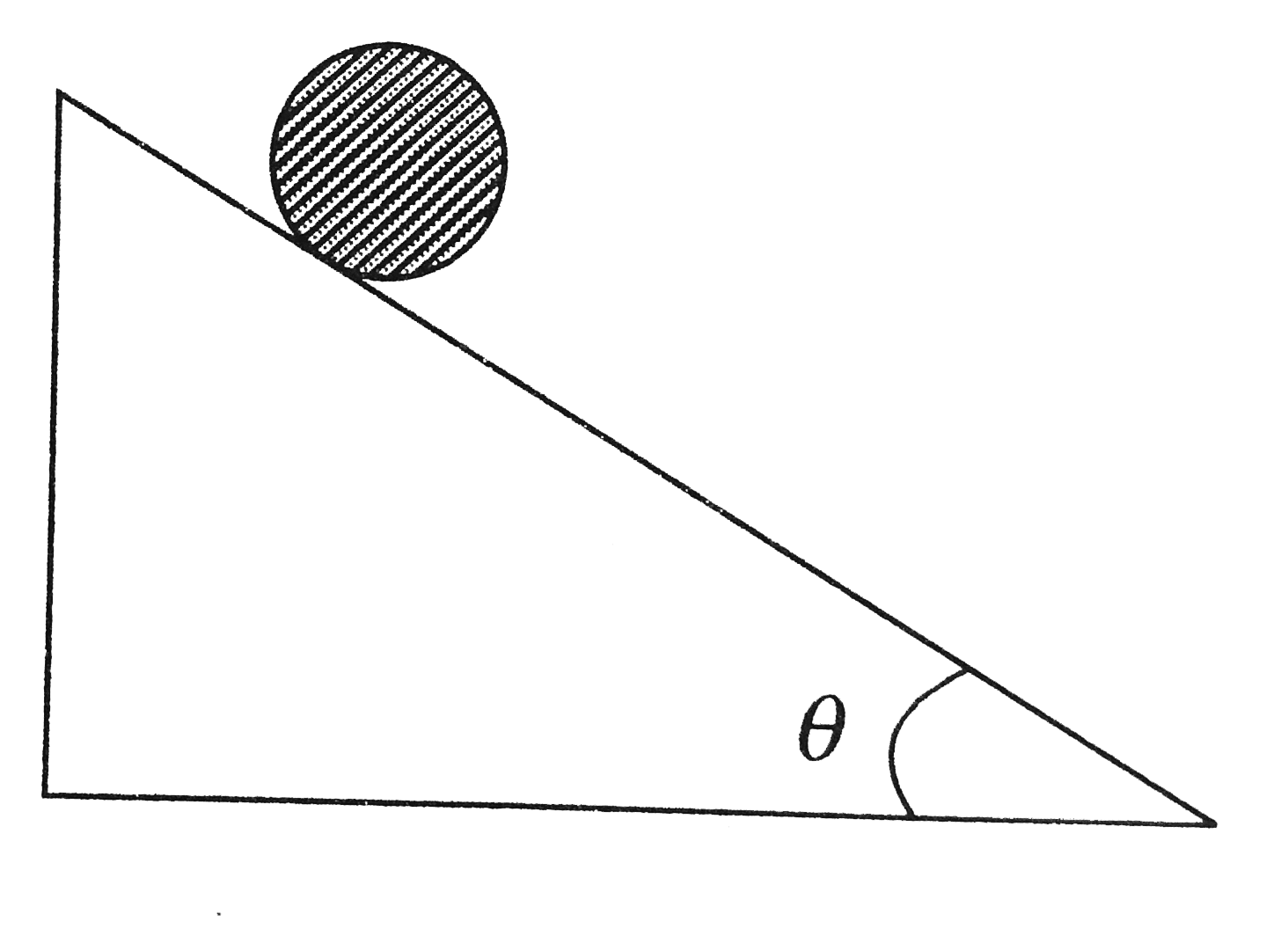 .
.
View Solution