The x-coordiante of the incentre of the triangle that has the coordiantes of mid points of its sides as (0,1),(1,1) and (1,0) is:

A

2+√2

B

2−√2

C

1+√2

D

1−√2

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

If D(x1,y1,E(x2,y2)andF(x3,y3) are mid-points of the sides BC,AC and AB respectively of ΔABC. Then, the coordinates of vertices are A(x2+x3−x1,y2+y3−y1),B(x3+x1−x2,y3+y1−y2)andC(x1+x2−x3,y1+y2,y3)
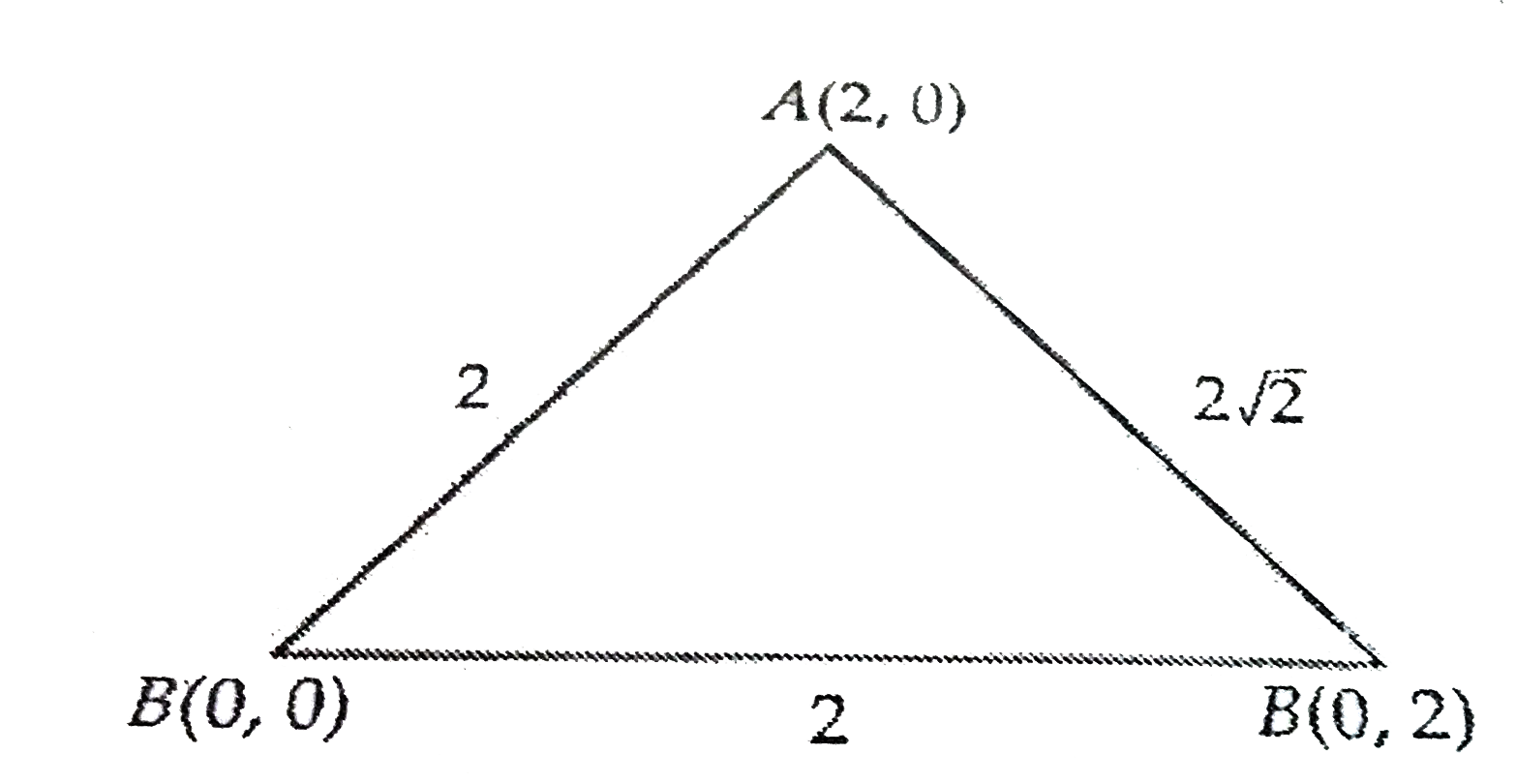
ocr_image
In the given problem the coordinates of the mid-points are D(0,1),E(1,1) and F((1,0). so, the coordinates of vertices are A((2,0) B(0,0) and C(0,2).
∴AB=2,BC=2andAC=2√2
Hence, the coordinats of the incentre are
(2×2×+2√2×0+2×02+2√2+2,2×0+2√2×0+2×22+2√2+2)
(22+√2,22+√2)or(2−√2,2−√2)

Updated on:21/07/2023
|
Class 11MATHSCOORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The x -coordinate of the incentre of the triangle that has the coordinates of mid-points its sides are (0,1), (1,1) and (1, 0) is

    A2+√2
    B2−√2
    C1+√2
    D1−√2

  • The x -coordinate of the incentre of the triangle that has the coordinates of mid-points its sides are (0,1), (1,1) and (1, 0) is

    A2+√2
    B2−√2
    C1+√2
    D1−√2

  • The x-coordinates of the incentre of the triangle that has the coordinates of mid-point of its sides as (0, 1), (1, 1) and (1, 0), is

    A2+√2
    B2−√2
    C1+√2
    D1−√2