Let A(h, k), B(1, 1) and C(2, 1) be the vertices of a right angled triangle with AC as its hypotenuse. If the area of the triangle is 1, then the set of values which k can take is given by

A

{1,3}

B

{0,2}

C

{−1,3}

D

{−3,−2}

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Since ordinates of B and C are same. So BC is parallel to x-axis . Also, ∠CB=90∘ . Therefore, BA is prallele to y-aixs. So, x-coordinate of A is 1 i.e., h=1
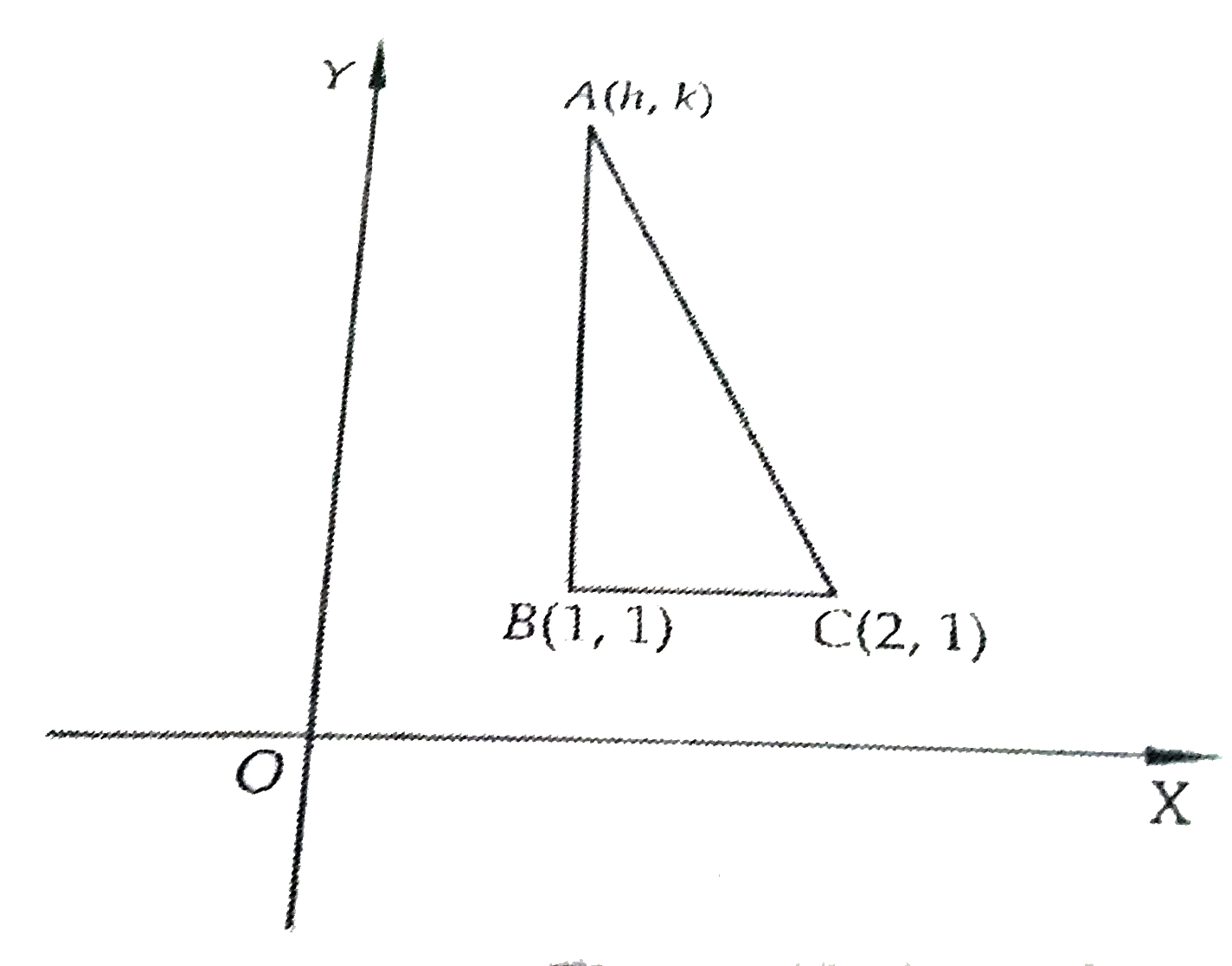
ocr_image
Now, Area of ΔABC=1 sq. unit
⇒12BC×AB=1
⇒12×1×|k−1|=1
⇒|k−|=2⇒k−1=±2⇒k=−1,3

Updated on:21/07/2023
|
Class 11MATHSCOORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Let A(1,k), B(1,1) and C(2,1) be the vertices of a right angled triangle with AC as its hypotenuse. If the area of the triangle is 1, then the set of values which 'k' can take is given by

    A(1,3)
    B(0,2)
    C(-1,3)
    D(-3,-2)

  • Let A(1,k),B(1,1) and C(2,1) be the vertices of a right angled triangle with AC as its hypotenuse. If the area of the triangle is 1, then the set of values which k can take is given by:

    A(1,3)
    B(0,2)
    C(-1,3)
    D(-3,-2)

  • Let A(1,k),B(1,1) and C(2,1) be the vertices of a right angled triangle with AC as its hypotenuse. If the area of the triangle is 1, then the set of values which k can take is given by:

    A{1, 3}
    B{0, 2}
    C{-1, 3}
    D{-3, -2}