A sphere and a cube having same volume and height of cube is equal to the radius of the sphere placed on a horizontal surface. What is the ratio of the heights of their centre of gravities from the surface?
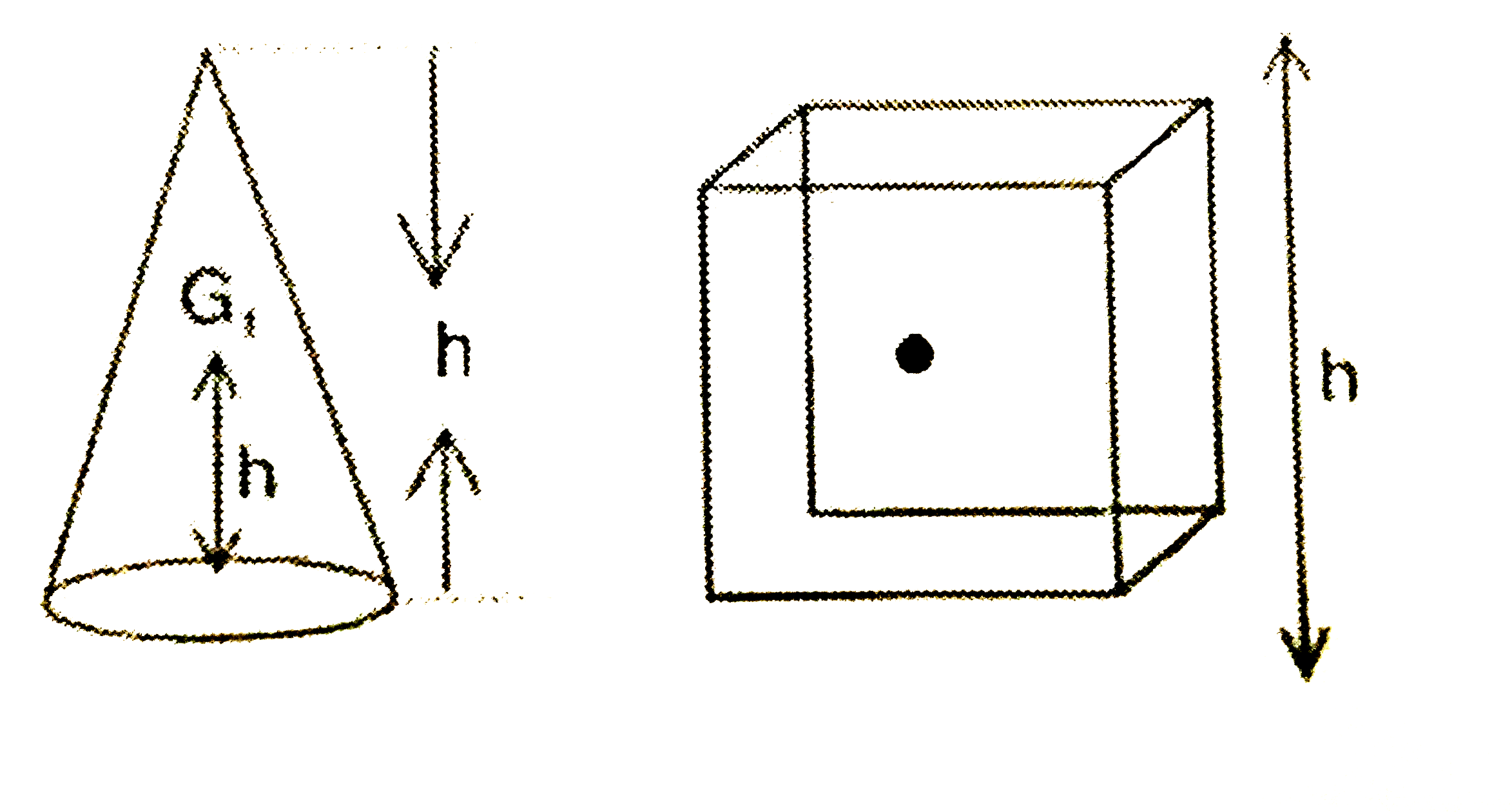
The heights of the centre of gravity of the sphere and cube from their respective base are
handh2⇒hh−2=21
Topper's Solved these Questions
- GRAVITATIONBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GRAVITATIONEXERCISE - LEVEL-3
10 Videos
- GRAVITATIONBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GRAVITATIONEXERCISE - LEVEL-1
44 Videos
- ElectricityBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ElectricityEXERCISE - Level 3
10 Videos
- HEATBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - HEATEXERCISE - All Questions
115 Videos
Similar Questions
The side of a cube is equal to the radius of the sphere. Find the ratio of their volumes.
View SolutionA cylinder and a hollow sphere are placed on a surface. If the height of the cylinder is equal to the diameter of the sphere, what is the ratio of the heights of centre of gravity of the cylinder and the sphere from the surfaced?
View Solution
Knowledge Check
A cone and a cylinder having same base area and height are placed on a horizontal surface. What is the ratio of the heights of centre of gravity of the cone and the cylinder from the surface?
A5 : 3B3 : 5C3 : 2D1 : 2
A cube and a sphere have equal total surface area. Find the ratio of the volume of sphere and cube.
View SolutionA sphere, a cylinder and a cone having same radius and height of cylinder and cone are equal to diameter of sphere. Ratio of their volume are
View SolutionA sphere and a cube are of the same height. The ratio of their volume is
View SolutionIn a sphere is inscribed in a cube, find the ratio of the volume of cube to the volume of the sphere.
View SolutionThe sphere and cube have same surface. Show that the ratio of the volume of sphere to that of cube is √6 : √π
View SolutionA sphere and a cube has same surface area.Show that the ratio of the volume of sphere to cube is √6:√π
View SolutionA sphere, a cylinder and a cone have the same radius and same height. Find the ratio of their volumes.
View Solution
