If $\frac{sin i}{sin r} = n_{21} > 1 .$ Out of medium 1 and medium 2, which is more optically denser ?

Video Solution

Text Solution

Generated By DoubtnutGPT

To determine which medium is more optically dense based on the given relationship $(sin i) / (sin r) = n_{21} > 1$ , we can follow these steps:
Step 1: Understand the relationship
The equation $(sin i) / (sin r) = n_{21}$ is derived from Snell's Law, which states:
$n_{1} sin i = n_{2} sin r$
where:
- $n_{1}$ is the refractive index of medium 1,
- $n_{2}$ is the refractive index of medium 2,
- $i$ is the angle of incidence,
- $r$ is the angle of refraction.
Step 2: Analyze the condition $n_{21} > 1$
Since $n_{21} = n_{2} / n_{1}$ and it is given that $n_{21} > 1$ , this implies:
$\frac{n_{2}}{n_{1}} > 1$
This means that $n_{2} > n_{1}$ .
Step 3: Relate refractive indices to optical density
The refractive index of a medium is directly related to its optical density. A higher refractive index indicates a denser medium. Therefore, since $n_{2} > n_{1}$ , it follows that:
- Medium 2 is more optically dense than Medium 1.
Conclusion
From the analysis, we conclude that Medium 2 is more optically dense than Medium 1.
---

Updated on:18/9/2024

A beam of light passes from medium 1 to medium 2 to medium 3 as shown figure. What may be concluded about the three indices of refraction, $n_{1}, n_{2}, and n_{3}$ ?

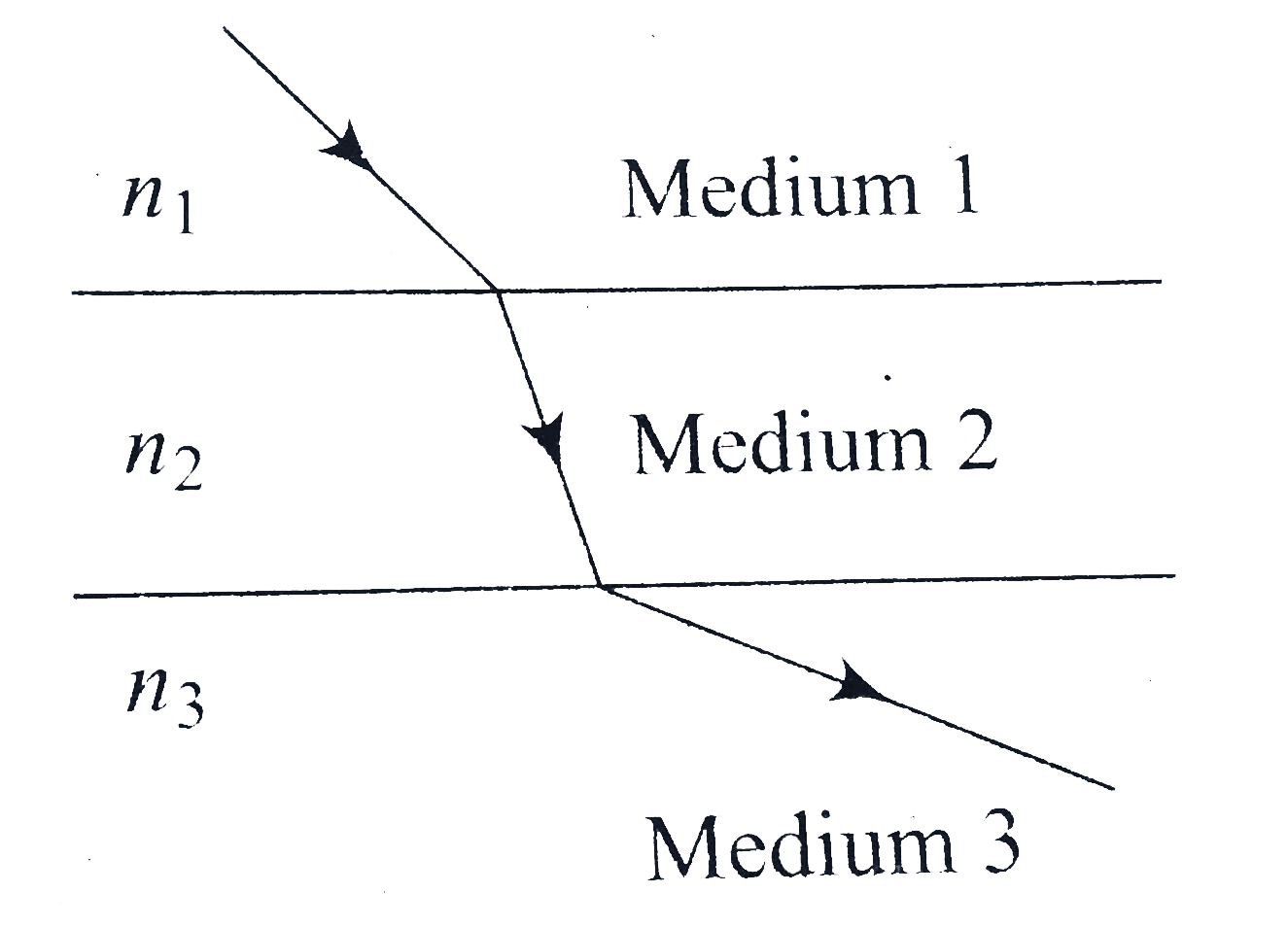A $n_{3} > n_{1} > n_{2}$
B $n_{1} > n_{3} > n_{2}$
C $n_{2} > n_{3} > n_{1}$
D $n_{2} > n_{1} > n_{3}$
The critical angle for refraction from medium -1 to air is $θ_{1}$ and that from medium _2 to air is $θ_{2}$ . If medium _2 is denser than medium _1. The critical angle for refraction from medium _2 to medium _ 1 is
A ${sin}^{- 1} (\frac{{sin θ}_{2}}{{sin θ}_{1}})$
B ${sin}^{- 1} (\frac{{sin θ}_{1}}{{sin θ}_{2}})$
C ${sin}^{- 1} ({sin θ}_{2})$
D ${sin}^{- 1} ({sin θ}_{1})$