To determine the refractive index of a liquid experimentally, a mark is made on the beaker and a microscope was focussed on it, giving a reading of 3.42 cm. On pouring liquid till a height 2.68 cm inside the beaker, the reading of refocussed microscope is 3.38 cm. Calculate the refractive index of the liquid.
To determine the refractive index of a liquid experimentally, we can follow these steps:
Step 1: Identify the initial and final readings
- Initial reading (before pouring the liquid): 3.42 cm
- Final reading (after pouring the liquid): 3.38 cm
Step 2: Calculate the apparent shift
The apparent shift can be calculated using the formula:
Apparent Shift=Initial Reading−Final Reading
Substituting the values:
Apparent Shift=3.42cm−3.38cm=0.04cm
Step 3: Identify the real depth
The real depth (T) of the liquid in the beaker is given as:
T=2.68cm
Step 4: Use the formula relating apparent shift and refractive index
The relationship between the apparent shift, real depth, and refractive index (μ) is given by:
Apparent Shift=T(1−1μ)
Substituting the known values:
0.04cm=2.68cm(1−1μ)
Step 5: Rearranging the equation to solve for μ
First, we can isolate 1−1μ:
1−1μ=0.042.68
Calculating the right side:
1−1μ=0.014925
Step 6: Solve for 1μ
Now, we can isolate 1μ:
1μ=1−0.014925=0.985075
Step 7: Calculate the refractive index (μ)
Taking the reciprocal gives us:
μ=10.985075≈1.015
Final Answer
The refractive index of the liquid is approximately:
μ≈1.015
---
- Initial reading (before pouring the liquid): 3.42 cm
- Final reading (after pouring the liquid): 3.38 cm
The apparent shift can be calculated using the formula:
Apparent Shift=Initial Reading−Final Reading
Substituting the values:
Apparent Shift=3.42cm−3.38cm=0.04cm
The real depth (T) of the liquid in the beaker is given as:
T=2.68cm
The relationship between the apparent shift, real depth, and refractive index (μ) is given by:
Apparent Shift=T(1−1μ)
Substituting the known values:
0.04cm=2.68cm(1−1μ)
First, we can isolate 1−1μ:
1−1μ=0.042.68
Calculating the right side:
1−1μ=0.014925
Now, we can isolate 1μ:
1μ=1−0.014925=0.985075
Taking the reciprocal gives us:
μ=10.985075≈1.015
The refractive index of the liquid is approximately:
μ≈1.015
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (1)
13 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (2)
9 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
While determining the refractive index of a liquid experimentally, the microscope was focussed at the bottom of a beaker, when its reading was 3.965 cm. on pouring liquid upto a height 2.537 cm inside the beaker, the reading of the refocussed microscope was 3.348 cm. Find the refractive index of the liquid.
View SolutionA mark is made on the bottom of beaker and a microscope is focussed on it. The microscope is raised through 1.5cm. To what height water must be poured into the beaker to bring the mark again into focus ? Given that μ for water is 4/3.
View Solution
Knowledge Check
A thin equiconvex lens of refractive index 3/2 is placed on a horizontal plane mirror as shown in figure. The space between the lens and the mirror is filled with a liquid of refractive index 4/3 . It is found that when a point object is placed 15 cm above the lens on its principal axis, the object coincides with its own image.
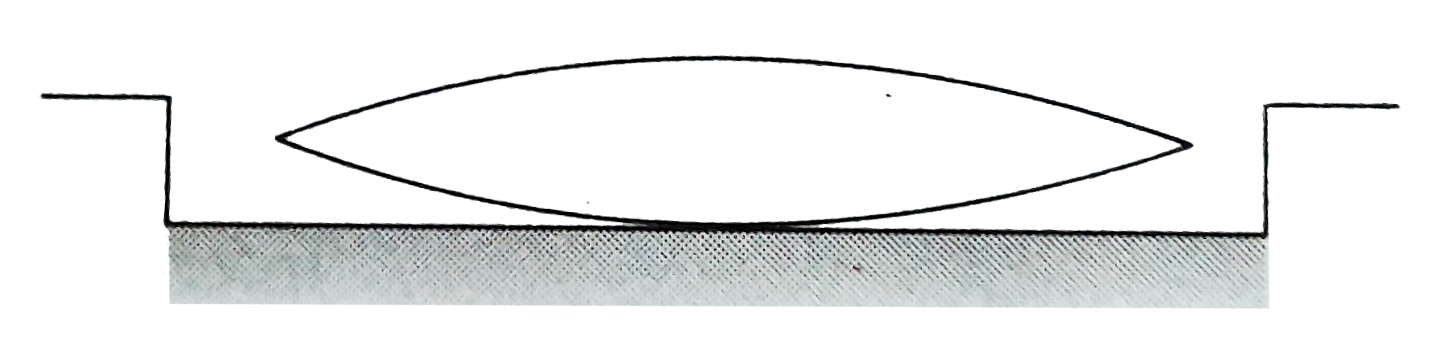
Q. If another liquid is filled instead of water, the object and the image coincide at a distance 25 cm from the lens.
Calculate the refractive index of the liquid.A1.6B3.2C0.8D2A beaker containing liquid is placed on a table, underneath a microscope which can be moved along a vertical scale. The microscope is focused, through the liquid onto a mark on the table when the reading on the scale is a. It is next focused on the upper surface of the liquid and the reading is b. More liquid is added and the observations are repeated, the corresponding readings are c and d. The refractive index of the liquid is
Ad−bd−c−b+aBb−dd−c−b+aCd−c−b+ad−bDd−ba+b−c−dA microscope is focussed on an ink mark on the top of a table. If we place a glass slab of 3 cm thick on it, how should the microscope be moved to focus the ink spot again? The refractive index of glass is 1.5
A2 cm upwardsB2 cm downwardsC1 cm upwardsD1 cm upwards
A travelling microscope is focussed on a mark made on a paper. When a slab of 1.45cm thickness is placed on the marl, the microscope has to be raised through 0.49 cm to focus the mark again. Calculate the refractive index of glass.
View SolutionA convex lens has a refractive index 1.5 and power 7 diopter. When the lens is immersed in a liquid, it behaves as a concave lens of focal length 120 cm. Calculate the refractive index of the liquid.
View SolutionA thin convex lens made of glass of refractive index 3/2 has a power of 5 D. When it is immersed in a liquid of refractive index μ, it behaves like a divergent lens of focal length 1 m. Calculate the refractive index of the liquid.
View SolutionA mark at the bottom of a bekar is focussed by a microscope .When water is poured in the beaker up to a height of16cm,the microscope has to be raised through a distance of4cm in order to bring the mark to focus again .Calculate the refractive index of water.
View SolutionA jar 15cm long is filled with a transparent liquid. When viewed from the top, its bottom appears to be 12cm below. What is the refractive index of the liquid ?
View Solution
