Question

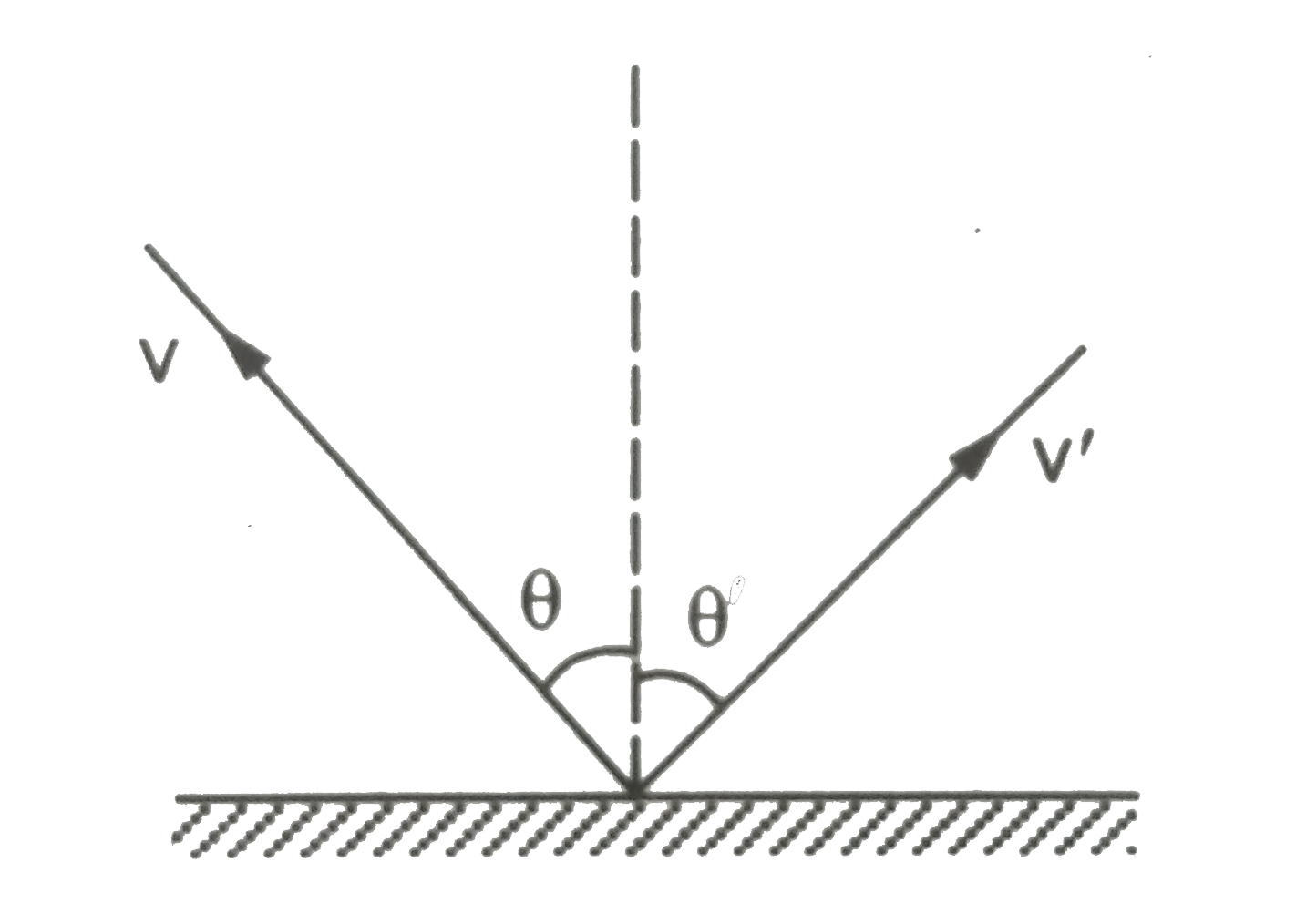
A particle of mass m is strikes on ground at an angle of incidence ${30}^{\circ }$ with initial speed of 5 m/s . Calculate (i) its velocity after impact (ii) angle of reflection . Given that the coefficient of restitution is $\frac{1}{\sqrt{3}}$

Explanation

To solve the problem step by step, we will break down the calculations for both parts of the question: (i) the velocity after impact and (ii) the angle of reflection.

Step 1: Resolve the initial velocity into components
The initial velocity \( V \) of the particle is given as 5 m/s, and it strikes the ground at an angle of incidence of \( 30^\circ \).

- The horizontal component of the velocity \( V_x \) can be calculated using:
\( V_x = V \cdot \cos(30^\circ) = 5 \cdot \frac{\sqrt{3}}{2} = \frac{5\sqrt{3}}{2} \text{ m/s} \)

- The vertical component of the velocity \( V_y \) can be calculated using:
\( V_y = V \cdot \sin(30^\circ) = 5 \cdot \frac{1}{2} = 2.5 \text{ m/s} \)

Step 2: Analyze the impact
Since the surface is frictionless, the horizontal component of the velocity remains unchanged after the impact:
\( V_x' = V_x = \frac{5\sqrt{3}}{2} \text{ m/s} \)

Step 3: Calculate the vertical component after impact using the coefficient of restitution
The coefficient of restitution \( e \) is given as \( \frac{1}{\sqrt{3}} \). The relationship between the velocities before and after impact in the vertical direction is given by:
\( e = \frac{\text{Velocity of separation}}{\text{Velocity of approach}} \)
Here, the velocity of approach in the vertical direction is \( V_y \) and the velocity of separation is \( V_y' \):
\( \frac{1}{\sqrt{3}} = \frac{V_y'}{V_y} \)
Substituting the values:
\( \frac{1}{\sqrt{3}} = \frac{V_y'}{2.5} \)
Solving for \( V_y' \):
\( V_y' = 2.5 \cdot \frac{1}{\sqrt{3}} = \frac{2.5}{\sqrt{3}} \text{ m/s} \)

Step 4: Calculate the magnitude of the velocity after impact
Now, we can find the resultant velocity \( V' \) after impact using the Pythagorean theorem:
\( V' = \sqrt{(V_x')^2 + (V_y')^2} \)
Substituting the values:
\( V' = \sqrt{\left(\frac{5\sqrt{3}}{2}\right)^2 + \left(\frac{2.5}{\sqrt{3}}\right)^2} \)
Calculating:
\( V' = \sqrt{\left(\frac{75}{4}\right) + \left(\frac{6.25}{3}\right)} = \sqrt{\frac{75}{4} + \frac{25}{12}} \)
Finding a common denominator and simplifying:
\( V' = \sqrt{\frac{225 + 100}{12}} = \sqrt{\frac{325}{12}} = \frac{\sqrt{325}}{2\sqrt{3}} \text{ m/s} \)

Step 5: Calculate the angle of reflection
The angle of reflection \( r \) can be calculated using:
\( \tan(r) = \frac{V_x'}{V_y'} \)
Substituting the values:
\( \tan(r) = \frac{\frac{5\sqrt{3}}{2}}{\frac{2.5}{\sqrt{3}}} = \frac{5\sqrt{3} \cdot \sqrt{3}}{2 \cdot 2.5} = \frac{15}{5} = 3 \)
Thus, the angle \( r \) can be calculated as:
\( r = \tan^{-1}(3) \)

Final Answers
1. The velocity after impact is \( V' = \frac{\sqrt{325}}{2\sqrt{3}} \) m/s.
2. The angle of reflection is \( r = \tan^{-1}(3) \).