A charged particle having charge 2q and mass m is projected from origin in X-Y plane with a velocity v inclined at an angle of 45∘ with positive X-axis in a region where a uniform magnetic field B unit exists along +z axis. The coordinates of the centre of the circular path of the particle are
(0,0,0)
(−mv√2qB,mv√2qB,0)
(−√2mvqB,√2mvqB,0)
none of the above
The correct Answer is:D
To solve the problem of finding the coordinates of the center of the circular path of a charged particle projected in a magnetic field, we can follow these steps:
1. Understanding the Problem:
- We have a charged particle with charge 2q and mass m.
- It is projected from the origin (0, 0) in the XY plane with a velocity v at an angle of 45∘ with the positive X-axis.
- There is a uniform magnetic field B directed along the positive Z-axis.
2. Components of Velocity:
- The velocity v can be resolved into its components:
vx=vcos(45∘)=v√2,vy=vsin(45∘)=v√2
3. Magnetic Force:
- The magnetic force F acting on the particle is given by:
F=q(v×B)
- Here, the charge q=2q and the magnetic field B is along the Z-axis.
- The magnitude of the magnetic force can be calculated as:
F=2q⋅v⋅B
4. Centripetal Force:
- This magnetic force acts as the centripetal force required for circular motion:
F=mv2r
- Setting the two expressions for force equal gives:
2qvB=mv2r
5. Solving for Radius r:
- Rearranging the above equation to find the radius r:
r=mv2qB
6. Coordinates of the Center of the Circular Path:
- The center of the circular path will be offset from the origin due to the circular motion.
- The coordinates of the center can be determined using the radius and the angle of projection:
- The X-coordinate of the center will be:
x=rcos(45∘)=mv2qB⋅1√2=mv2qB√2
- The Y-coordinate of the center will be:
y=−rsin(45∘)=−mv2qB⋅1√2=−mv2qB√2
- The Z-coordinate is 0 since the motion is confined to the XY plane.
7. Final Coordinates:
- Thus, the coordinates of the center of the circular path are:
(mv2qB√2,−mv2qB√2,0)
- We have a charged particle with charge 2q and mass m.
- It is projected from the origin (0, 0) in the XY plane with a velocity v at an angle of 45∘ with the positive X-axis.
- There is a uniform magnetic field B directed along the positive Z-axis.
- The velocity v can be resolved into its components:
vx=vcos(45∘)=v√2,vy=vsin(45∘)=v√2
- The magnetic force F acting on the particle is given by:
F=q(v×B)
- Here, the charge q=2q and the magnetic field B is along the Z-axis.
- The magnitude of the magnetic force can be calculated as:
F=2q⋅v⋅B
- This magnetic force acts as the centripetal force required for circular motion:
F=mv2r
- Setting the two expressions for force equal gives:
2qvB=mv2r
- Rearranging the above equation to find the radius r:
r=mv2qB
- The center of the circular path will be offset from the origin due to the circular motion.
- The coordinates of the center can be determined using the radius and the angle of projection:
- The X-coordinate of the center will be:
x=rcos(45∘)=mv2qB⋅1√2=mv2qB√2
- The Y-coordinate of the center will be:
y=−rsin(45∘)=−mv2qB⋅1√2=−mv2qB√2
- The Z-coordinate is 0 since the motion is confined to the XY plane.
- Thus, the coordinates of the center of the circular path are:
(mv2qB√2,−mv2qB√2,0)
Topper's Solved these Questions
- PHYSICS PART2Book:FIITJEEChapter:PHYSICS PART2Exercise:Section (I) : Subjective
Explore 4 Videos
- PHYSICS PART2Book:FIITJEEChapter:PHYSICS PART2Exercise:Section (II) : MCQ
Explore 27 Videos
- PHYSICS PART-IIIBook:FIITJEEChapter:PHYSICS PART-IIIExercise:NUMERICAL BASED QUESTIONS DECIMAL TYPE
Explore 11 Videos
- ROTATIONAL MECHANICSBook:FIITJEEChapter:ROTATIONAL MECHANICSExercise:COMPREHENSION - IV
Explore 2 Videos
Similar Questions
A particle having mass m and charge q is projected with a velocity v making an angle q with the direction of a uniform magnetic field B. Calculate the magnitude of change in velocity of the particle after time t=πm2qB.
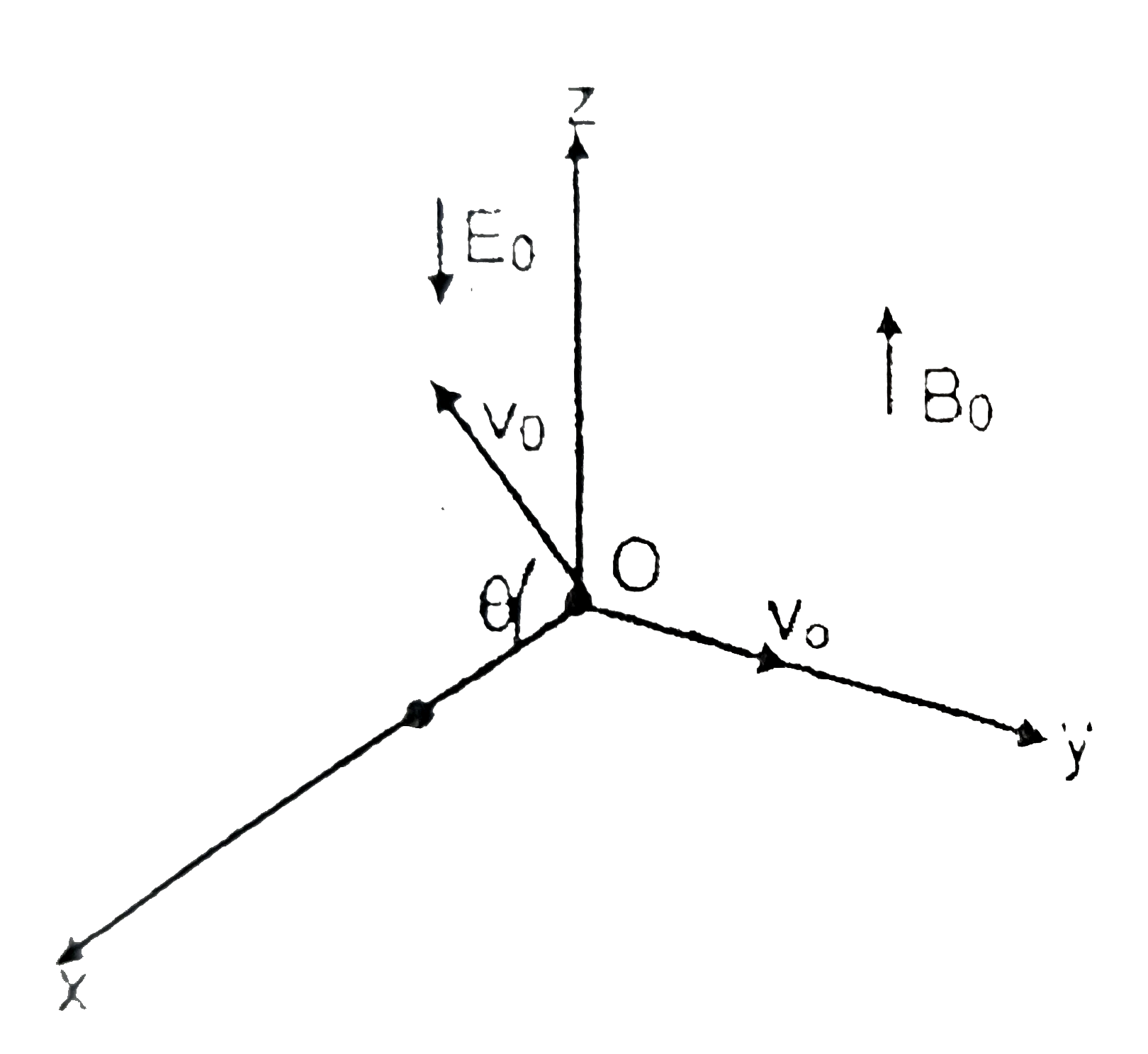
View SolutionA charged point particle having positive charge q, mass m is projected in x−z plane with a speed v0 at an angle θ with the x−axis , while another identical particle is projected simultaneously along the y−axis from the origin (see figure) with an equal speed v0.
An electric field →E=−E0ˆk exists in the x−z plane, while a magnetic field →B=B0ˆk exists in the region y>0 (F0, B0>0). It is observed that both the particles collide after some time. Assume that the space is gravity free.
(i) Find the time after which the particles collide and the coordinates of the point of collision.
(ii) Find the velocity v0 and the angle θ in terms of the other variables.
(iii) What is the maximum value of the z− coordinate of the first particle ?
View Solution
Knowledge Check
A charged particle is fired at an angle θ to a uniform magnetic field directed along the x-axis. During its motionalong a helical path, the particle will
Anever move parallel to the x-axisBmove parallel to the x-axis once during every rotation for all velues of θCmove parallel to the x-axis at least once during every rotation if θ=45∘Dnever move perpendicular to the x-directionIn a certain region of space, there exists a uniform and constant electric field of magnitude E along the positive y-axis of a coordinate system. A charged particle of mass m and charge -q (qgt0) is projected form the origin with speed 2v at an angle of 60∘ with the positive x-axis in x-y plane. When the x-coordinate of particle becomes √3mv2/qE a uniform and constant magnetic. field of strength B is also switched on along positive y-axis.
z-coordinate of the particle as a function of time after the magnetic field is switched on isAmvqB[1−cos(qBmt)]B−mvqB[1+cos(qBmt)]C−mvqB[1−cos(qBmt)]DmvqB[1+cos(qBmt)]In a certain region of space, there exists a uniform and constant electric field of magnitude E along the positive y-axis of a coordinate system. A charged particle of mass m and charge -q (qgt0) is projected form the origin with speed 2v at an angle of 60∘ with the positive x-axis in x-y plane. When the x-coordinate of particle becomes √3mv2/qE a uniform and constant magnetic. field of strength B is also switched on along positive y-axis.
x-coordinate of the particle as a function of time after the magnetic field is switched on isA√3mv2qE−mvqBsin(qBmt)B√3mv2qE+mvqBsin(qBmt)C√3mv2qE−mvqBcos(qBmt)D√3mv2qE+mvqBcos(qBmt)
An electron is projected along positive z-axis, magnetic field is present along y-axis then direction of initial acceleration of the particle is
View SolutionIn a certain region of space, there exists a uniform and constant electric field of magnitude E along the positive y-axis of a coordinate system. A charged particle of mass m and charge -q (qgt0) is projected form the origin with speed 2v at an angle of 60∘ with the positive x-axis in x-y plane. When the x-coordinate of particle becomes √3mv2/qE a uniform and constant magnetic. field of strength B is also switched on along positive y-axis.
Velocity of the particle just before the magnetic field is switched on is
View SolutionA particle of mass 'm' and charge 'q' is incident on XZ plane with velocity 'v' in a direction making angle θ with a uniform magnetic field applied along X-axis. The magnetic field applied along X-axis. The nature of motion performed by the particle is
View SolutionA charged particle of charge Q and massm moves with velocity v in a circular path due to transverse magnetic field, B, then its frequency is
View SolutionA charged particle of mass m and charge q enters a magnetic field B with a velocity v at an angle θ with the direction of B. The radius fo the resulting path is
View Solution
