Aa simple pendulum is suspended from the ceiling of a vehicle which moves down an inclined plane o inclination $Θ$ . The period of oscilation of the pendulum is

A

$2 π \sqrt{\frac{L}{g cos θ}}$

B

$2 π \sqrt{L \frac{cos θ}{g}}$

C

$2 π \sqrt{\frac{L}{g sin θ}}$

D

$2 π \sqrt{L \frac{sin θ}{g}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Acceleration of trolley is g $sin θ$ along the plane.

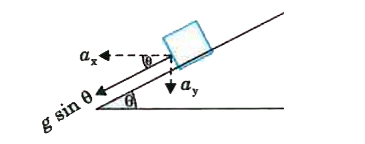
We can resolve acceleration of trolley along horizontal and vertical.
$a_{x} = (g sin θ) cos θ = g sin θ cos θ$
$a_{y} = (g sin θ) sin θ = g {sin}^{2} θ$
Effective acceleration due to gravity for hte pendulum can be calculated as follows :
$g_{e f f}^{2} = a_{x}^{2} + {(g - a_{y})}_{y}^{2}$
$= g^{2} {sin}^{2} θ {cos}^{2} θ + g^{2} + g^{2} {sin}^{4} θ - 2 g^{2} {sin}^{2} θ$
$= g^{2} {sin}^{2} θ [{cos}^{2} θ + {sin}^{2} θ] + g^{2} - 2 g^{2} {sin}^{2} θ$
$= g^{2} {sin}^{2} θ g^{2} - 2 g^{2} {sin}^{2} θ$
$= g^{2} - g^{2} {sin}^{2} θ$
$g^{2} (1 - {sin}^{2} θ) = g^{2} {cos}^{2} θ$
$\Rightarrow g_{e f f} = g cos θ$
$T = 2 π \sqrt{\frac{L}{g_{e f f}}}$
$\Rightarrow T = 2 π \sqrt{\frac{L}{g cos θ}}$
Hence option (a) is correct.

|

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The period of oscillation of a simple pendulum of length l suspended from the roof of a vehicle which moves down without friction on an inclined plane of inclination $θ$ , is given by
A $2 π \sqrt{\frac{l}{g cos θ}}$
B $2 π \sqrt{\frac{l}{g sin t θ}}$
C $2 π \sqrt{\frac{l}{g tan θ}}$
D $2 π \sqrt{\frac{l}{g}}$
Question 2 - Select One
A simple pendulum of length $l$ is connected to the ceiling of a vehicle that is moving down along a smooth inclined plane $4$ in $5$ . Then its period of oscillation is
A $2 π \sqrt{\frac{5 l}{4 g}}$
B $2 π \sqrt{\frac{4 l}{5 g}}$
C $2 π \sqrt{\frac{5 l}{3 g}}$
D $2 π \sqrt{\frac{3 l}{5 g}}$
Question 3 - Select One
A simple pendulum is suspended from the ceiling of a train which is moving with an acceleration a .The angular of inclination of the pendulum from the vertical will be
A $90^{\circ}$
B $0^{\circ}$
C ${tan}^{- 1} (\frac{a}{g})$
D ${tan}^{- 1} (\frac{g}{a})$

MODERN PUBLICATION-OSCILLATIONS -Competition File (Multiple choice questions)

Home
Ask Doubt
Profile