What will be the spring constant k of a spring if spring is divided in n equal parts?
To find the spring constant k′ of a spring when it is divided into n equal parts, we can follow these steps:
1. Understanding the Spring Constant:
The spring constant k relates the force F applied to the spring and the displacement x from its equilibrium position, given by Hooke's Law:
F=k⋅x
2. Dividing the Spring:
When the spring is divided into n equal parts, each part will have a new length. If the original length of the spring is L, then the length of each part after division will be:
L′=Ln
3. Force on Each Part:
The force F applied to the entire spring is the same as the force applied to each individual part. Therefore, for each part, we can express the relationship as:
F=k′⋅x′
where k′ is the spring constant of one part and x′ is the displacement of that part.
4. Relating Displacement:
Since each part is Ln long, the displacement x′ for each part when the same force F is applied will be:
x′=xn
where x is the total displacement of the original spring.
5. Substituting into Hooke's Law:
Now, substituting x′ into the equation for each part:
F=k′⋅xn
6. Equating Forces:
Since the force F is the same for the entire spring and for each part, we can equate:
k⋅x=k′⋅xn
7. Solving for k′:
To find k′, we can rearrange the equation:
k′=k⋅n
Conclusion:
Thus, the spring constant k′ of each part of the spring when it is divided into n equal parts is:
k′=n⋅k
The spring constant k relates the force F applied to the spring and the displacement x from its equilibrium position, given by Hooke's Law:
F=k⋅x
When the spring is divided into n equal parts, each part will have a new length. If the original length of the spring is L, then the length of each part after division will be:
L′=Ln
The force F applied to the entire spring is the same as the force applied to each individual part. Therefore, for each part, we can express the relationship as:
F=k′⋅x′
where k′ is the spring constant of one part and x′ is the displacement of that part.
Since each part is Ln long, the displacement x′ for each part when the same force F is applied will be:
x′=xn
where x is the total displacement of the original spring.
Now, substituting x′ into the equation for each part:
F=k′⋅xn
Since the force F is the same for the entire spring and for each part, we can equate:
k⋅x=k′⋅xn
To find k′, we can rearrange the equation:
k′=k⋅n
Thus, the spring constant k′ of each part of the spring when it is divided into n equal parts is:
k′=n⋅k
Topper's Solved these Questions
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Revision Exercises (Additional Question )
3 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Revision Exercises (fill in the blanks)
9 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - HOTS (Higher Order Thinking Skills & Advanced Level)
11 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
Similar Questions
Knowledge Check
Assertion : The spring constant of a spring is k. When it is divided into n equal parts, then springconstant of one piece is k/n.
Reason : The spring constant is independent of material used for the spring.AIf both assertion and reason are true and the reason is the correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of the assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is trueThe spring constant of spring A is twice the spring constant of spring B. Each of the spring is cut into two pieces. First piece of spring A is (4/5) of the total length. Second piece of spring B is (5/6) of its total length. Both springs are of equal lengh initially :
Athe ratio of force constant of first piece of spring B to the first piece of spring A is (12/5)Bthe ratio of force constant of first piece of spring B to the first piece of spring A is 2Cthe ratio of force constant of second piece of spring A to the first piece of spring B is 5/3Dthe ratio of force constant of second piece of spring A to the first piece of spring B is 7/5.Force constant of a spring (k) is anonymous to
AYALBYLACALYDALY
The spring constant of a spring depends on its
View SolutionOne body is suspended from a spring of length l, spring constant k and has time period T, Now if spring is divided in two equal parts which are joined in paralled and the same body is suspended from this arrangements then determine new time period.
View SolutionWhat is the spring constant for the combination of spring shown in fig. ?

View SolutionWhat will be the equivalent spring constant of the spring system shown in the figure ?
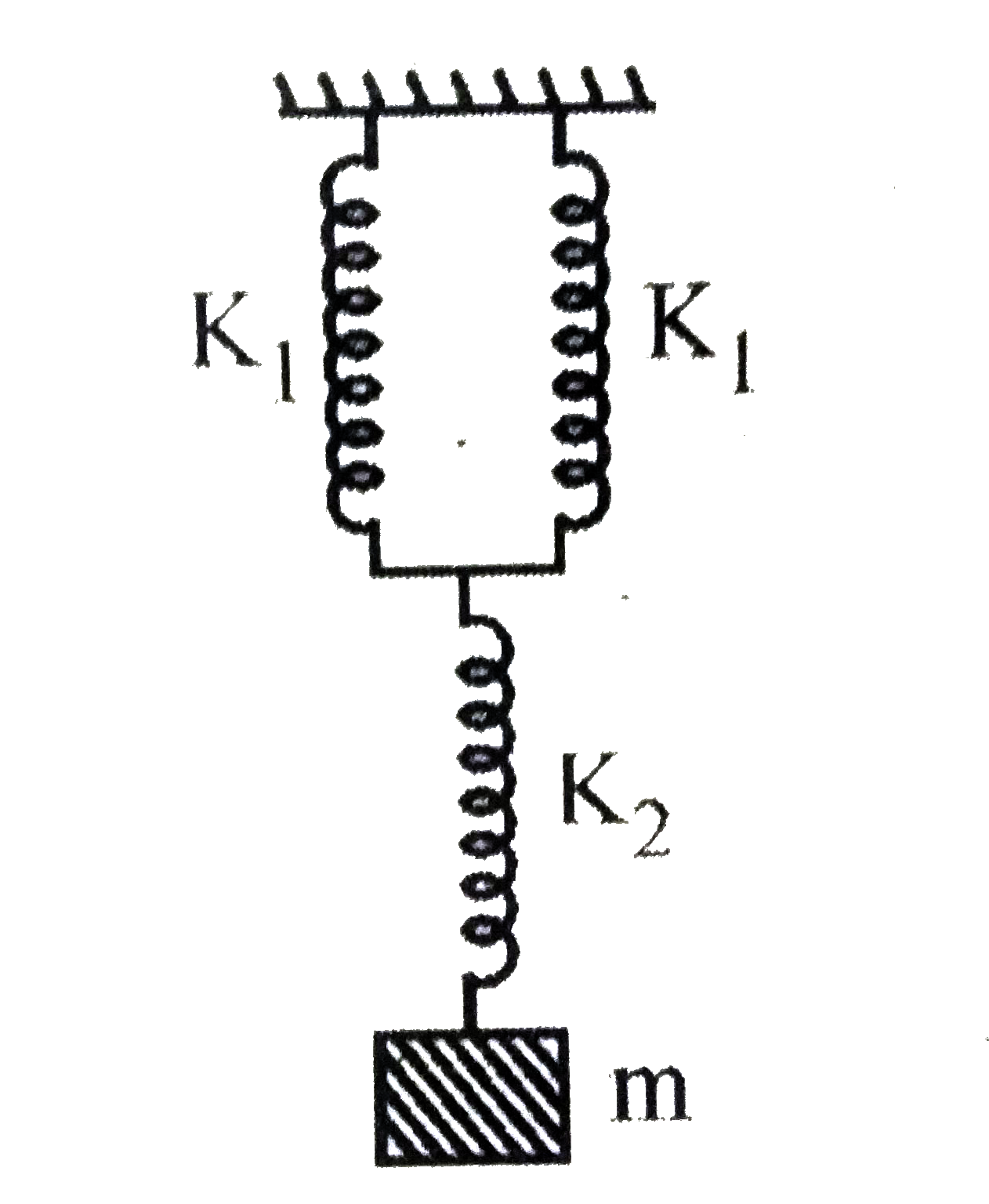
View SolutionA spring having a spring constant k is loaded with a mass m. The spring is cut into two equal parts and one of these is loaded again with the same mass. The new spring constant i
View Solution
