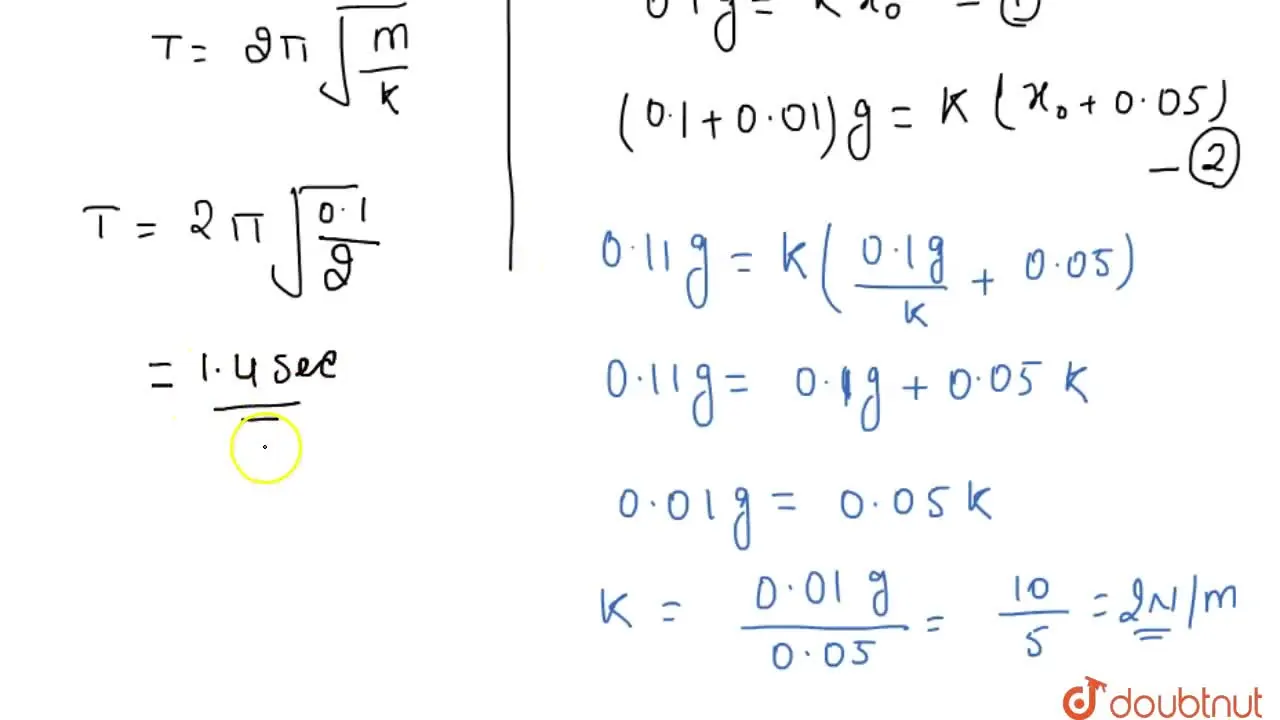A mass of 0.1 kg hangs at the end of a spring. When a mass of 0.01 kg more is added at the end of the spring, it stretches by 5 cm more. When the extra 0.01 kg mass is removed, determine the time period of vibration of the system.
Displacement produced, x = 5 cm, =5×10−2 m
Mass added, m = 0.01 kg
Since mg = kx
∴k=mgx=0.01×105×10−2=105=2 N/m
When mass m = 0.01 kg is removed, then mass hanging from the spring will become m = 0.01 kg
the time period of vibration is
T=2π√m.k=2π√0.12=1.404 s
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - PRACTICE PROBLEMS15videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - PRACTICE PROBLEMS 220videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST16videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
Similar Questions
A 0.2kg of mass hangs at the end of a spring. When 0.002kg more mass is added to the end of the spring, it stretches 7 cm more. If the 0.02 kg mass is removed what will be the period of vibration of the system?
View SolutionA 50g mass hangs at the end of a massless spring. When 20g more are added to the end of the spring, it stretches 7.0cm more. (a) Find the spring constant. (b) If the 20g are now removed, what will be the period of the motion ?
View SolutionWhen a mass m is hung on a spring, the spring stretched by 6 cm. If the loaded spring is pulled downward a little and released, then the period of vibration of the system will be
View SolutionA mass of 1 kg is hanged through a spring. The spring is stretched through 1cm. What is the energy stared in spring?
View SolutionThree mass 0.1 kg ,0.3 kg and 0.4 kg are suspended at end of a spring. When is 0.4 kg mass is removed , the system oscillates with a period 2 s . When the 0.3 kg mass is also removed , the system will oscillates with a period
View SolutionA mass of 1kg is hanged through a spring. The spring is stretched through 1cm. What is the energy stared in spring?
View SolutionA mass suspended on a vertical spring oscillates with a period of 0.5s. When the mass is allowed to hang at rest, the spring is stretched by
View SolutionA spring of force constant 1200Nm−1 is mounted on a horizontal table as shown in figure. A mass of 3.0kg is attached to the free end of the spring, pulled side ways to a distance of 2.0cm and released. Determing.
(a) the frequency of oscillation of the mass.
(b) the maximum acceleration of the mass.
(c) the maximum speed of the mass.
 View Solution
View SolutionA weightless spring of length 60 cm and force constant 100Nm−1 is kept straight and unstretched on a smooth horizontal table and its ends are rigidly fixed. A mass of 0.25 kg is attached at the middle of the spring and is slightly displaced along the length. The time period of the oscillation of the mass is
View SolutionWhen a force of 0.1 N is applied, the spring is stretched by 1.5 cm. The spring is cut into three parts and one part is stretched by 3 cm. Find the force required for doing so.
View Solution