If the right circular cone is separated into three solids of volumes V1,V2andV3 by two planes which are parallel to the base and trisects the altitude , then V1:V2:V3 is :
1:2:3
1:4:6
1:6:9
1:7:19
The correct Answer is:D
To solve the problem of finding the ratio of the volumes V1:V2:V3 of a right circular cone that is trisected by two planes parallel to the base, we can follow these steps:
1. Understand the Cone's Structure:
- Let the height of the cone be H.
- The cone is divided into three sections by two planes that trisect the height, creating three sections with heights h1=H3, h2=H3, and h3=H3.
2. Volume of the Entire Cone:
- The volume V of a cone is given by the formula:
V=13πr2H
- Let the radius of the base of the cone be r.
3. Volume of the First Section V1:
- The volume of the smaller cone formed at the top (with height h1=H3) can be calculated as:
V1=13πr21h1
- The radius r1 at height h1 can be found using similar triangles:
r1=rH⋅h1=rH⋅H3=r3
- Therefore, substituting r1 into the volume formula:
V1=13π(r3)2⋅H3=13π⋅r29⋅H3=πr2H81
4. Volume of the Second Section V2:
- The volume of the cone formed from the top to the height h2=2H3:
Vtotal_up_to_h2=13πr22h2
- The radius r2 at height h2:
r2=rH⋅h2=rH⋅2H3=2r3
- Thus, the volume up to h2:
Vtotal_up_to_h2=13π(2r3)2⋅2H3=13π⋅4r29⋅2H3=8πr2H243
- To find V2, we subtract V1:
V2=Vtotal_up_to_h2−V1=8πr2H243−πr2H81=8πr2H243−3πr2H243=5πr2H243
5. Volume of the Third Section V3:
- The volume of the entire cone is:
Vtotal=13πr2H
- To find V3, we subtract Vtotal_up_to_h2:
V3=Vtotal−Vtotal_up_to_h2=13πr2H−8πr2H243
- Converting 13 to a fraction with a denominator of 243:
V3=81πr2H243−8πr2H243=73πr2H243
6. Finding the Ratios:
- Now we have:
V1=πr2H81,V2=5πr2H243,V3=73πr2H243
- To find the ratio V1:V2:V3:
- Convert V1 to have a denominator of 243:
V1=3πr2H243
- So the ratio becomes:
V1:V2:V3=3:5:73
Final Answer:
The ratio V1:V2:V3 is 1:7:19.
- Let the height of the cone be H.
- The cone is divided into three sections by two planes that trisect the height, creating three sections with heights h1=H3, h2=H3, and h3=H3.
- The volume V of a cone is given by the formula:
V=13πr2H
- Let the radius of the base of the cone be r.
- The volume of the smaller cone formed at the top (with height h1=H3) can be calculated as:
V1=13πr21h1
- The radius r1 at height h1 can be found using similar triangles:
r1=rH⋅h1=rH⋅H3=r3
- Therefore, substituting r1 into the volume formula:
V1=13π(r3)2⋅H3=13π⋅r29⋅H3=πr2H81
- The volume of the cone formed from the top to the height h2=2H3:
Vtotal_up_to_h2=13πr22h2
- The radius r2 at height h2:
r2=rH⋅h2=rH⋅2H3=2r3
- Thus, the volume up to h2:
Vtotal_up_to_h2=13π(2r3)2⋅2H3=13π⋅4r29⋅2H3=8πr2H243
- To find V2, we subtract V1:
V2=Vtotal_up_to_h2−V1=8πr2H243−πr2H81=8πr2H243−3πr2H243=5πr2H243
- The volume of the entire cone is:
Vtotal=13πr2H
- To find V3, we subtract Vtotal_up_to_h2:
V3=Vtotal−Vtotal_up_to_h2=13πr2H−8πr2H243
- Converting 13 to a fraction with a denominator of 243:
V3=81πr2H243−8πr2H243=73πr2H243
- Now we have:
V1=πr2H81,V2=5πr2H243,V3=73πr2H243
- To find the ratio V1:V2:V3:
- Convert V1 to have a denominator of 243:
V1=3πr2H243
- So the ratio becomes:
V1:V2:V3=3:5:73
The ratio V1:V2:V3 is 1:7:19.
Topper's Solved these Questions
- MENSURATIONBook:ARIHANT SSCChapter:MENSURATIONExercise:EXERCISE (LEVEL 1)
Explore 55 Videos
- MENSURATIONBook:ARIHANT SSCChapter:MENSURATIONExercise:EXERCISE (LEVEL 2)
Explore 68 Videos
- MENSURATIONBook:ARIHANT SSCChapter:MENSURATIONExercise:INTRODUCTORY EXERCISE- 10.7
Explore 12 Videos
- LOGARITHM Book:ARIHANT SSCChapter:LOGARITHM Exercise:EXERCISE LEVEL 2
Explore 19 Videos
- MIXED GRAPHBook:ARIHANT SSCChapter:MIXED GRAPHExercise:Higher Skill Level Questions
Explore 15 Videos
Similar Questions
A fountain having the shape of a right circular cone is fitted into a cylindrical tank of volume V so that the base of the tank coincides with the base of the cone and the height of the tank is the same as that of the cone. The volume of water in the tank, when it is completely filled with water from the fountain, is V2 (b) V3 (c) V4 (d) 2V3
View SolutionA right circular cone is divided into three parts by trisecting its height by two planes drawn parallel to the base. Show that the volumes of the three portions starting from the top are in the ratio 1 : 7: 19.
View Solution
Knowledge Check
Let V1 be the volume of a given right circular cone with O as the centre of the base and A as its apex. Let V2 be the maximum volume of the right circular cone inscribed in the given cone whose apex is O and whose base is parallel to the base of the given cone. then the ratio V2/V1 is -
A325B49C427D827A car completes its journey in a straight line in three equal parts with speeds v1,v2andv3 respectively. The average speed v is given by :
Av1+v2+v33B3√v1v2v3C1v=1v1+1v2+1v3D3v=1v1+1v2+1v3A sample of gas expands from volume V1 to V2. The amount of work done by the gas is greatest when the expansion is
AAdiabaticBEqual in all casesCIsothermalDIsobaric
A regular triangular pyramid is cut by 2 planes which are parallel to its base. The planes trisects the altitude of the pyramid. Volume of top, middle and bottom part is V1,V_2andV_3respectively.WˆisthevalueofV_1: V_2: V_3?
एक सम त्रिभुजाकार पिरामिड को दो तल जो उसके आधार के समांतर है, द्वारा काया जाता है। तल पिरामिड की ऊँचाई को समत्रिभाजित करते है उसके ऊपरी, मध्य तथा निचले भाग का आयतन क्रमशः V_1, V2तथाV_3है।V_1: V_2: V_3 का मान क्या है?
View SolutionLet L1,L2,L3 be three distinct lines in a plane P and another line L, is equally inclined with these three lines. Statement-1: The line L is normal to the plane P. because Statement-2: if non zero vector →V is equally inclined to three non zero coplanar vector →V1,→V2,→V3 then the vector →V is normal to the plane of →V1,→V2 and →V3
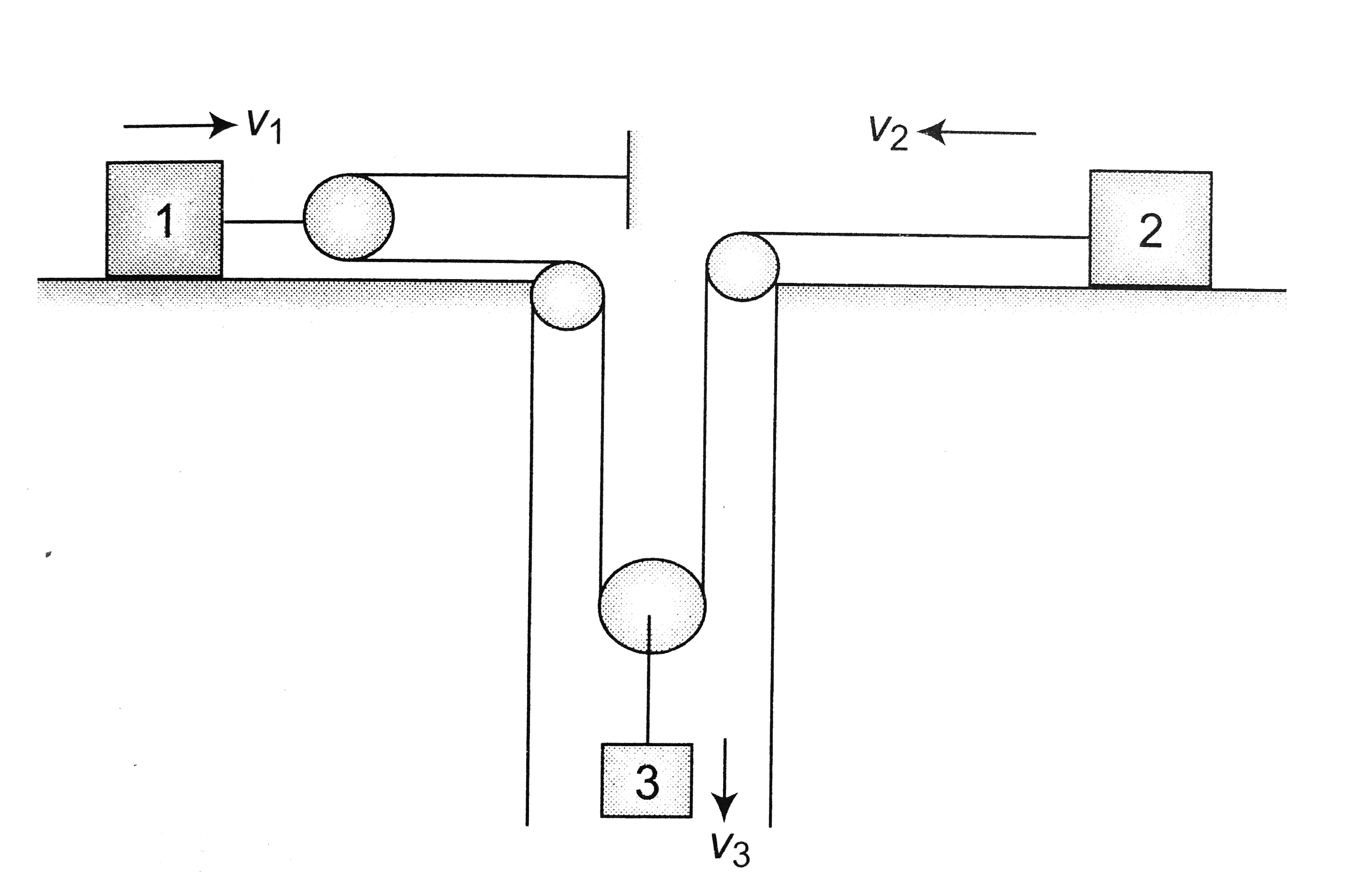
View SolutionThree block 1, 2 and 3 are arranged as shown in the figure. The velocities of the blockes v1 , v2 and v3 are shown in the figure. What is the relationship between v1 , v2 and v3 ?
View SolutionWhen a string is divided into three segments of
lengths l1,l2andl3 the fundamental frequencies of
these three segments are v1,v2andv3 respectively.
The original fundamental frequency (v) of the string is
View SolutionGiven the set of vectors v1=(1,2,3):v2=(1,0,1) and v3=(0,1,0)
View Solution