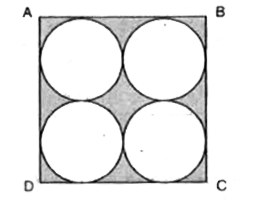
Find the area of the shaded region in fig., where ABCD is a square of side 14 cm and four circles are of same radii each.
128cm2
192cm2
148cm2
168cm2
The correct Answer is:A
To find the area of the shaded region in the square ABCD with side length 14 cm and four circles of equal radius, we can follow these steps:
Step 1: Calculate the area of the square
The area A of a square is given by the formula:
A=side2
Here, the side of the square is 14 cm. Thus,
A=142=196 cm2
Hint: Remember that the area of a square is calculated by squaring the length of one of its sides.
Step 2: Determine the radius of the circles
Since there are four circles inscribed in the square, we can infer that the circles are arranged such that their diameters fit within the side of the square. If we denote the radius of each circle as r, then the total diameter of the two circles along one side of the square is equal to the side length of the square:
2r+2r=14⟹4r=14⟹r=144=3.5 cm
Hint: The diameter of a circle is twice the radius, so when fitting multiple circles along a line, consider their combined diameters.
Step 3: Calculate the area of one circle
The area Ac of a circle is given by the formula:
Ac=πr2
Using r=3.5 cm, we have:
Ac=π(3.5)2=π×12.25≈38.48 cm2
Hint: Use π≈227 for easier calculations if needed.
Step 4: Calculate the total area of the four circles
Since there are four circles, the total area Atotal_circles is:
Atotal_circles=4×Ac=4×38.48≈153.92 cm2
Hint: To find the total area of multiple identical shapes, multiply the area of one shape by the number of shapes.
Step 5: Calculate the area of the shaded region
The area of the shaded region Ashaded is the area of the square minus the total area of the circles:
Ashaded=Asquare−Atotal_circles=196−153.92≈42.08 cm2
Hint: The shaded area is found by subtracting the area of the circles from the area of the square.
Final Answer
The area of the shaded region is approximately:
42 cm2
The area A of a square is given by the formula:
A=side2
Here, the side of the square is 14 cm. Thus,
A=142=196 cm2
Since there are four circles inscribed in the square, we can infer that the circles are arranged such that their diameters fit within the side of the square. If we denote the radius of each circle as r, then the total diameter of the two circles along one side of the square is equal to the side length of the square:
2r+2r=14⟹4r=14⟹r=144=3.5 cm
The area Ac of a circle is given by the formula:
Ac=πr2
Using r=3.5 cm, we have:
Ac=π(3.5)2=π×12.25≈38.48 cm2
Since there are four circles, the total area Atotal_circles is:
Atotal_circles=4×Ac=4×38.48≈153.92 cm2
The area of the shaded region Ashaded is the area of the square minus the total area of the circles:
Ashaded=Asquare−Atotal_circles=196−153.92≈42.08 cm2
The area of the shaded region is approximately:
42 cm2
Topper's Solved these Questions
- MENSURATIONBook:ARIHANT SSCChapter:MENSURATIONExercise:EXERCISE (LEVEL 1)
Explore 55 Videos
- MENSURATIONBook:ARIHANT SSCChapter:MENSURATIONExercise:EXERCISE (LEVEL 2)
Explore 68 Videos
- MENSURATIONBook:ARIHANT SSCChapter:MENSURATIONExercise:INTRODUCTORY EXERCISE- 10.7
Explore 12 Videos
- LOGARITHM Book:ARIHANT SSCChapter:LOGARITHM Exercise:EXERCISE LEVEL 2
Explore 19 Videos
- MIXED GRAPHBook:ARIHANT SSCChapter:MIXED GRAPHExercise:Higher Skill Level Questions
Explore 15 Videos
Similar Questions
Knowledge Check
Find the area of the shaded region in fig., where ABCD is a square of side 14 cm and four circles are of same radii each.
A128cm2B192cm2C148cm2D168cm2
In the given figure, find the area of the shaded region, where ABCD is a square of side 14 cm and al circles are of the same diameter.
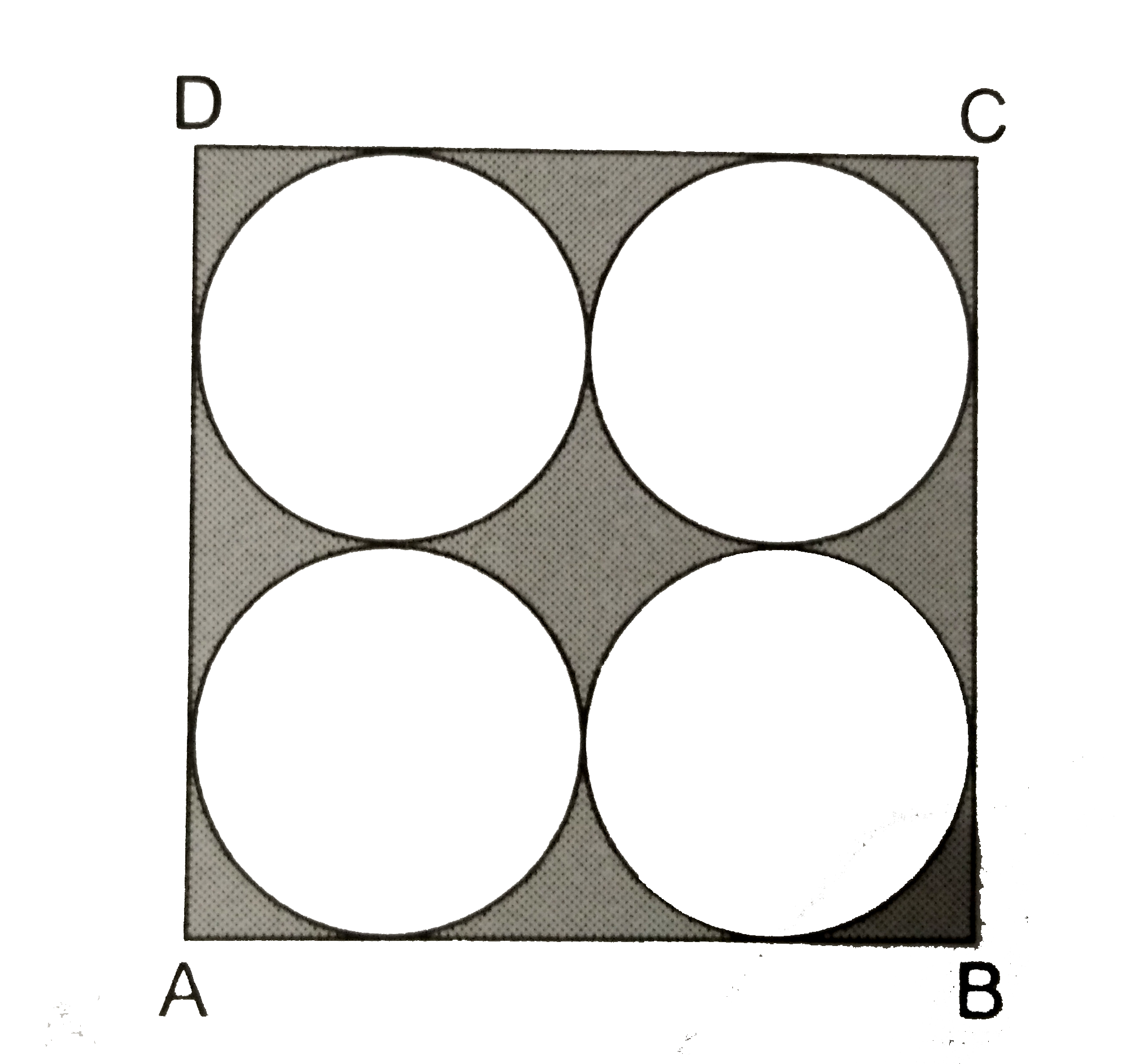
View SolutionFind the area of the shaded region as shown in figure, where ABCD is square of side 14 cm.
View SolutionFind the area of the shaded design in Fig. 12.17, where ABCD is a square of side 10 cm and semicircles are drawn with each side of the square asdiameter.
View SolutionFind the area of the shaded region in figure, if ABCD is a square of side 7 cm. and APD and BPC are semi-circles
View SolutionFind the area of the shaded region in Fig. 12.21, if ABCD is a square of side 14 cm and APD and BPC are semicircles.
View SolutionFind the perimeter of the shaded region if ABCD is a square of side 14 cm and APB and CPD are semicircles
View SolutionFind the area of the shaded region in Fig. 15.48, where ABCD is a square of side 10 cm. (Use π=3.14) (FIGURE)
View Solution