Consider a wire carrying a steady current, I placed in a uniform magnetic field $\to B$ perpendicular to its length. Consider the charges inside the wire. It is known that magnetic forces do not work. This implies that

A

Maximum possible magnetic field is $B_{0} + \frac{μ_{0} I}{2 π R}$

B

Minimum magnetic field at some point is zero if $R < \frac{μ_{0} I}{2 π B_{0}}$

C

Minimum magnetic field at some point is $B_{0} - \frac{μ_{0} I}{2 π R}, if R > \frac{μ_{0} I}{2 π B_{0}}$

D

Magnetic field at a point on its axis is $B_{0}$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:a, b, c, d

Magnetic field lines due to the current in cylinder are circles perpendicular to the length of cylinder and hence at some points, its magnetic field will become parallel or anti-parallel to the applied magnetic field.
Maximum possible magnetic field is obtained for points where the field due to cylinder is maximum (points on its surface) and parallel to the applied magnetic field. Hence $B_{0} + \frac{μ_{0} I}{2 π R}$ will be the maximum possible magnetic field at some suitable point on its surface.
Option (a) is correct.
We know that at some point on its surface, the applied magnetic field is anti-parallel to the field created by current. But in case of the minimum possible magnetic field, there is a little twist.
Let us suppose that the applied magnetic field is less than the maximum field due to current, then there will be a point on either side of the surface where the field due to current is equal to the applied field and thus the resultant becomes zero for these points.
$B_{0} < \frac{μ_{0} I}{2 π R} \Rightarrow R < \frac{μ_{0} I}{2 π B_{0}}$
Hence option (b) is correct.
If applied field is more than the maximum possible field due to current, then the minimum magnitude of magnetic field will be certainly on the surface and its magnitude will be $B_{0} - \frac{μ_{0} I}{2 π R}$ . This is the case
when $B_{0} > \frac{μ_{0} I}{2 π R} \Rightarrow R > \frac{μ_{0} I}{2 π B_{0}}$
Option (c ) is correct.
Option (d) is also correct because magnetic field due to the current is zero at a point on the axis of the cylinder.

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A wire carrying a current $i$ is placed in a uniform magnetic field in the form of the curve $y = a sin (\frac{π x}{L}) 0 \leq x \leq 2 L$ . The force acting on the wire is

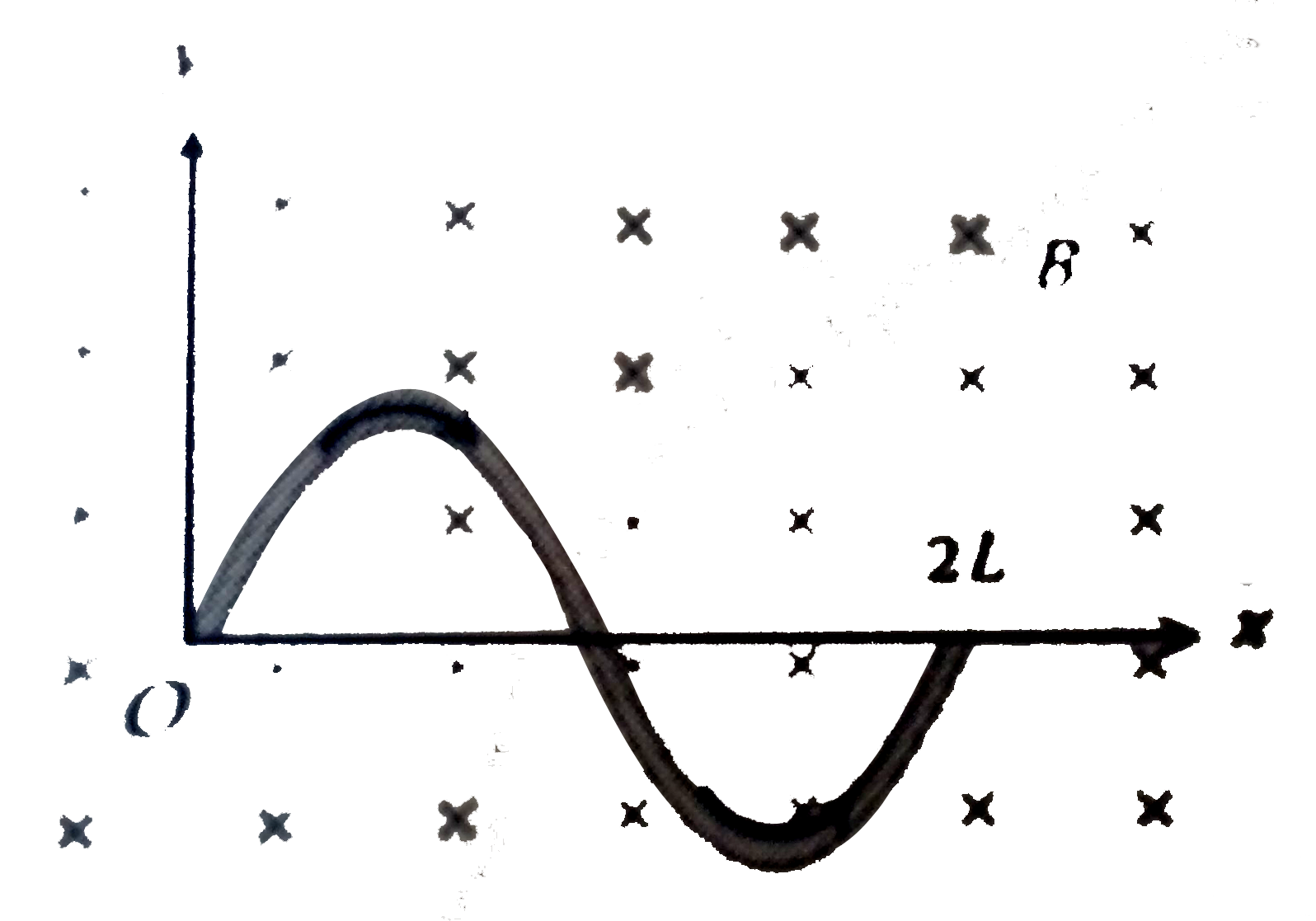A $\frac{i B L}{π}$
B $i B L π$
C $2 i B L$
Dzero
Question 2 - Select One
An 8 cm long wire carrying a current of 10 A is placed inside a solenoid perpendicular to its axis. If the magnetic field inside the solenoid is 0.3 T, then magnetic force on the wire is
A0.14N
B0.24N
C0.34N
D0.44N
Question 3 - Select One
A current (I) carrying circular wire of radius R is placed in a magnetic field B perpendicular to its plane. The tension T along the circumference of the wire is

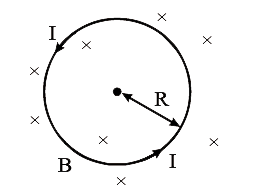A $B I R$
B $2 π B I R$
C $π B I R$
D $2 B I R$

MODERN PUBLICATION-MOVING CHARGES AND MAGNETISM-COMPETITION FILE (C. MULTIPLE CHOICE QUESTIONS)

Home
Ask Doubt
Profile