Consider a wire carrying a steady current, I placed in a uniform magnetic field $\vec{B}$ perpendicular to its length. Consider the charges inside the wire. It is known that magnetic forces do not work. This implies that

A

the motion of charges inside the conductor is unaffected by B since they do not absorb energy.

B

some charges inside the wire move to the surface as a result of B.

C

if the wire moves under the influence of B, no work is done by the force.

D

if the wire moves under the influence of B, no work is done by the magnetic force on the ions that is assumed fixed within the wire.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:b, d

Let us consider a wire connected to a battery between two rails and a uniform external magnetic field $\vec{B}$ as shown in the figure.

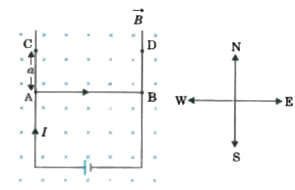
Due to current flowing through the wire AB on application of external electric field (battery), the free charges inside the wire will start drifting towards the positive terminal of the battery with an average velocity v.
The force acting on the charge due to external magnetic field B will be:
$F_{q} = q \vec{v} \times \vec{B}$
The force acting on the free charges will be given by the left-hand rule and is from south to north (if the charge is positive) and from north to south (if the charge is negative).
Now, due to this force experienced by the moving charges, there will be shifting of the charges on the surface of wire and it will also contribute to the net force acting on the wire.
When the wire moves under the influence of this magnetic force, the ions fixed inside the wire are dragged along with it. So, the velocity of ions is now towards the north direction and due to magnetic field, they will experience a force along the length of wire (towards west).
Since, the displacement of ions and force acting on them are mutually perpendicular, the network done on the ions by the magnetic force will be zero.
Also, force acting on wire of length l and carrying a current I in an external field is given by:
$F_{1} = I (\vec{l} \times \vec{B}) = I l B \sin θ$
Here , $θ$ = angle between length of the wire and external magnetic field $= 90^{\circ}$
$∴ F_{1} = I l B$
Since the displacement of wire is along the direction of force acting on it, work will be done on the wire if it moves under the influence of $\vec{B}$ .
Thus, options (b) and (d) are correct.

Updated on:21/07/2023

|

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

A wire carrying a current $i$ is placed in a uniform magnetic field in the form of the curve $y = a sin (\frac{π x}{L}) 0 \leq x \leq 2 L$ . The force acting on the wire is

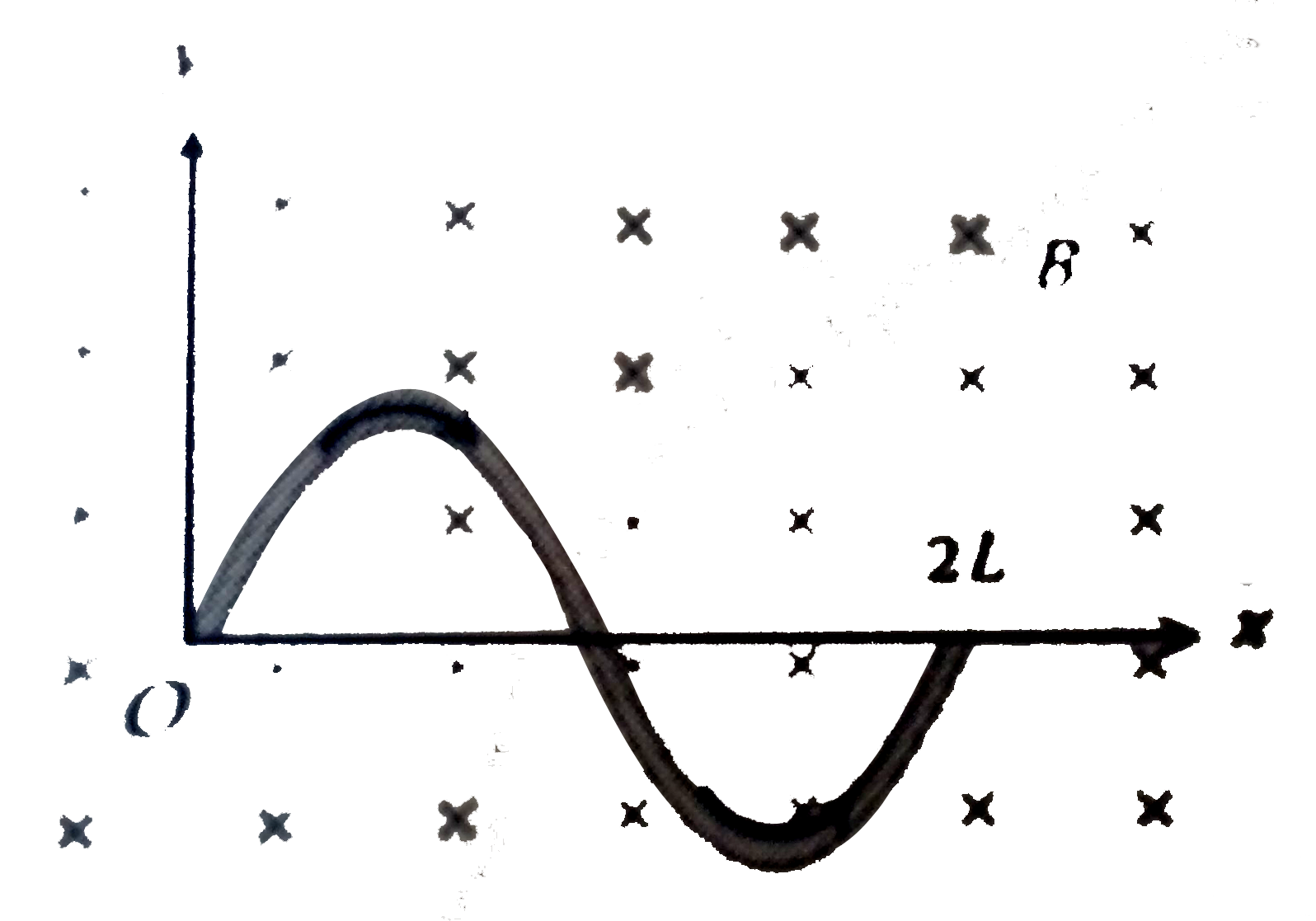A $\frac{i B L}{π}$
B $i B L π$
C $2 i B L$
Dzero
An 8 cm long wire carrying a current of 10 A is placed inside a solenoid perpendicular to its axis. If the magnetic field inside the solenoid is 0.3 T, then magnetic force on the wire is
A0.14N
B0.24N
C0.34N
D0.44N
A current (I) carrying circular wire of radius R is placed in a magnetic field B perpendicular to its plane. The tension T along the circumference of the wire is

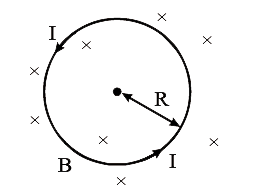A $B I R$
B $2 π B I R$
C $π B I R$
D $2 B I R$

MODERN PUBLICATION-MOVING CHARGES AND MAGNETISM-NCERT (Exemplar Problems ) Objective Questions (MCQ Type - II)

Home
Ask Doubt
Profile