Question

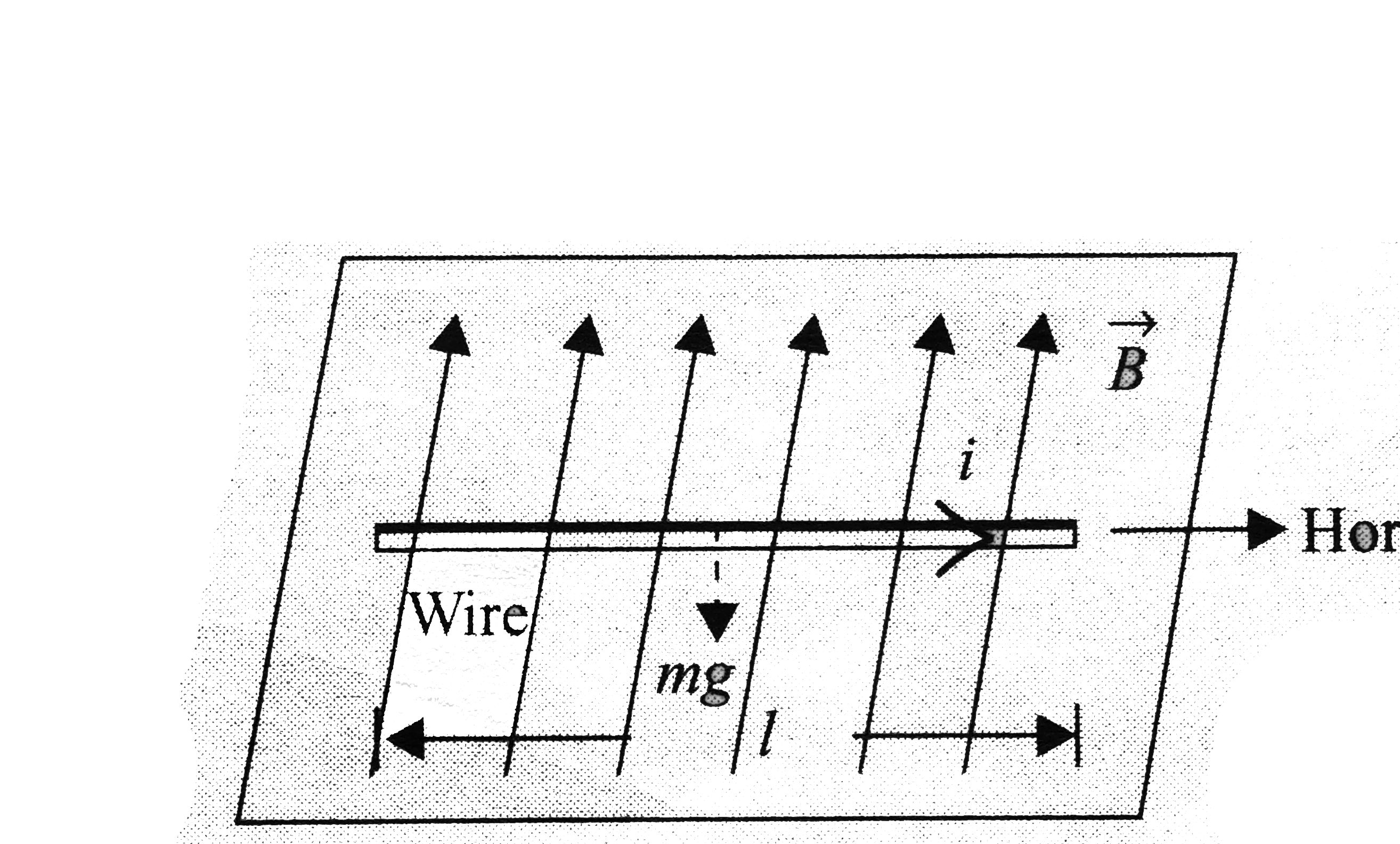
A straight wire carrying current of 1 A is suspended in a magnetic field. If the length and mass of the wire are 50 cm and 500 g respectively, what should be the magnitude of the magnetic field, so that it stays in equilibrium? Take $g=10m/s^2$.

Explanation

To find the magnitude of the magnetic field required for the straight wire carrying a current of 1 A to stay in equilibrium, we can follow these steps:

1. Identify the Forces Acting on the Wire:
The wire experiences two forces:
- The downward gravitational force (weight) due to its mass.
- The upward magnetic force due to the magnetic field.

2. Calculate the Weight of the Wire:
The weight (W) of the wire can be calculated using the formula:
\( W = mg \)
where:
- \( m = 500 \, \text{g} = 0.5 \, \text{kg} \) (mass of the wire)
- \( g = 10 \, \text{m/s}^2 \) (acceleration due to gravity)

Substituting the values:
\( W = 0.5 \, \text{kg} \times 10 \, \text{m/s}^2 = 5 \, \text{N} \)

3. Set Up the Equation for Equilibrium:
For the wire to be in equilibrium, the upward magnetic force must equal the downward weight of the wire. The magnetic force (F) on the wire can be calculated using the formula:
\( F = I \cdot L \cdot B \)
where:
- \( I = 1 \, \text{A} \) (current through the wire)
- \( L = 50 \, \text{cm} = 0.5 \, \text{m} \) (length of the wire)
- \( B \) is the magnetic field strength we need to find.

Setting the forces equal for equilibrium:
\( I \cdot L \cdot B = mg \)

4. Rearranging the Equation to Solve for B:
\( B = \frac{mg}{IL} \)

5. Substituting the Known Values:
Now substitute \( m = 0.5 \, \text{kg} \), \( g = 10 \, \text{m/s}^2 \), \( I = 1 \, \text{A} \), and \( L = 0.5 \, \text{m} \):
\( B = \frac{(0.5 \, \text{kg})(10 \, \text{m/s}^2)}{(1 \, \text{A})(0.5 \, \text{m})} \)

6. Calculating B:
\( B = \frac{5 \, \text{N}}{0.5 \, \text{A} \cdot \text{m}} = \frac{5}{0.5} = 10 \, \text{T} \)

Final Answer:
The magnitude of the magnetic field required for the wire to stay in equilibrium is 10 T (Tesla).

---