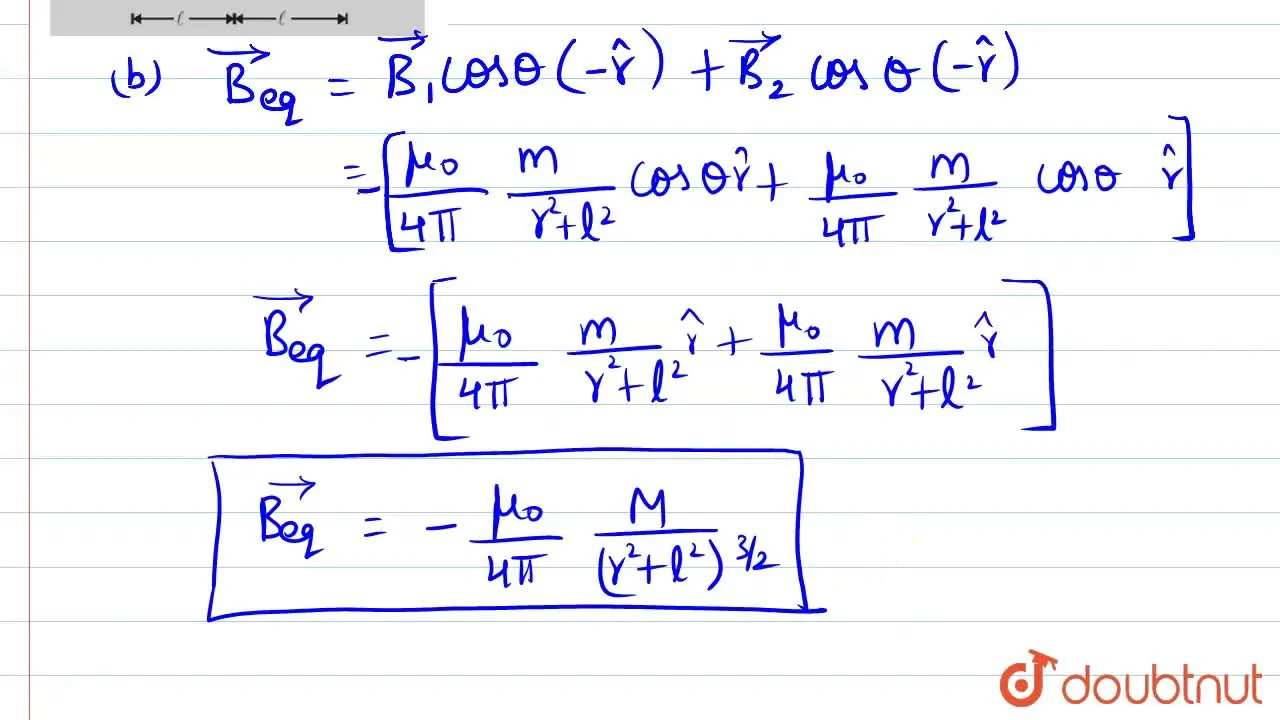
What is the magnetic field along the axis and equatorial line of a bar magnet ? Solution in Tamil

Video Solution

Text Solution

Verified by Experts

Magnetic field at a point along the axial line of the magnetic dipole (bar magnet) :
Consider a bar magnet NS. Let N be the North Pole and S be the south pole of the bar magnet, each of pole strength $q_{m}$ and separated by a distance of 2l. The magnetic field at a point C (lies along the axis of the magnet at a distance from the geometrical center O of the bar magnet can be compute by keeping unit north pole ( $q_{M C}$ = 1 A m ) at C. the force e $\times$ perienced by the unit north pole at C due to pole strength can be compute using Coulomb.s law of magnetism as follows :
The force of repulsion between north pole of the bar magnet and unit north pole at point C (in free space ) is
$- \to F_{N} = \frac{μ_{0}}{4 π} \frac{q_{m}}{{(r - l)}^{2}} ˆ l$
where r + l is the distance between north pole of the bar magnet and unit north pole at C
The force of attraction between south Pole of the bar magnet and unit North Pole at point C (in free space ) is
$- \to F_{S} = \frac{μ_{0}}{4 π} \frac{q_{m}}{{(r - l)}^{2}} ˆ l$
where r + l is the distance between south pole of the bar magnet and unit north pole at C.
Magnetic field at a point along the axial line due to magnetic dipole
From equation (1) and (2) , then net force at point C is $\to F = - \to F_{N} + - \to F_{S}$ . From definition, this net force is the magnetic field due to magnetic dipole at a point C $(\to F = \to B)$
$\to B = \frac{μ_{0}}{4 π {(r - l)}^{2}} ˆ i + (\frac{μ_{0}}{4 π} \frac{q_{m}}{{(4 + l)}^{2}} ˆ i) = \frac{μ_{0} q_{m}}{4 π} (\frac{1}{{(r - l)}^{2}} - \frac{1}{{(r + l)}^{2}}) ˆ i$
$\to B = \frac{μ_{0} 2 r}{4 π} (\frac{q_{m} . (2 l)}{{(r^{2} - l^{2})}^{2}}) ˆ i$
Since, magnitude of magnetic dipole moment is $∣ ∣ ∣ - \to P_{m} ∣ ∣ ∣ = p_{m} = q_{m} .2 l$ then magnetic field point C equation (3) can be written as
$- -- \to B_{axial} = \frac{μ_{0}}{4 π} (\frac{2 r p_{m}}{{(r^{2} - l^{2})}^{2}}) ˆ i$
if the distance between two poles in a bar magnet are small (looks like short magnet ) compared to the distnace between geometical centre O of bar magnet and the locatio of point C i.e.,
$r > >$ l then, ${(r^{2} - l^{2})}^{2} \approx r^{4}$
Therefore, using equation (5) in equation (4) , we get
$- -- \to B_{axial} = \frac{μ_{0}}{4 π} (\frac{2 r p_{m}}{(r^{3})}) ˆ i = \frac{μ_{0}}{4 π} \frac{2}{r^{3}} - \to p_{m}$
Where $- \to P_{m} = p_{m} ˆ i$ .

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

FULL MARKS-MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT -TEXT EVALUATION SOLVED - LONG ANSWER QUESTIONS

Home
Ask Doubt
Profile