Question

Derive an expression for electric flux of rectangular area placed in uniform electric field.

Explanation

(i) Electric flux for uniform electric field: Consider a uniform electric field in a region of space. Let uschoose an area A normal to the electric field lines as shown in figure (a).
The electric flux for this case is
`Phi_(E) = "EA "`...(1)
Suppose the same area A is kept parallel to the uniform electric field, then no electric field lines pierce through the area A, as shown in figure (b). The electric flux for this case is zero.
`Phi_(E) = 0 " "`....(2)
If the area is inclined at an angle `theta` with the field, then the component of the electric field perpendicular to the area alone contributes to the electric flux. The electric field component parallel to the surface area will not contribute to the electric flux. This is shown in figure (c ). For this case, the electric flux
`Phi E = (E cos theta)"A "`....(3)
Further, `theta` is also the angle between the electric field and the direction normal to the area. hence in general, for uniform electric field. The electric flux is defined as
`Phi_(E) = vec(E).vec(A)" = EA cos" theta" "`....(4)

Here, note that `vec(A)` is the area vector `vec(A) = A hat(n)`. Its magnitude is simply the area A and the direction is along the unit vector `hat(n)` perpendicular to the area. Using this definition for flux. `Phi_(E) = vec(E).vec(A)`.equations (2) and (3) can be obtained as special cases.
In figure(a), `theta = 0^(@) " so "Phi_(E) = vec(E).vec(A) = EA`
In figure (b), `theta = 90^(@) "so" Phi_(1) = vec(E).vec(A) =0`
(ii) Electric flux in a non uniform electric field and an arbitrarily shaped area: Suppose the electric field is not uniform and the area A is not flat, then the entire area is divided into n small area segments `Delta vec(A_(1)), Delta vec(A_(2)), Delta vec(A_(3)), ........ Delta vec(A_(n))`, such that each area element is almost flat and the electric field over each area element is considered to be uniform .
The electric flux for the entire area A is approximately written as
`Phi_(E) = vec(E_(1)) . Delta vec(A_(1)) + vec(E_(2)).Delta vec(A_(2)) + vec(E_(3)).Delta vec(A_(3)) + ....... + vec(E_(n)). Delta vec(A_(n)) = sum_(i = l)^(n) vec(E_(i)). Delta vec(A_(i))` .... (5)
By taking the limit `Delta vec(A_(1)) rarr theta` (for all i) the summation in equation (5) becomes integration . the total electric flux for the entire area is given by
`Phi_(E) = int vec(E).d vec(A) " "`....(6)
From Equation (6), It is clear that the electric flux for a given surface depends on both the electric field pattern on the surface area and orientation of the surface with respect to the electric field.
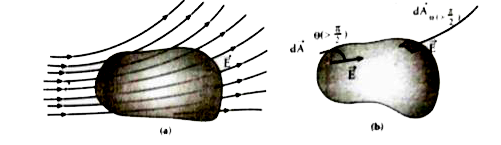
(iii) Electric flux for closed surfaces : In the previous section, the electric flux for any arbitrary curved surface is discussed Suppose a closed surface is present in the region of the non-uniform electric field as shown in figure (a).
The total electric flux over this closed surface is written as
`Phi_(E) - oint E.d vec(A)" "`....(7)
Note the difference between equations (6) and (7) the integration equation (7) is a closed surface integration and for each areal element, the outward normal is the direction of d`vec(A)` as shown in the figure (b).
The total electric flux over a closed surface can be negative,
Positive or zero. In the figure (b), it is shown that in one area element , the angle between d`vec(A) and vec(E)` is less than `90^(@)`, then the electric flux is positive and in another areal element, the angle between d `vec(A) and vec(E)` is greater than `90^(@)` , then the electric flux is negative.
In general, the electric flux is negative if the electric field lines enter the closed surface and positive if the electric field lines leave the closed surface.