Calculate the viscous force on a ball of radius Imm moving through a liquid of viscosity at a speed of .Solution in Tamil
According to Stoke.s law
Topper's Solved these Questions
- SAMPLE PAPER -6 (SOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -6 (SOLVED)EXERCISE - PART-III
9 Videos
- SAMPLE PAPER -6 (SOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -6 (SOLVED)EXERCISE - PART-IV
12 Videos
- SAMPLE PAPER -6 (SOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -6 (SOLVED)EXERCISE - PART-IV
12 Videos
- SAMPLE PAPER -4BOOK - FULL MARKSCHAPTER - SAMPLE PAPER -4EXERCISE - PART-IV
10 Videos
- SAMPLE PAPER 11 (UNSOLVED)BOOK - FULL MARKSCHAPTER - SAMPLE PAPER 11 (UNSOLVED)EXERCISE - Part IV
10 Videos
Similar Questions
Centripetal acceleration is given by
View SolutionObtain an expression for the time period T of a simple pendulum. [The time period T depend upon (i) mass l of the bob (ii) length m of the pendulum and (iii) acceleration due to gravity g at the place where pendulum is suspended.
Assume the constant k=2π]
View SolutionObtain an expression for the time period T of a simple pendulum. [The time period T depend upon (i) mass l of the bob (ii) length m of the pendulum and (iii) acceleration due to gravity g at the place where pendulum is suspended.
Assume the constant k=2π]
View SolutionAt the highest point of oblique projection, which of the following is correct?
View SolutionObtain in expression for the time period T of a simple pendulun. The time period depend upon (i) mass 'm' of the bob (ii) length 'l' of the pendulum and (iii) acceieration due to gravity g at the place where the pendulum is suspended. (Constant k = 2π ) i.e.
View Solution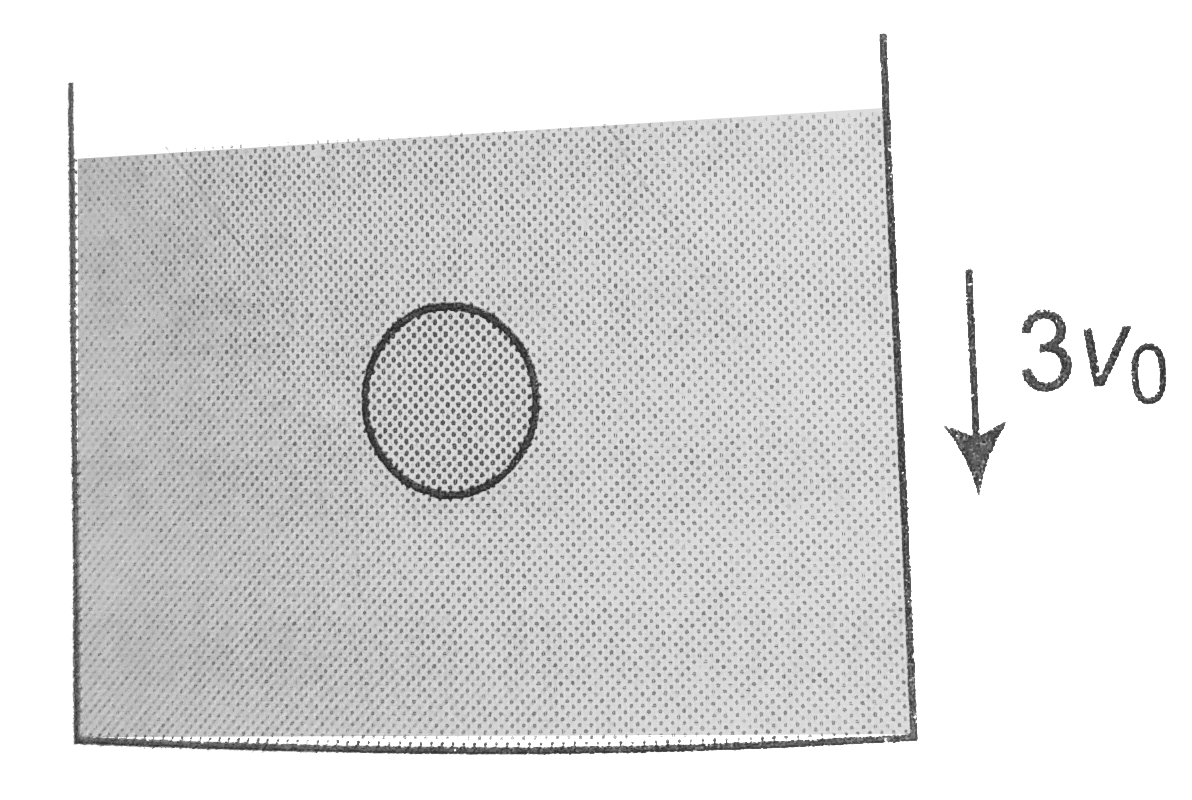
A container filled with viscous liquid is moving vertically downwards with constant speed 3v0. At the instant shown, a sphere of radius r is moving vertically downwards (in liquid) has speed v0. The coefficient of viscosity is η. There is no relative motion between the liquid and the container. Then at the shoen instant, The magnitude of viscous force acting on sphere is
View SolutionWhat is the viscous force on the drop of liquid of radius 0.2 mm moving with a constant velocity 4cms−1 throught a medium of viscosity 1.8×10−5Nm−2s.
View SolutionThe viscous force on a spherical body, when it moves through a viscous liquid, depends on the radius of the body, the coefficient of viscosity of the liquid and the velocity of the body.Find an expression for the viscous force.
View SolutionWhen a liquid flows in a tube, there is relative motion between adjacent layers of the liquid. This force is called the viscous force which tends to oppose the relative motion between the layers of the liquid. Newton was the first person to study the factors that govern the viscous force in a liquid. According to Newton’s law of viscous flow, the magnitude of the viscous force on a certain layer of a liquid is given by
F=−ηAdvdx
where A is the area of the layer dvdx is the velocity gradient at the layer and η is the coefficient of viscosity of the liquid.
A river is 5 m deep. The velocity of water on its surface is 2ms−1 If the coefficient of viscosity of water is 10−3Nsm−2 , the viscous force per unit area is :
View SolutionR त्रिज्या की गोल गेंद η श्यानता के किसी श्यान तरल में वेग v से गिर रही है । गोल गेंद पर कार्यरत मंदक श्यान बल है -
View SolutionA plate of area 10m2 is made to move horizontally with a speed of 0.5ms−1 by applying a force over the surface a liquid. If the depth of the liquid is 2.5m, coefficient of viscosity of liquid is 10−3Nsm−2, then calculate the horizontal force needed to move the plate.
View SolutionThe speed of a ball of radius 2 cm in a viscous liquid medium is 20 cm .S−1 . The speed of a ball of radius 1 cm in the same liquid will be
View SolutionThe speed of a ball of radius 2 cm in viscous liquid medium is 20 cm .s^-1 . The speed of a ball of radius 1 cm in the same liquid will be
View SolutionR ব্যাসার্ধের গোলাকার বল (η) সান্দ্রতাঙ্কবিশিষ্ট একটি সান্দ্র মাধ্যমের মধ্য দিয়ে v বেগে পতনশীল। গোলাকার বলের ওপর সান্দ্রতাজনিত বল F হলে
View SolutionCalculate the viscous force on a liquid drop of radius 0.3 mm moving with a velocity of 5 cms^(-1) through a medium of viscosity 2 xx 10^(-5) Nm^(-2)s.
View Solution
